Atomic-Sized Particles Have Unusual Properties
Bohr’s model predicted experimental data for the hydrogen-atom emission spectra and was widely accepted, but it also raised questions. Why did electrons have energies defined by a single quantum number n = 1, 2, 3, and so on, but never in between? How could the model work so well for a hydrogen atom and one-electron ions, but not accurately predict the emission spectrum for helium or any atom or ion with two or more electrons? To answer such questions, we need to explore the unusual properties of matter at the atomic scale.
It is natural to try to interpret the behavior of electrons in atoms using analogies to the behavior of things we can see and experience. By the turn of the 20th century, scientists had identified two different kinds of behavior that might serve as analogies: waves and particles. Waves, such as water waves, can bend around objects and exhibit interference. A particle, such as a pea or tennis ball, moves in a straight line unless its path is changed by a force (such as friction, gravity, or hitting something). It turns out that properties of atomic-scale matter are best explained using a combination of these analogies.
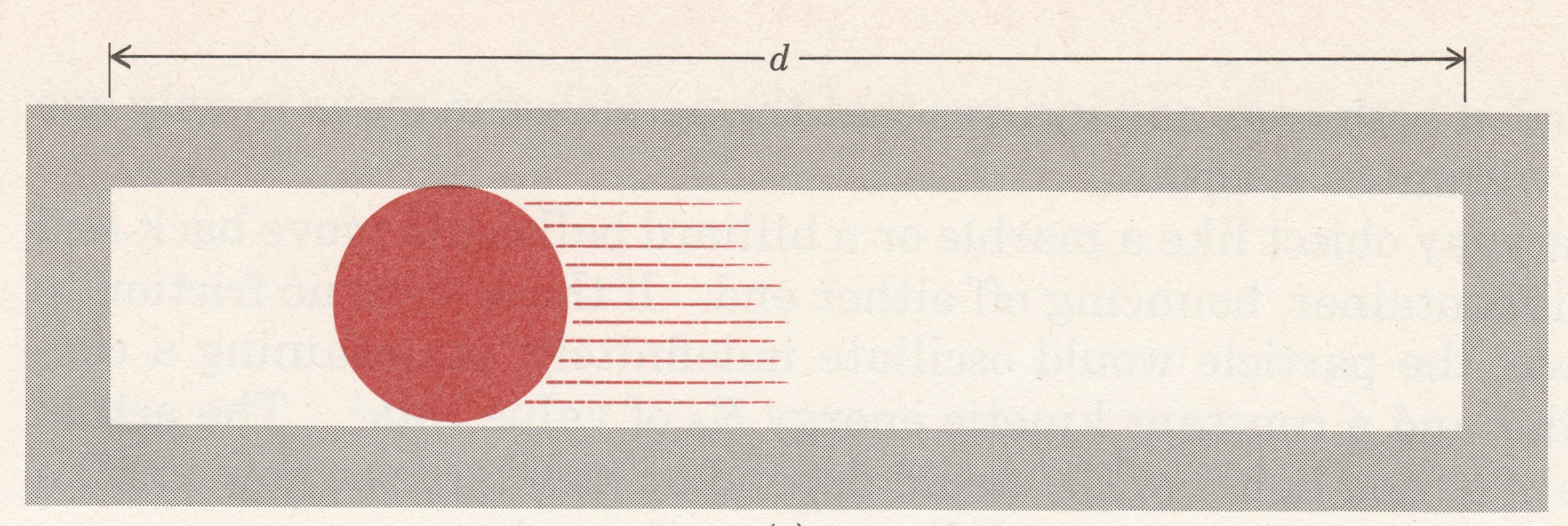
Let’s think about an electron as if it were a particle and choose a very simple, but artificial, situation. Figure 1 shows an electron that is constrained to move in a single dimension, say the x direction. There are walls to the left and right of the electron that prevent it from moving beyond them; the distance between the walls is d (the electron is in a box of length d). Given an initial push, the electron will move back and forth forever (assuming no friction). The electron’s kinetic energy would be given by the equation Ek = ½mv2, where m is the mass and v the velocity.
Consider these questions regarding the electron moving in a one dimensional box:
But to explain the hydrogen-atom line spectrum, Bohr had to assume that an electron in an atom can only have certain energies. This assumption seemed very unsatisfactory based on the particle analogy—as we just saw, an electron should be able to have any energy value.
Let’s explore a wave analogy instead. In 1924, Louis de Broglie proposed that a wave of wavelength λ is associated with every particle. The larger the mass of the particle and the faster it is moving, the smaller this wavelength becomes. The relationship is given by the formula:
where p is the momentum of the electron, the product of its mass and velocity (p = m × v), and h is Planck’s constant (h = 6.626 × 10–34 J·s).
Optional Information: Wavelike behavior of electrons was confirmed in 1927; read about it here. View this video for another description of the relevant experiments.
Suppose a wave, such as a vibrating guitar string, occupies the box. A guitar string is constrained by being fixed at each end so we assume the ends of the string are tied to the walls at the ends of the box.
In your course notebook, make a drawing of what a vibrating string of length d, with its ends in fixed positions, would look like. If you are studying with someone else, each make a drawing individually and then compare.
If an electron behaves like a guitar string, then only certain wavelengths will fit within the one-dimensional box with length d (see the figure in Activity 2). The length of the box can thus correspond to: a single half-wavelength, two half-wavelengths, three half-wavelengths, etc., but not to any wave where either end of the string is moving. In other words, the length d must correspond to an integer number of half wavelengths:
where n = 1, 2, 3, 4,… Solving the equation for λ, we obtain:
Combining this with de Broglie’s equation, ![]() , we have:
, we have:
which rearranges to give:
We can now calculate the kinetic energy of our wave-particle. It is given by the formula:
Because n is a positive whole number, this equation says that the kinetic energy of the electron can have only certain values and not others.
This result shows that if we think of an electron as a wave in a 100 pm box, its energy is automatically restricted to certain specific values: the electron can have an energy of 6.0 attojoules (aJ) or 24.0 aJ, but not an intermediate energy such as 7.3 aJ or 11.6 aJ. We describe this situation by saying that the energy of the electron is quantized. Because the energy of 6.0 aJ is the lowest possible energy, the electron is most stable at this energy, where the box contains half a wavelength. This is the ground state. If the energy has a higher value, such as 24 aJ or 54 aJ, the electron is in an excited state. Notice that for the excited states the electron waves have one or more nodes. A node is a point where the wave has zero amplitude; that is, where the wave is not moving up or down at all. The greater the number of nodes, the higher the energy, a generalization that is true for all kinds of waves.
This new way of approaching the behavior of electrons (and other atomic-sized particles) became known as wave mechanics or quantum mechanics. Using both wave and particle analogies to describe atomic-scale matter is referred to as wave-particle duality. Wave-particle duality implies that we can no longer state that the electron is located at a specific position within the box or is moving in one direction or the other: the electron seems to be all over the box at once!
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂 (Note that we cannot answer questions via the google form. If you have a question, please post it on Piazza.)

