D41.3 Relationships among ΔG°, K°, and E°
The thermodynamics of a redox reaction can be described in terms of ΔrG° and K°, and these can be further related to E°cell because they all describe whether the redox reaction is reactant-favored or product-favored at equilibrium.
In a voltaic cell, the difference in Gibbs free energy between products and reactants allows the cell to do electrical work. We represent electrical work done by the cell as welec.
The charge of 1 mole of electrons is known as the Faraday’s constant (F):
Hence, the total quantity of charge transferred per mole of a redox reaction is:
In this equation, n is the number of electrons transferred in the balanced redox reaction, which can be obtained from the balanced half-reactions that are added to produce the overall redox reaction. For example, in the reaction:
6 e− are transferred because each half-reaction, after multiplying by a factor to balance electrons, involves 6 e−:
| Oxidation: | 3 × [Zn(s) | ⟶ | Zn2+(aq) + 2 e−] |
| Reduction: | 2 × [Au3+(aq) + 3 e− | ⟶ | Au(s)] |
| Overall: | 2 Au3+(aq) + 3 Zn(s) | ⟶ | 3 Zn2+(aq) + 2 Au(s) |
When this redox reaction happens once, 6 e− are transferred. When a mole of this reaction takes place, 6 moles of e− are transferred; that is, 6 mol × 96485 C/mol = 578910 C are transferred. Hence, we have:
| welec | = | charge transferred × potential difference |
| welec | = | (nF) × (Ecell) |
If we operate a voltaic cell such that the maximum possible electrical work, welec, is done and the only work done is electrical work, then:
The negative sign makes sense because a positive Ecell indicates a spontaneous redox reaction while for ΔrG a negative value indicates a spontaneous reaction.
If all the reactants and products are in their standard states, then the equation becomes:
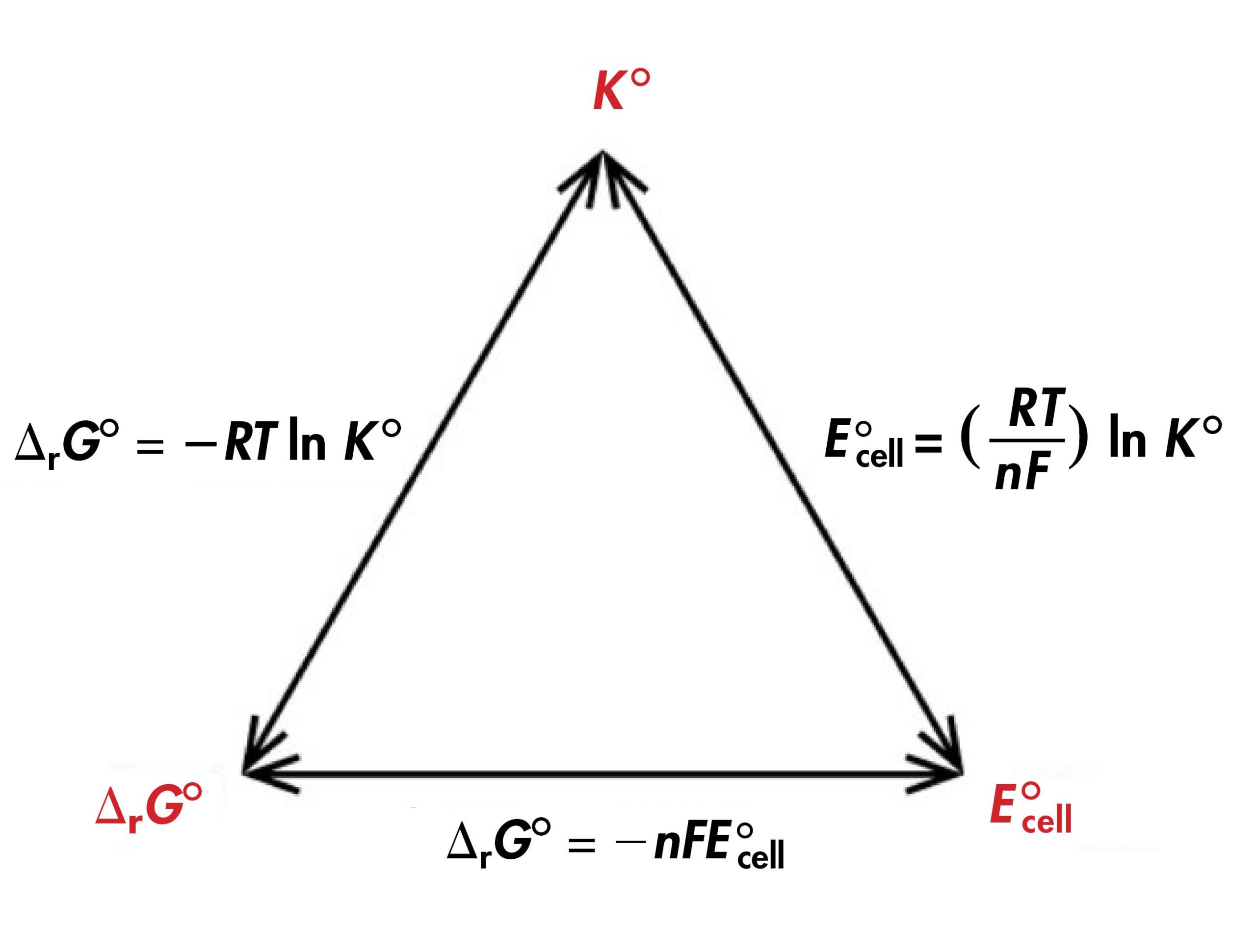
This equation also links standard cell potentials to equilibrium constants, since:
Therefore:
Thus, if any one of ΔrG°, K°, or E°cell is known or can be calculated for a redox reaction, the other two quantities can be determined using the relationships shown below. Moreover, any of the three quantities can be used to determine whether a reaction is product-favored at equilibrium.
Exercise: Standard Gibbs Free Energy Change for a Redox Reaction
Exercise: Gibbs Free Energy Change and Equilibrium Constant for a Redox Reaction
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂

