D26.1 Kinetic Metastability
We have used a simplified reaction energy diagram, as shown below, to help us think about the energies involved in the transformation of cis-1,2-difluoroethene molecules to trans-1,2-difluoroethene molecules.
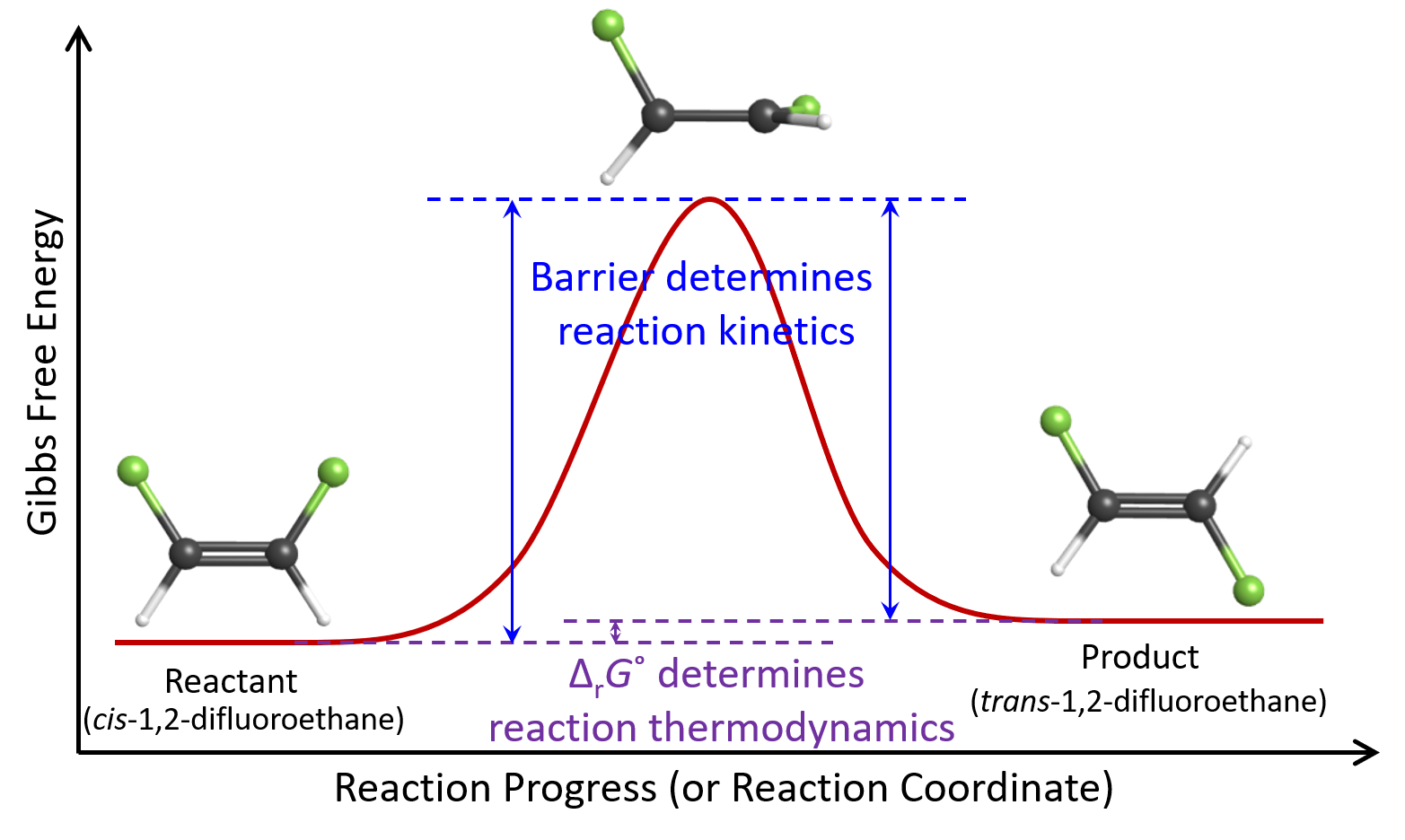
The thermodynamics of this reaction is dictated by the free energy difference (ΔrG°) between the reactant and the product. The kinetics of this reaction is influenced by the highest energy point in the reaction energy diagram: if a cis-1,2-difluoroethene molecule does not have energy higher than the highest energy point, the reaction will not occur. This “barrier” is the minimum energy needed to rotate about the double bond.
It is useful to distinguish between thermodynamic stability and kinetic metastability. When a reaction favors product(s) at equilibrium (ΔrG° < 0), we say that the product(s) are thermodynamically stable relative to the reactant(s). When a product-favored reaction proceeds so slowly that it is considered to not be occurring, we say that the reactant(s) are kinetically metastable (or kinetically stable) relative to the product(s). Note that stability (and metastability) are defined by comparing one substance with another: it is possible that a substance is stable relative to one substance, but unstable relative to another substance. Additionally, stability depends on the conditions the substance is under: a substance may be stable under one set of conditions but unstable under other conditions (such as changes in temperature or pressure).
For an example, consider again the reaction:
Here cis-1,2-difluoroethene has lower ΔfG° than trans-1,2-difluoroethene, hence ΔrG° of the forward reaction is positive and reactant is favored at equilibrium. In other words, cis-1,2-difluoroethene is thermodynamically stable relative to trans-1,2-difluoroethene. However, at room temperature, the reaction barrier is high enough that neither forward nor reverse reaction occurs. Therefore, trans-1,2-difluoroethene is kinetically metastable relative to cis-1,2-difluoroethene. We can describe trans-1,2-difluoroethene as being inert (unreactive) at room temperature. As we have seen earlier, if temperature is raised high enough, then the forward and reverse reaction will occur, and the resulting equilibrium will contain some fraction of both the cis and trans isomer, depending on the exact ΔrG° at that temperature.
In another example, consider diamond and graphite, two well-known substances. They are both composed solely of carbon atoms. The title of an old James Bond film, and an even older advertising slogan, says, “Diamonds Are Forever.” This implies some stability: we don’t expect the diamond in a ring to change anytime soon.
Exercise: Diamond and Graphite
Diamond is kinetically metastable relative to graphite. Thermodynamics says it should change to graphite, but, at the temperature of the human body, the reaction is so slow as to be essentially undetectable in a human lifetime. The fact that diamond exists is due to a very large energetic barrier for conversion of diamond to graphite: diamond would convert to graphite at temperatures above 4500 K.
This very large barrier, as well as the fact that diamond is one of the hardest known solids and graphite is one of the softest, can be explained by differences in the way the atoms are bonded. In diamond, every carbon atom has sp3 hybridization and each sp3 carbon is bonded to 4 other sp3 carbon atoms at the corners of a tetrahedron. In graphite, every carbon atom has sp2 hybridization and each sp2 carbon is bonded to 3 other sp2 carbon atoms in planar sheets of connected benzene rings. Because the sheets can slide past one another relatively easily, graphite is soft and slippery.

Conversion of diamond to graphite requires breaking numerous C−C single bonds with bond energy of 346 kJ/mol. Therefore, transforming diamond into graphite, or vice versa, requires almost as much energy as destroying the entire crystal lattice and rebuilding it. This is why once diamond is formed, it does not convert back to graphite under normal conditions: diamond is said to be metastable because its stability depends on kinetics, not thermodynamics.
Exercise: Kinetic Metastability
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂

