D34.3 Autoionization of Water
H2O is the base of its conjugate acid H3O+, and H2O is also the acid of its conjugate base OH–. (However, H3O+ is not the conjugate acid of OH–; these two species are not a conjugate acid-base pair because their structures do not differ by a single H+.)
As we have seen in the example acid-base reactions thus far, H2O can react as an acid or a base depending on the other species involved in the reaction. In pure water, H2O acts as both acid and base—a very small fraction of water molecules donate protons to other water molecules:
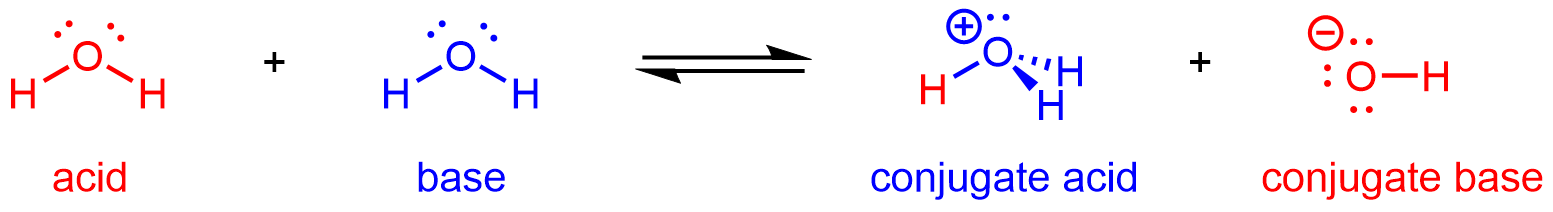
This type of reaction, in which a substance ionizes when one molecule of the substance reacts with another molecule of the same substance, is referred to as autoionization.
Pure water undergoes autoionization to a very slight extent at 25 °C. The equilibrium concentrations of H3O+ and OH– give an autoionization constant for water, Kw = 1.0 × 10−14 at 25 °C.
Because it is the mathematical product of concentrations of two ions, it is also called the ion-product constant for water.
Activity: Autoionization of Water
The subscript “w” in Kw denotes that this is the equilibrium constant for the reaction associated with the autoionization of water:
H2O(ℓ) + H2O(ℓ) ⇌ H3O+(aq) + OH–(aq)
Kw = [H3O+][OH–] = 1.0 × 10−14 at 25 °C; ΔrH° = 55.8 kJ/mol, ΔrS° = -80.7 J/mol
And just like any equilibrium constant, Kw is also temperature-dependent. Suppose you needed to predict quantitatively the value of Kw at 100 °C. Write in your notebook the way you would make the quantitative prediction (don’t actually calculate, just describe how you would solve the problem).
Write in your notebook, then left-click here for an explanation.
You know Kw and ΔrH° at 25 °C. To make a quantitative prediction for Kw at 100 °C, use the van’t Hoff equation:
This equation more directly shows the effect of temperature on equilibrium.
But perhaps you approached this problem by solving for ΔrG° at 100 °C using ΔrG° = ΔrH° – TΔrS°, and then found Kw via K° = e-ΔrG°/RT? You can check if both approach works in this situation by calculating the value of Kw at 100 °C both ways. Do the results agree?
Why is the sign of ΔrS° for the autoionization of water negative?
The polar water molecules will arrange around the ions to make a solvation shell. Ions with a stronger interaction with water molecules (for example, ions with a smaller/more concentrated charge, or ions with a higher charge) will lead to the formation of a more extensive solvation shell, which leads to a decrease in entropy.
The effect can be though of as:
(n)H2O ⇌ H+·(x)H2O + OH–·(n-x-1)H2O
Water is an example of an amphiprotic chemical species, a molecule that can either gain a proton or lose a proton in a Brønsted-Lowry acid-base reaction. Amphiprotic species are also amphoteric, a more general term for a species that may act either as an acid or a base by any definition (not just the Brønsted-Lowry definition). For example, the bicarbonate ion is also amphoteric:
HCO3–(aq) + H2O(ℓ) ⇌ H2CO3(aq) + OH–(aq)
Exercise: Autoionization of Water
As part (b) of the exercise above suggests, adding an acid in water will perturb the autoionization equilibrium, and the aqueous solution will reach new equilibrium concentrations in the process of re-establishing the equilibrium. This is an application of Le Chatelier’s principle.
For example, if we are to add a small amount of hydrochloric acid (HCl) to a sample of pure water, it would react as
HCl(aq) + H2O(ℓ) ⇌ H3O+(aq) + Cl–(aq)
which effectively increases the H3O+ concentration in autoionization reaction:
H2O(ℓ) + H2O(ℓ) ⇌ H3O+(aq) + OH–(aq)
This increase in one of the product concentrations leads to the reaction proceeding to form more reactants, effectively decreasing the OH– concentration, leading to the results you just calculated in part (b).
Similarly, adding a base to water would lead to a higher [OH¯] and a lower [H3O+] when the equilibrium is re-established.
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂 (Note that we cannot answer questions via the google form. If you have a question, please post it on Piazza.)
