D22.1 Entropy and Microstates
Activity: Entropy Change and Microstates
Atoms tend to spontaneously go to the lowest-energy state. For example, when an atom is excited by a high temperature, the excited state emits radiation and the atom returns to the ground state. Based on this observation, another student says that everything tends to go to the lowest energy state.
Consider these three thermochemical expressions for three substances that dissolve in water at 25 °C.
| NH4NO3(s) → NH4+(aq) + NO3−(aq) | ΔrH° = 25.69 kJ/mol |
| NaCl(s) → Na+(aq) + Cl−(aq) | ΔrH° = 3.87 kJ/mol |
| NaOH(s) → Na+(aq) + OH−(aq) | ΔrH° = −44.50 kJ/mol |
Based on the data given here, write a brief explanation of whether you agree with the student.
Write in your notebook, then left-click here for an explanation.
Deciding whether a process is spontaneous requires knowledge of the system’s enthalpy change, but that is not sufficient. It also requires knowledge of change in another property: entropy. (Similarly, deciding whether a process is product-favored requires knowledge of the system’s standard enthalpy change and standard entropy change.) The entropy change, ΔS, at constant temperature is defined as:
Here, qrev is the heat transfer of energy for a reversible process, a theoretical process that takes place such that its direction can be changed (it can be “reversed”) by an infinitesimally small change in some condition. An example of a reversible process is melting water at 0 °C and 1 bar, where liquid water and ice are at equilibrium. Raising the temperature a tiny bit causes the ice to melt; lowering the temperature a tiny bit reverses the process, causing liquid water to freeze.
On a molecular scale, the entropy of a system can be related to the number of possible microstates (W). A microstate is a specific configuration of the energies of a system. The relationship is:
where kB is the Boltzmann constant with a value of 1.38×10−23 J/K (or 8.314 J/mol·K).
Similar to enthalpy, the change in entropy for a process is the difference between its final (Sf) and initial (Si) values:
For processes involving an increase in the number of microstates, Wf > Wi, the entropy of the system increases, ΔS > 0. Conversely, processes that reduce the number of microstates, Wf < Wi, yield a decrease in system entropy, ΔS < 0.
Consider a simplistic case of a system comprised of four distinguishable particles distributed among two energetically-equivalent states, as illustrated in the figure below. The number of microstates possible for such a system is 24 = 16. Microstates with equivalent particle arrangements (not considering individual particle identities) are grouped together and are called distributions. The probability that a system exists as a given distribution is proportional to the number of microstates within the distribution. Because entropy increases logarithmically with the number of microstates, the most probable distribution is the one of greatest entropy.
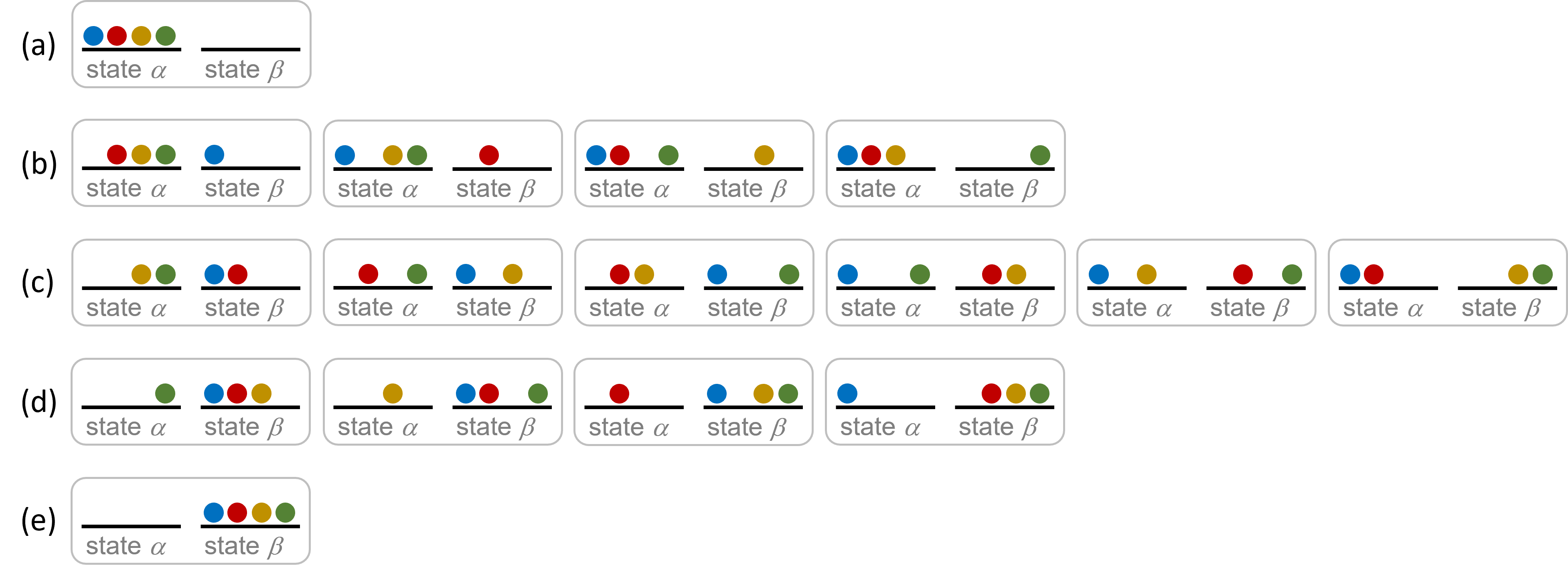
For the system in the figure above, the most probable distribution is (c), with the probability of finding the system in this configuration being [latex]\frac{6}{16}[/latex] or 37.5%. The least probable configuration of the system is distributions (a) and (e), where each distribution has a probability of [latex]\frac{1}{16}[/latex].
Activity: Entropy Change and Microstates
Consider another system as shown in the next figure. This system consists of two objects, AB and CD, and two units of energy (represented as “*”). Distribution (a) shows the three microstates possible for the initial state of the system, where both units of energy are contained within the hot AB object. If one energy unit is transferred, the result is distribution (b) consisting of four microstates. If both energy units are transferred, the result is distribution (c) consisting of three microstates.
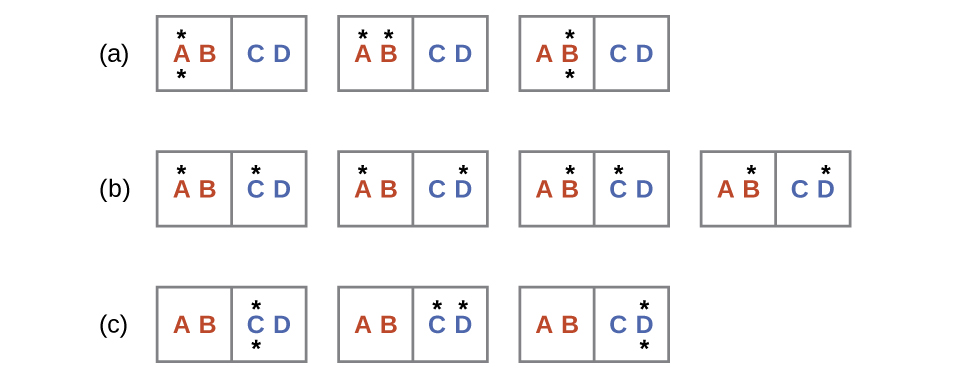
Hence, we may describe this system by a total of ten microstates. The probability that there is no heat transfer of energy when the two objects are brought into contact (the system remains in distribution (a)) is 30%. It is much more likely for heat transfer to occur and yield either distribution (b) or (c), the combined probability being 70%. The most likely result, with 40% probability, is heat transfer to yield the uniform dispersal of energy represented by distribution (b).
This simple example supports the common observation that placing hot and cold objects in contact results in heat transfer that ultimately equalizes the objects’ temperatures, at which point thermal equilibrium has been achieved. Such a process is characterized by an increase in system entropy.
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂 (Note that we cannot answer questions via the google form. If you have a question, please post it on Piazza.)

