Cognitive Load Theory and the Novice/Expert Divide

Cogntive Load Theory

In 1956 George Miller published “The Magical Number Seven, Plus or Minus Two: Some Limits on Our Capacity for Processing Information” and often cited article about the processing capabilities, or working memory, of adult humans.   Cognitive researchers John Sweller, Paul Ayres, Slava Kalyuga have expanded Miller’s work, adding complexity and further understanding of cognitive processing capabilities in their theory of cognitive load, or CLT for short. While the capacity of working memory probably can’t be distilled down into a number like 5-9 bits, for our purposes, it’s a useful simplification.

Novice/Expert Divide

One of the challenges of teaching learners who are novices in the discipline is, well, remembering that they are novices and they do not have the cognitive networks to efficiently and effectively integrated new information.  Take a look at the following images:

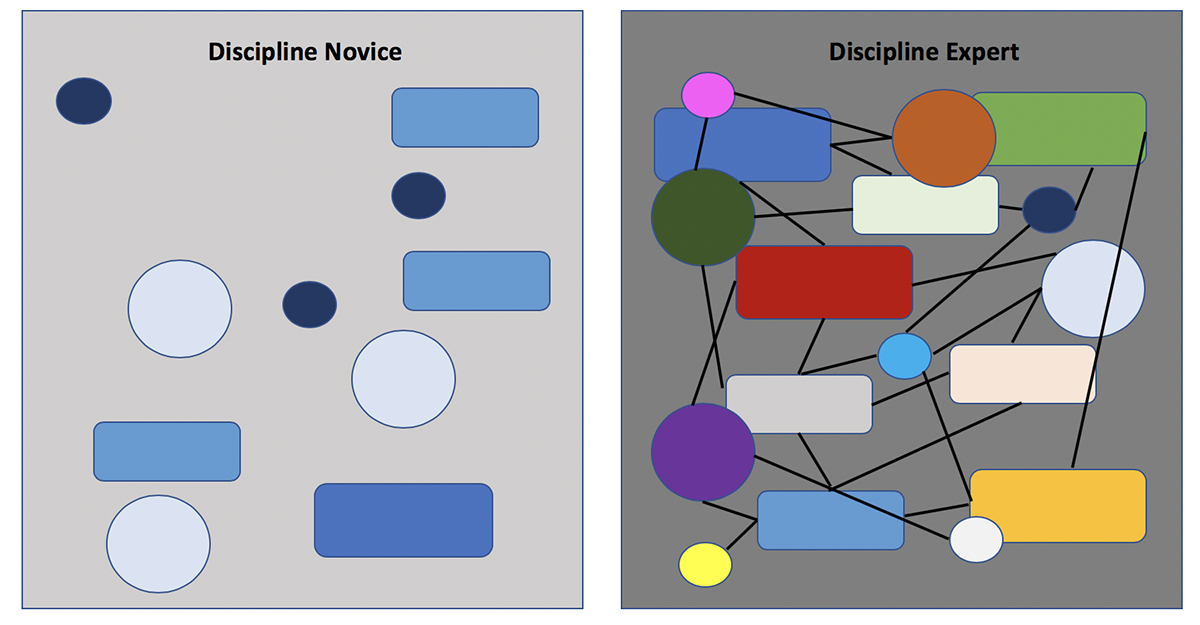
They’re not poorly rendered abstract art.  Rather, they’re an abstraction of the difference between the cogntive networks of a disicpline novice and a discipline expert.  A novice acks the cognitive architecture that discipline experts possess. Their background knowledge is often shaky and disconnected, and when they recall declarative knowledge and apply it with procedural knowledge they are experiencing these as discreet inputs in their working memory. Which means, those 7 “slots” fill up rather quickly.

An expert has highly interconnected cognitive networks, meaning the expert can “chunk” declarative and procedural knowledge together in a variety of different ways depending on the context of the task.  That means an expert more readily, even automatically, imposes meaning and order.  That also means an expert might only need to engage 2 “slots,” leaving 5 more  to deal with novel inputs, like metacognition, processess, or new knolwedge.

Here’s and example.  Think about writing the word, “cat.”  I’m assuming you really don’t need to think about it.  If you want to write cat, you just write it automatically.  Think about a 4 or 5-year-old writing cat.  Consider all the operations a typical, novice speller and writer has to go through to write “cat.”

1)Apply Proper Pencil grip; 2) Conceptualize idea of “Cat”; 3) Decode word into discreet sounds; 4) Translate first sound into symbol; 5) From first symbol; 6) Sequence next sound; 7) Form next sound

You can even see those “slots” fill up quickly when children first start spelling.  They often skip letters, especially vowels.

If you’ve looked at enough physics students’ problem solving work you’d probably see the same types of patterns.  They skip steps, they don’t cluster disparate pieces of information into chunks, which means they may not readily understand a concept or a process holistically.  In the next section, we’ll talk about instructional practices help students progress.