50 Valence Bond Theory and Hybridization (M9Q3)

Introduction

This section explores valence bond theory and orbital hyrbidization. We will first explore valence bond theory. Then we will look at orbital hybridization for molecules that contain single, double, and triple bonds. The section below provides a more detailed description of these topics, worked examples, practice problems and a glossary of important terms.

Learning Objectives for Valence Bond Theory and Hybridization

 

| Key Concepts and Summary | Glossary | End of Section Exercises |

Valence Bond Theory

As we know, a scientific theory is a strongly supported explanation for observed natural laws or large bodies of experimental data. For a theory to be accepted, it must explain experimental data and be able to predict behavior. For example, the VSEPR model has gained widespread acceptance because of its simplicity and its ability to predict the three-dimensional molecular shapes of many molecules that are consistent with experimental data. The VSEPR model, however, does not accurately predict all molecular shapes or electron domain geometries. Furthermore, VSEPR does not provide an explanation of chemical bonding.

Valence bond theory describes a covalent bond as the overlap of singly-occupied atomic orbitals that yield a pair of electrons shared between the two bonded atoms. We say that orbitals on two different atoms overlap when a portion of one orbital and a portion of a second orbital occupy the same region of space. According to valence bond theory, a covalent bond results when two conditions are met: (1) an orbital on one atom overlaps an orbital on a second atom and (2) the single electrons in each orbital combine to form an electron pair. The mutual attraction between this negatively charged electron pair and the two atoms’ positively charged nuclei contributes to the link between the two atoms that we define as a covalent bond. The strength of a covalent bond depends on the extent of overlap of the orbitals involved. Orbitals that overlap extensively form bonds that are stronger than those that have less overlap.

The energy of the system depends on how much the orbitals overlap. Figure 1 illustrates how the sum of the energies of two hydrogen atoms (the colored curve) changes as they approach each other. When the atoms are infinitely far apart there is no overlap, and by convention we set the sum of the energies at zero. Even at large distances between the atoms there is some small stabilizing interaction which is why the graph only approaches zero at real distances. As the atoms move closer together, their orbitals overlap more effectively forming a stronger covalent bond between the nuclei, which lowers the energy of the system. Covalent bond formation stabilizes the system until the optimum bond distance is achieved. At distances closer than the optimum bond distance, the nuclear-nuclear repulsion of the two nuclei increase and destabilize the system, as shown at the far left of Figure 1. The optimum bond distance is largely due to a compromise between two opposing factors, orbital overlap stabilizing the system and nuclear-nuclear repulsion destabilizing the system as the internuclear distance decreases.

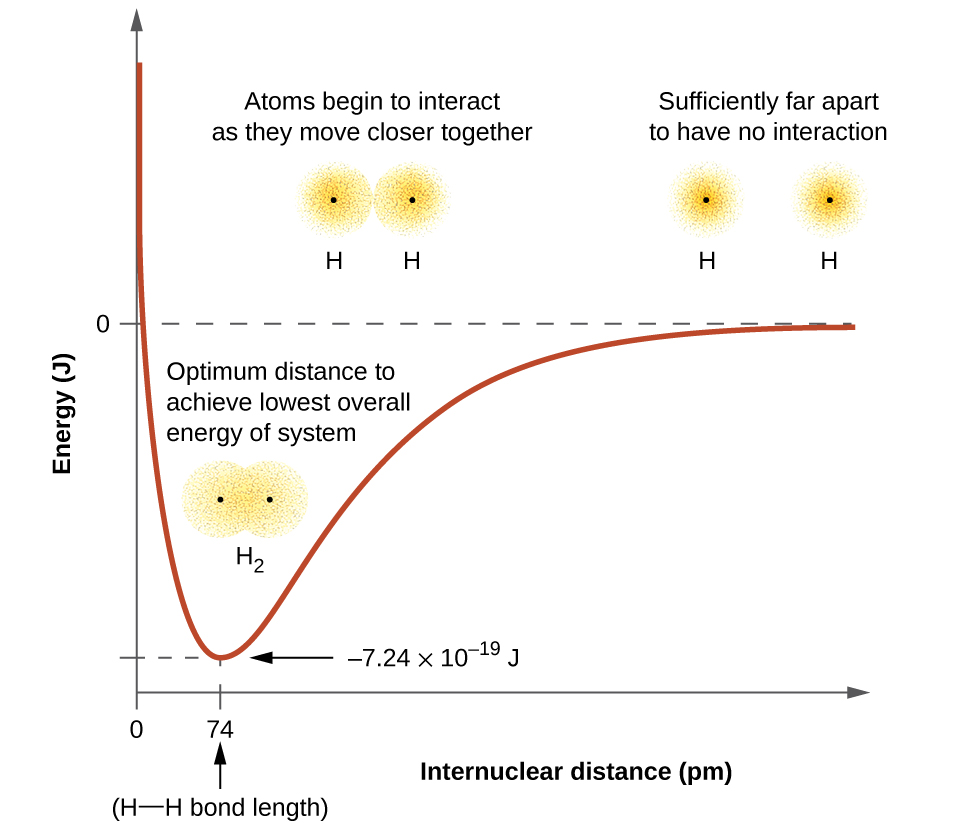
A pair of diagrams are shown and labeled “a” and “b”. Diagram a shows three consecutive images. The first image depicts two separated blurry circles, each labeled with a positive sign and the term “H atom.” The phrase written under them reads, “Sufficiently far apart to have no interaction.” The second image shows the same two circles, but this time they are much closer together and are labeled, “Atoms begin to interact as they move closer together.” The third image shows the two circles overlapping, labeled, “H subscript 2,” and, “Optimum distance to achieve lowest overall energy of system.” Diagram b shows a graph on which the y-axis is labeled “Energy ( J ),” and the x-axis is labeled, “Internuclear distance ( p m ).” The midpoint of the y-axis is labeled as zero. The curve on the graph begins at zero p m and high on the y-axis. The graph slopes downward steeply to a point far below the zero joule line on the y-axis and the lowest point reads “0.74 p m” and “H bonded to H bond length.” It is also labeled “ negative 7.24 times 10 superscript negative 19 J.” The graph then rises again to zero J. The graph is accompanied by the same images from diagram a; the first image correlates to the point in the graph where it crosses the zero point on the y-axis, the third image where the graph is lowest.
Figure 1. (a) The interaction of two hydrogen atoms changes as a function of distance. (b) The energy of the system changes as the atoms interact. The lowest (most stable) energy occurs at a distance of 74 pm, which is the bond length observed for the H2 molecule.

The bond energy is the difference between the energy minimum (which occurs at the bond distance) and the energy of the two separated atoms. This is the quantity of energy released when the bond is formed. Conversely, the same amount of energy is required to break the bond. For the H2 molecule shown in Figure 1, at the bond distance of 74 pm the system is 7.24 × 10−19 J lower in energy than the two separated hydrogen atoms. This may seem like a small number. However, we know from our earlier description of thermochemistry that bond energies are often discussed on a per-mole basis. For example, it requires 7.24 × 10−19 J to break one H–H bond, but it takes 4.36 × 105 J to break 1 mole of H–H bonds. A comparison of some bond lengths and energies is shown in Table 1. We can find many of these bonds in a variety of molecules, and this table provides average values. For example, breaking the first C–H bond in CH4 requires 439.3 kJ/mol, while breaking the first C–H bond in H–CH2C6H5 (a common paint thinner) requires 375.5 kJ/mol.

Bond Length (pm) Energy (kJ/mol) Bond Length (pm) Energy (kJ/mol)
H–H 74 436 C–O 143 336
H–C 110 416 C=O 121 695
H–N 98 391 C≡O 113 1073
H–O 94 467 H–Cl 127 431
C–C 154 356 H–Br 142 366
C=C 134 598 H–I 161 299
C≡C 121 813 O–O 148 146
C–N 147 285 O=O 121 498
C=N 127 616 F–F 128 158
C≡N 115 866 Cl–Cl 200 242
Table 1. Representative Bond Energies and Lengths

In addition to the distance between two orbitals, the orientation of orbitals also affects their overlap (other than for two s orbitals, which are spherically symmetric). Greater overlap is possible when orbitals are oriented such that they overlap on a direct line between the two nuclei. Figure 2 illustrates this for two p orbitals from different atoms; the overlap is greater when the orbitals overlap end to end rather than at an angle.

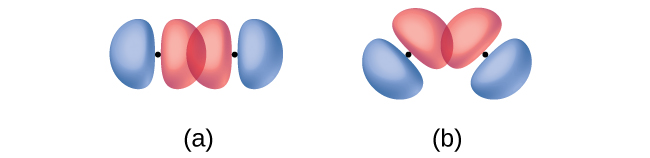
Two diagrams are shown. Diagram a contains two molecules whose p orbitals, which are depicted as two balloon-shaped structures that meet together to form a peanut shape, are laid end over end, creating an area of overlap. In diagram b, the same two molecules are shown, but this time, they are laid out in a way so as to form a near-ninety degree angle. In this diagram, the ends of two of these peanut-shaped orbitals do not overlap nearly as much.
Figure 2. (a) The overlap of two p orbitals is greatest when the orbitals are directed end to end. (b) Any other arrangement results in less overlap. The dots indicate the locations of the nuclei.

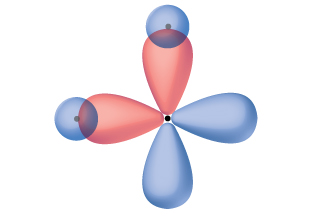
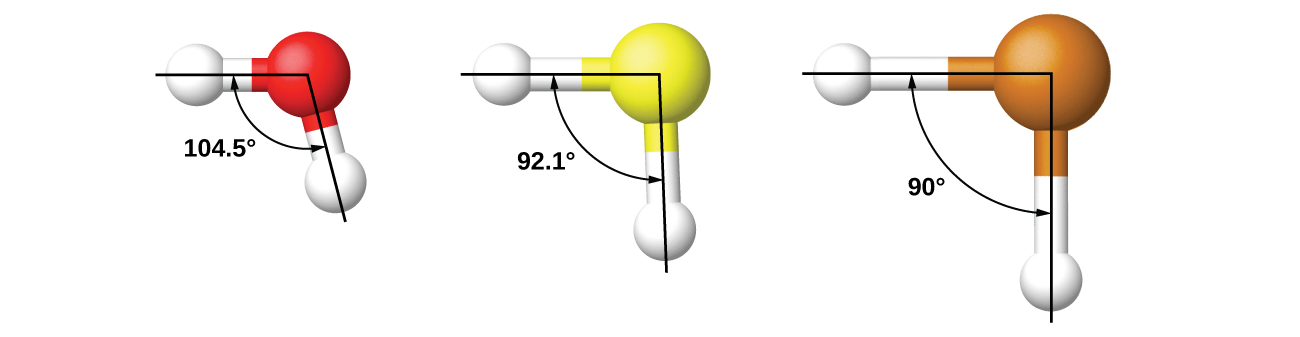
Thinking in terms of overlapping atomic orbitals is one way for us to explain how chemical bonds form in diatomic molecules. However, to understand how molecules with more than two atoms form stable bonds, we require a more detailed model. As an example, let us consider the water molecule, in which we have one oxygen atom bonding to two hydrogen atoms. Oxygen has the electron configuration 1s22s22p4, with two unpaired electrons (one in each of two 2p orbitals). Valence bond theory would predict that the two O–H bonds form from the overlap of these two 2p orbitals with the 1s orbitals of the hydrogen atoms. If this were the case, the bond angle would be 90°, as shown in Figure 3, because p orbitals are perpendicular to each other. Experimental evidence shows that the bond angle is 104.5°, not 90°. The prediction of the valence bond theory alone does not match the real-world observations of a water molecule; a different model is needed.

Two peanut-shaped orbitals lie perpendicular to one another. They overlap with spherical orbitals to the left and top of the diagram.
Figure 3. The hypothetical overlap of two of the 2p orbitals on an oxygen atom (red) with the 1s orbitals of two hydrogen atoms (blue) would produce a bond angle of 90°. This is not consistent with experimental evidence.[1]

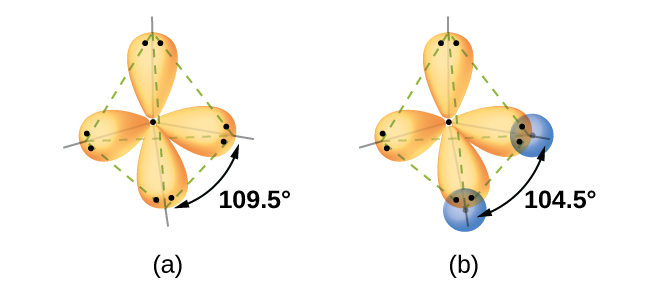
Quantum-mechanical calculations suggest why the observed bond angles in H2O differ from those predicted by the overlap of the 1s orbital of the hydrogen atoms with the 2p orbitals of the oxygen atom. The mathematical expression known as the wave function, ψ, contains information about each orbital and the wavelike properties of electrons in an isolated atom. When atoms are bound together in a molecule, the wave functions combine to produce new mathematical descriptions that have different shapes. This process of combining the wave functions for atomic orbitals is called hybridization and is mathematically accomplished by the linear combination of atomic orbitals, LCAO, (a technique that we will encounter again later). The new orbitals that result are called hybrid orbitals. The valence orbitals in an isolated oxygen atom are a 2s orbital and three 2p orbitals. The valence orbitals in an oxygen atom in a water molecule differ; they consist of four equivalent hybrid orbitals that point approximately toward the corners of a tetrahedron (Figure 4). Consequently, the overlap of the O and H orbitals should result in a tetrahedral bond angle (109.5°).

Two diagrams are shown and labeled “a” and “b.” Diagram a shows two peanut-shaped orbitals lying in a tetrahedral arrangement around the letter “O.” Diagram b shows the same two orbitals, but they now overlap to the top and to the left with two spherical orbitals, each labeled “H.” A pair of electrons occupies each lobe of the peanut-shaped orbitals.
Figure 4. (a) A water molecule has four regions of electron density, so VSEPR theory predicts a tetrahedral arrangement of hybrid orbitals. (b) Two of the hybrid orbitals on oxygen contain lone pairs, and the other two overlap with the 1s orbitals of hydrogen atoms to form the O–H bonds in H2O. This description is more consistent with the experimental structure.

The following ideas are important in understanding hybridization:

  1. Hybrid orbitals do not exist in isolated atoms. They are formed only in covalently bonded atoms.
  2. Hybrid orbitals have shapes and orientations that are very different from those of the atomic orbitals in isolated atoms.
  3. A set of hybrid orbitals is generated by combining atomic orbitals. The number of hybrid orbitals in a set is equal to the number of atomic orbitals that were combined to produce the set.
  4. All orbitals in a set of hybrid orbitals are equivalent in shape and energy.
  5. The type of hybrid orbitals formed in a bonded atom depends on its electron-pair geometry as predicted by the VSEPR theory.
  6. Hybrid orbitals overlap to form σ bonds. Unhybridized orbitals overlap to form π bonds. This will be discussed in the next section.

In the following sections, we shall discuss the common types of hybrid orbitals.

sp Hybridization

The beryllium atom in a gaseous BeCl2 molecule is an example of a central atom with no lone pairs of electrons in a linear arrangement of three atoms. There are two regions of valence electron density in the BeCl2 molecule that correspond to the two covalent Be–Cl bonds. To accommodate these two electron domains, two of the Be atom’s four valence orbitals will mix to yield two hybrid orbitals. This hybridization process involves mixing of the valence s orbital with one of the valence p orbitals to yield two equivalent sp hybrid orbitals that are oriented in a linear geometry (Figure 5). In this figure, the set of sp orbitals appears similar in shape to the original p orbital, but there is an important difference. The number of atomic orbitals combined always equals the number of hybrid orbitals formed. The p orbital is one orbital that can hold up to two electrons. The sp set is two equivalent orbitals that point 180° from each other. The two electrons that were originally in the s orbital are now distributed to the two sp orbitals, which are half filled. In gaseous BeCl2, these half-filled hybrid orbitals will overlap with orbitals from the chlorine atoms to form two identical bonds.

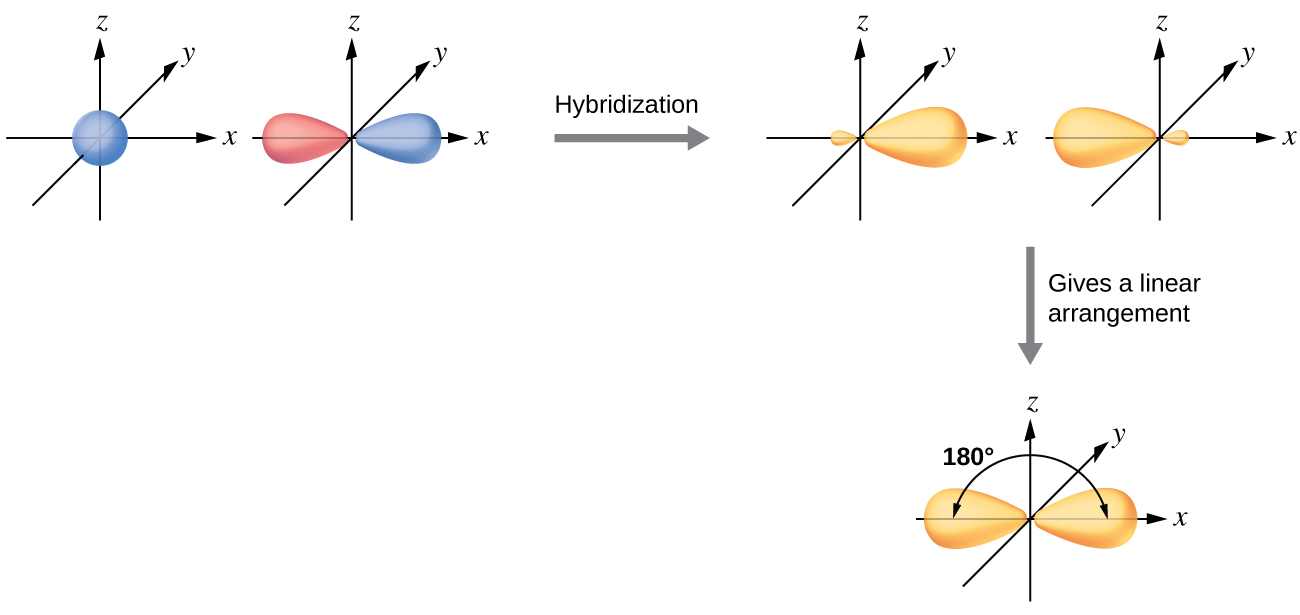
A series of three diagrams connected by a right-facing arrow that is labeled, “Hybridization,” and a downward-facing arrow labeled, “Gives a linear arrangement,” are shown. The first diagram shows a blue spherical orbital and a red, peanut-shaped orbital, each placed on an X, Y, Z axis system. The second diagram shows the same two orbitals, but they are now purple and have one enlarged lobe and one smaller lobe. Each lies along the x-axis in the drawing. The third diagram shows the same two orbitals, but their smaller lobes now overlap along the x-axis while their larger lobes are located at and labeled as “180 degrees” from one another.
Figure 5. Hybridization of an s orbital (blue) and a p orbital (red/blue) of the same atom produces two sp hybrid orbitals (yellow). Each hybrid orbital is oriented primarily in just one direction. Note that each sp orbital contains one lobe that is significantly larger than the other. The set of two sp orbitals are oriented at 180°, which is consistent with the geometry for two domains.

We illustrate the electronic differences in an isolated Be atom and in the bonded Be atom in the orbital energy-level diagram in Figure 6. These diagrams represent each orbital by a horizontal line (indicating its energy) and each electron by an arrow. Energy increases toward the top of the diagram. We use one upward arrow to indicate one electron in an orbital and two arrows (up and down) to indicate two electrons of opposite spin.

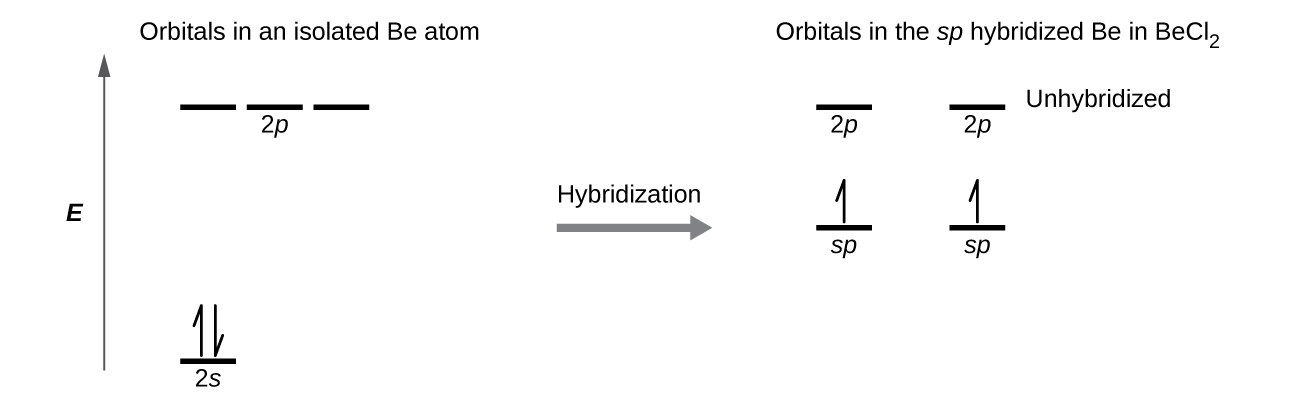
A diagram is shown in two parts, connected by a right facing arrow labeled, “Hybridization.” The left diagram shows an up-facing arrow labeled, “E.” To the lower right of the arrow is a short, horizontal line labeled, “2 s,” that has two vertical half-arrows facing up and down on it. To the upper right of the arrow are a series of three short, horizontal lines labeled, “2 p.” Above these two sets of lines is the phrase, “Orbitals in an isolated B e atom.” The right side of the diagram shows two short, horizontal lines placed halfway up the space and each labeled, “s p.” An upward-facing half arrow is drawn vertically on each line. Above these lines are two other short, horizontal lines, each labeled, “2 p.” Above these two sets of lines is the phrase, “Orbitals in the s p hybridized B e in B e C l subscript 2.”
Figure 6. This orbital energy-level diagram shows the sp hybridized orbitals on Be in the linear BeCl2 molecule. Each of the two sp hybrid orbitals holds one electron and is thus half filled and available for bonding via overlap with a Cl 3p orbital.

When atomic orbitals hybridize, the valence electrons occupy the newly created orbitals. The Be atom had two valence electrons, so each of the sp orbitals gets one of these electrons. Each of these electrons pairs up with the unpaired electron on a chlorine atom when a hybrid orbital and a chlorine orbital overlap during the formation of the Be–Cl bonds.

Any central atom surrounded by just two regions of valence electron density in a molecule will exhibit sp hybridization. Other examples include the mercury atom in the linear HgCl2 molecule, the zinc atom in Zn(CH3)2, which contains a linear C–Zn–C arrangement, and the carbon atoms in HCN, HCCH, and CO2.

 

Check out the University of Wisconsin-Oshkosh website to learn about visualizing hybrid orbitals in three dimensions.

sp2 Hybridization

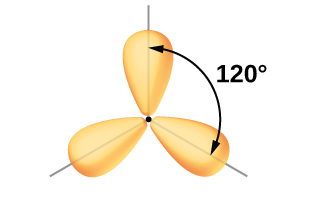
The valence orbitals of a central atom surrounded by three regions of electron density consist of a set of three sp2 hybrid orbitals and one unhybridized p orbital. This arrangement results from sp2 hybridization, the mixing of one s orbital and two p orbitals to produce three identical hybrid orbitals oriented in a trigonal planar geometry (Figure 7).

A series of three diagrams connected by a right-facing arrow that is labeled, “Hybridization,” and a downward-facing arrow labeled, “Gives a trigonal planar arrangement,” are shown. The first diagram shows a blue spherical orbital and two red, peanut-shaped orbitals, each placed on an X, Y, Z axis system. The two red orbitals are located on the x and z axes, respectively. The second diagram shows the same three orbitals, but they are now purple and have one enlarged lobe and one smaller lobe. Each lies in a different axis in the drawing. The third diagram shows the same three orbitals, but their smaller lobes now overlap while their larger lobes are located at and labeled as “120 degrees” from one another.
Figure 7. The hybridization of an s orbital (blue) and two p orbitals (red/blue) produces three equivalent sp2 hybridized orbitals (yellow) oriented at 120° with respect to each other. The remaining unhybridized p orbital is not shown here, but is located along the z axis.

Although quantum mechanics yields the “plump” orbital lobes as depicted in Figure 7, sometimes for clarity these orbitals are drawn thinner and without the minor lobes, as in Figure 8, to avoid obscuring other features of a given illustration. We will use these “thinner” representations whenever the true view is too crowded to easily visualize.

Three balloon-like orbitals are shown, and connect together near their narrower ends in one plane. The angle between a pair of lobes is labeled, “120 degrees.”
Figure 8. This alternate way of drawing the trigonal planar sp2 hybrid orbitals is sometimes used in more crowded figures.

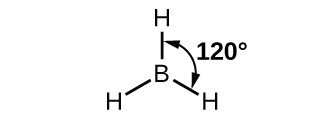
The observed structure of the borane molecule, BH3, suggests sp2 hybridization for boron in this compound. The molecule is trigonal planar, and the boron atom is involved in three bonds to hydrogen atoms (Figure 9). We can illustrate the comparison of orbitals and electron distribution in an isolated boron atom and in the bonded atom in BH3 as shown in the orbital energy level diagram in Figure 10. We redistribute the three valence electrons of the boron atom in the three sp2 hybrid orbitals, and each boron electron pairs with a hydrogen electron when B–H bonds form.

A boron atom is shown connected to three hydrogen atoms, which are arranged around it like a pyramid. The angle from one line connecting the boron atom to a hydrogen atom to another line connecting the boron atom to a hydrogen atom is labeled, “120 degrees.”
Figure 9. BH3 is an electron-deficient molecule with a trigonal planar structure.
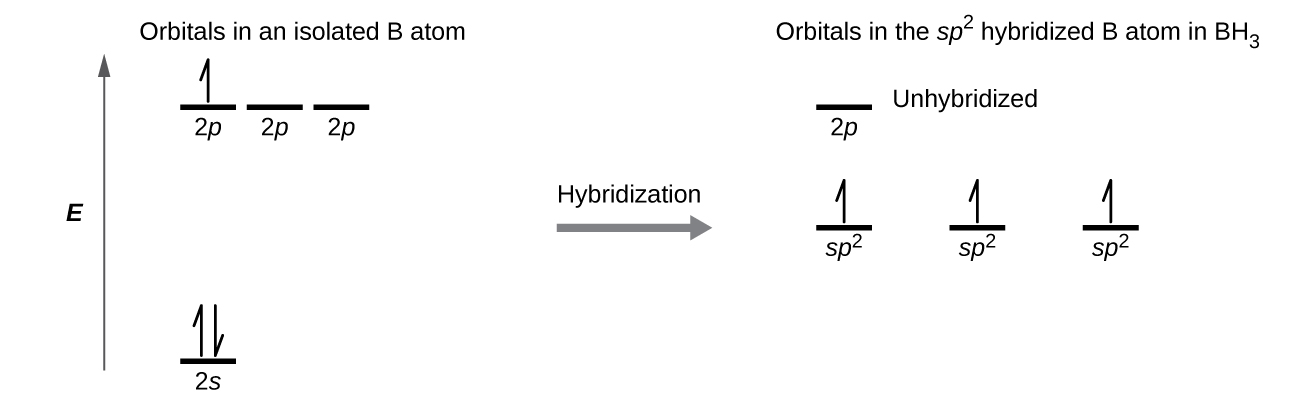
A diagram is shown in two parts, connected by a right facing arrow labeled, “Hybridization.” The left diagram shows an up-facing arrow labeled “E.” To the lower right of the arrow is a short, horizontal line labeled, “2 s,” that has two vertical half-arrows facing up and down on it. To the upper right of the arrow are a series of three short, horizontal lines labeled, “2 p.” Above both sets of these lines is the phrase, “Orbitals in an isolated B atom.” One of the lines has a vertical, up-facing arrow drawn on it. The right side of the diagram shows three short, horizontal lines placed halfway up the space and each labeled, “s p superscript 2.” An upward-facing half arrow is drawn vertically on each line. Above these lines is one other short, horizontal line, labeled, “2 p.” Above both sets of lines is the phrase, “Orbitals in the s p superscript 2 hybridized B atom in B H subscript 3.”
Figure 10. In an isolated B atom, there are one 2s and three 2p valence orbitals. When boron is in a molecule with three regions of electron density, three of the orbitals hybridize and create a set of three sp2 orbitals and one unhybridized 2p orbital. The three half-filled hybrid orbitals each overlap with an orbital from a hydrogen atom to form three single bonds in BH3.

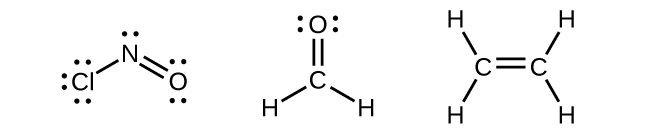
Any central atom surrounded by three regions of electron density will exhibit sp2 hybridization. This includes molecules with a lone pair on the central atom, such as ClNO (Figure 11), or molecules with two single bonds and a double bond connected to the central atom, as in formaldehyde, CH2O, and ethene, H2CCH2.

Three Lewis structures are shown. The left-hand structure shows a chlorine atom surrounded by three lone pairs of electrons single bonded to a nitrogen atom with one lone pair of electrons and double bonded to an oxygen atom with two lone pairs of electrons. The middle structure shows a carbon atom single bonded to two hydrogen atoms and double bonded to an oxygen atom that has two lone pairs of electrons. The right-hand structure shows two carbon atoms, double bonded to one another and each single bonded to two hydrogen atoms.
Figure 11. The central atom(s) in each of the structures shown contain three regions of electron density and are sp2 hybridized. As we know from the discussion of VSEPR theory, a region of electron density contains all of the electrons that point in one direction. A lone pair, an unpaired electron, a single bond, or a multiple bond would each count as one region of electron density.

sp3 Hybridization

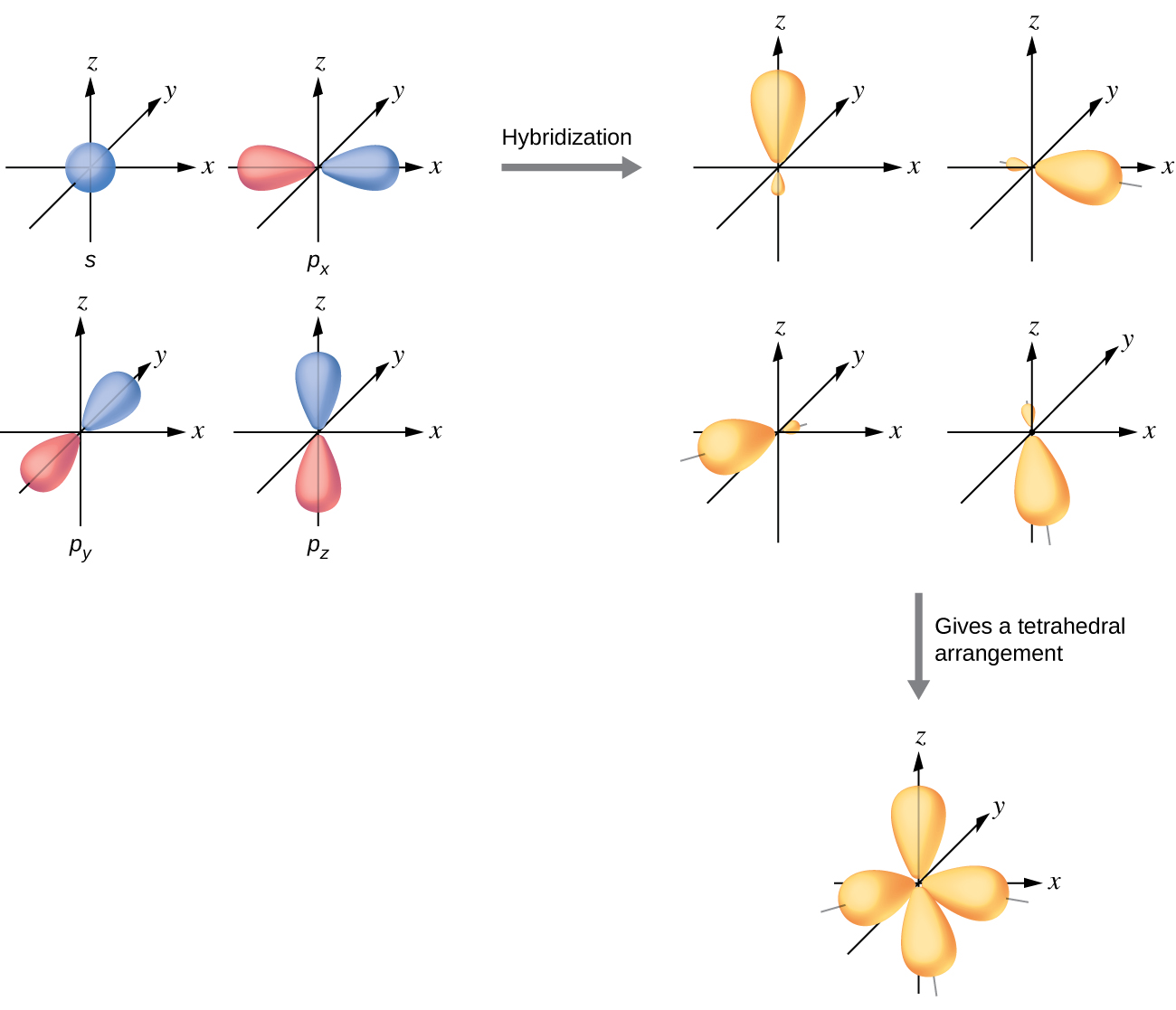
The valence orbitals of an atom surrounded by a tetrahedral arrangement of bonding pairs and lone pairs consist of a set of four sp3 hybrid orbitals. The hybrids result from the mixing of one s orbital and all three p orbitals that produces four identical sp3 hybrid orbitals (Figure 12). Each of these hybrid orbitals points toward a different corner of a tetrahedron.

A series of three diagrams connected by a right-facing arrow that is labeled, “Hybridization,” and a downward-facing arrow labeled, “Gives a tetrahedral arrangement,” are shown. The first diagram shows a blue spherical orbital and three red, peanut-shaped orbitals, each placed on an x, y, z axis system. The three red orbitals are located on the x , y and z axes, respectively. The second diagram shows the same four orbitals, but they are now purple and have one enlarged lobe and one smaller lobe. Each lies in a different axis in the drawing. The third diagram shows the same four orbitals, but their smaller lobes now overlap to form a tetrahedral structure.
Figure 12. The hybridization of an s orbital (blue) and three p orbitals (red/blue) produces four equivalent sp3 hybridized orbitals (yellow) oriented at 109.5° with respect to each other.

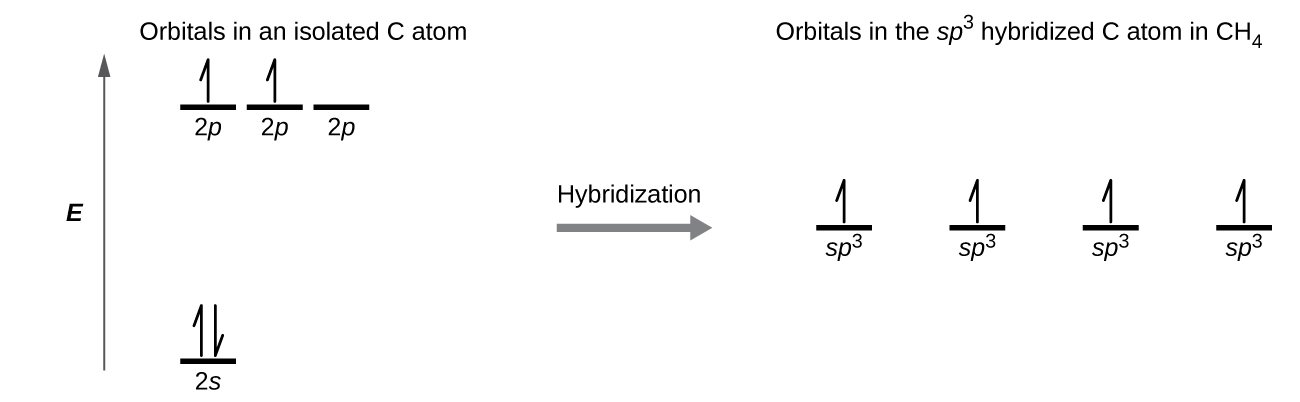
A molecule of methane, CH4, consists of a carbon atom surrounded by four hydrogen atoms at the corners of a tetrahedron. The carbon atom in methane exhibits sp3 hybridization. We illustrate the orbitals and electron distribution in an isolated carbon atom and in the bonded atom in CH4 in Figure 13. The four valence electrons of the carbon atom are distributed equally in the hybrid orbitals, and each carbon electron pairs with a hydrogen electron when the C–H bonds form.

A diagram is shown in two parts, connected by a right facing arrow labeled, “Hybridization.” The left diagram shows an up-facing arrow labeled “E.” To the lower right of the arrow is a short, horizontal line labeled, “2 s,” that has two vertical half-arrows facing up and down on it. To the upper right of the arrow are a series of three short, horizontal lines labeled, “2 p.” Two of the lines have a vertical, up-facing arrow drawn on them. Above both sets of lines is the phrase, “Orbitals in an isolated C atom.” The right side of the diagram shows four short, horizontal lines placed halfway up the space and each labeled, “s p superscript 3.” An upward-facing half arrow is drawn vertically on each line. Above these lines is the phrase, “Orbitals in the s p superscript 3 hybridized C atom in C H subscript 4.”
Figure 13. The four valence atomic orbitals from an isolated carbon atom all hybridize when the carbon bonds in a molecule like CH4 with four regions of electron density. This creates four equivalent sp3 hybridized orbitals. Overlap of each of the hybrid orbitals with a hydrogen orbital creates a C–H bond.

In a methane molecule, the 1s orbital of each of the four hydrogen atoms overlaps with one of the four sp3 orbitals of the carbon atom to form a bond. This results in the formation of four strong, equivalent covalent bonds between the carbon atom and each of the hydrogen atoms to produce the methane molecule, CH4.

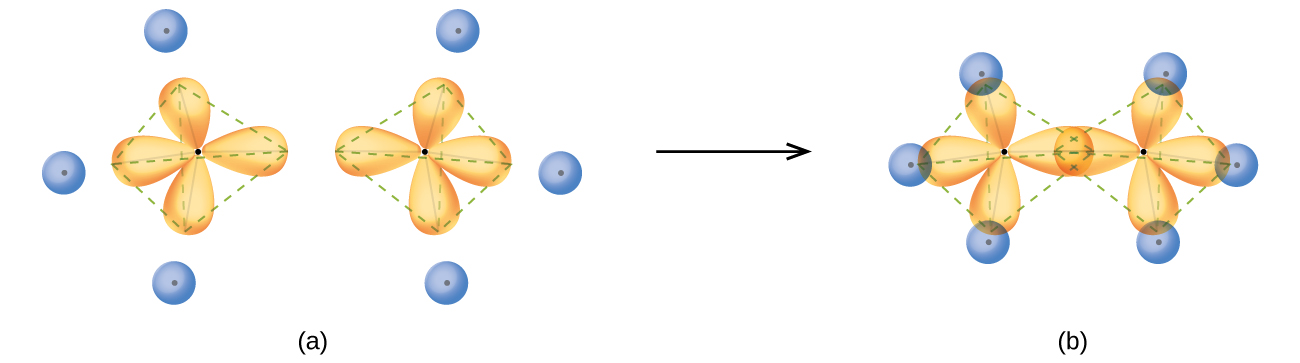
The structure of ethane, C2H6, is similar to that of methane in that each carbon in ethane has four neighboring atoms arranged at the corners of a tetrahedron—three hydrogen atoms and one carbon atom (Figure 14). However, in ethane an sp3 orbital of one carbon atom overlaps end to end with an sp3 orbital of a second carbon atom to form a bond between the two carbon atoms. Each of the remaining sp3 hybrid orbitals overlaps with an s orbital of a hydrogen atom to form carbon–hydrogen bonds. The structure and overall outline of the bonding orbitals of ethane are shown in Figure 14. The orientation of the two CH3 groups is not fixed relative to each other. Experimental evidence shows that rotation around single bonds occurs easily.

Two diagrams are shown and labeled “a” and “b.” Diagram a shows two carbon atoms, each surrounded by their four s p subscript three hybridized orbitals in a three dimensional arrangement. Each of the orbitals is shown overlapping with a spherical hydrogen atom. Diagram b shows the same general arrangement, but the hydrogen atoms are just represented by an, “H” and their spherical orbitals are not shown.
Figure 14. (a) In the ethane molecule, C2H6, each carbon has four sp3 orbitals. (b) These four orbitals overlap to form seven single bonds.

An sp3 hybrid orbital can also hold a lone pair of electrons. For example, the nitrogen atom in ammonia is surrounded by three bonding pairs and a lone pair of electrons directed to the four corners of a tetrahedron. The nitrogen atom is sp3 hybridized with one hybrid orbital occupied by the lone pair.

Since lone pairs occupy more space than bonding pairs, structures that contain lone pairs have bond angles slightly distorted from the ideal. Perfect tetrahedra have angles of 109.5°, but the observed angles, such as in ammonia (107.3°), are slightly smaller. Other examples of sp3 hybridization include CCl4, PCl3, and NCl3.

Assigning Hybrid Orbitals to Central Atoms

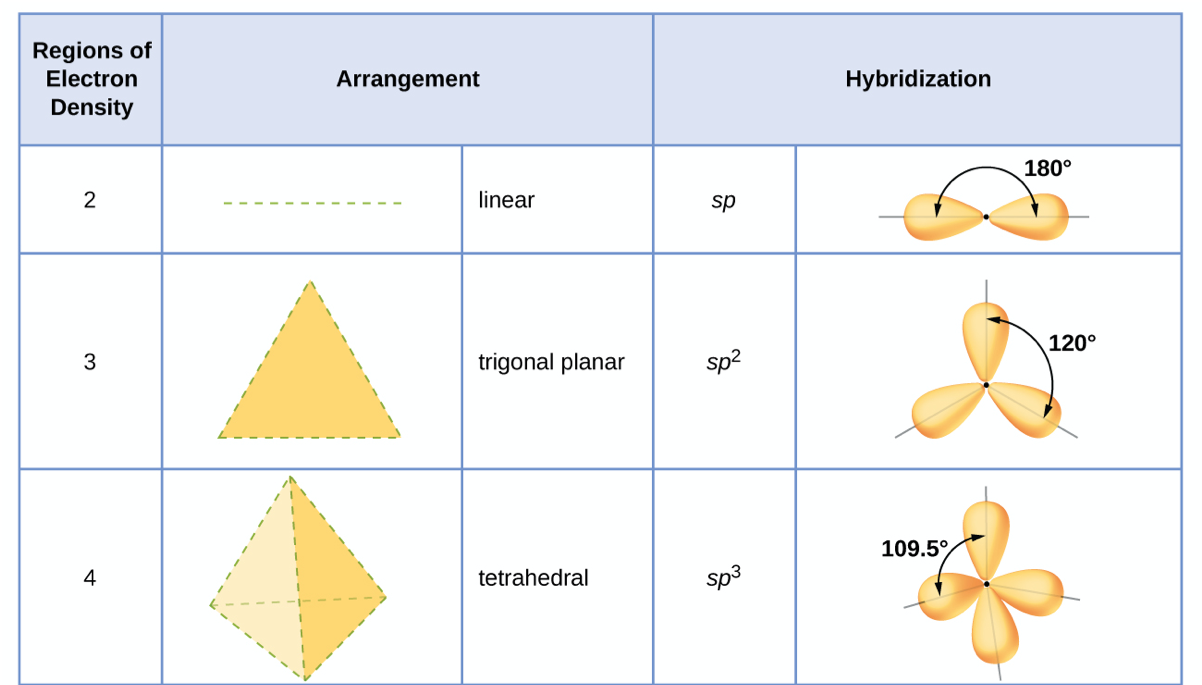
The hybridization of an atom is determined based on the number of regions of electron density that surround it. The geometrical arrangements characteristic of the various sets of hybrid orbitals are shown in Figure 15. These arrangements are identical to those of the electron-pair geometries predicted by VSEPR theory. VSEPR theory predicts the shapes of molecules, and hybrid orbital theory provides an explanation for how those shapes are formed. To find the hybridization of a central atom, we can use the following guidelines:

  1. Determine the Lewis structure of the molecule.
  2. Determine the number of regions of electron density around an atom using VSEPR theory, in which single bonds, multiple bonds, radicals, and lone pairs each count as one region.
  3. Assign the set of hybridized orbitals from Figure 15 that corresponds to this geometry.
A table is shown that is composed of five columns and four rows. The header row contains the phrases, “Regions of electron density,” “Arrangement,” (which has two columns below it), and “Hybridization,” (which has two columns below it). The first column contains the numbers “2,” “3,” and “4.” The second column contains images of a line, a triangle, and a three sided pyramid. The third column contains the terms, “Linear,” “Trigonal planar,” and “Tetrahedral.” The fourth column contains the terms “s p,” “s p superscript 2,” and “s p superscript 3.” The last column contains drawings of the molecules beginning with a peanut-shaped structure marked with an angle of “180 degrees.” The second structure is made up of three equal-sized, rounded structures connected at one point with an angle of “120 degrees,” while the third structure is a three-dimensional arrangement of four equal-sized, rounded structures labeled as “109.5 degrees.”
Figure 15. The shapes of hybridized orbital sets are consistent with the electron-pair geometries. For example, an atom surrounded by three regions of electron density is sp2 hybridized, and the three sp2 orbitals are arranged in a trigonal planar fashion.

It is important to remember that hybridization was devised to rationalize experimentally observed molecular geometries. The model works well for molecules containing small central atoms, in which the valence electron pairs are close together in space. However, for larger central atoms, the valence-shell electron pairs are farther from the nucleus, and there are fewer repulsions. Their compounds exhibit structures that are often not consistent with VSEPR theory, and hybridized orbitals are not necessary to explain the observed data. For example, we have discussed the H–O–H bond angle in H2O, 104.5°, which is more consistent with sp3 hybrid orbitals (109.5°) on the central atom than with 2p orbitals (90°). Sulfur is in the same group as oxygen, and H2S has a similar Lewis structure. However, it has a much smaller bond angle (92.1°), which indicates much less hybridization on sulfur than oxygen. Continuing down the group, tellurium is even larger than sulfur, and for H2Te, the observed bond angle (90°) is consistent with overlap of the 5p orbitals, without invoking hybridization. We invoke hybridization where it is necessary to explain the observed structures.

Three Lewis structures are shown. The left structure shows an oxygen atom with two lone pairs of electrons single bonded to two hydrogen atoms. The middle structure is made up of a sulfur atom with two lone pairs of electrons single bonded to two hydrogen atoms. The right structure is made up of a tellurium atom with two lone pairs of electrons single bonded to two hydrogen atoms. From left to right, the bond angles of each molecule decrease.

Example 1

Assigning Hybridization
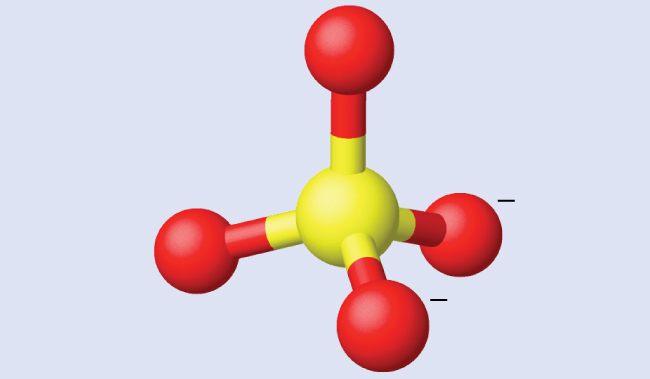
Ammonium sulfate is important as a fertilizer. What is the hybridization of the sulfur atom in the sulfate ion, SO42−?

Solution
The molecular geometry of sulfate shows there are four regions of electron density. The hybridization is sp3.

A structure is shown in which a sulfur atom is bonded to four oxygen atoms in a tetrahedral arrangement. Two of the oxygen atoms have a negative charge.

Check Your Learning
What is the hybridization of the nitrogen atom in H2CNH?

Answer:

The nitrogen atom is sp2 hybridized.

Example 2

Assigning Hybridization
Glycine is shown below. The lone pairs have been omitted. Determine the hybridization for the nitrogen atom, C1, C2, and O1.

A structure is shown with lone pairs omitted. Starting at the left, there is a nitrogen atom connected to two hydrogen atoms. The nitrogen atom is then connected to a carbon atom labeled “C 1”. The C 1 carbon is attached to two hydrogen atoms. The C 1 carbon is then attached to a carbon labeled “C 2”. This C 2 carbon is double bonded to an oxygen atom and single bonded to an oxygen atom labeled “O 1”. The O 1 oxygen atom is bonded to a hydrogen atom.

Solution

First, fill in lone pairs where needed.

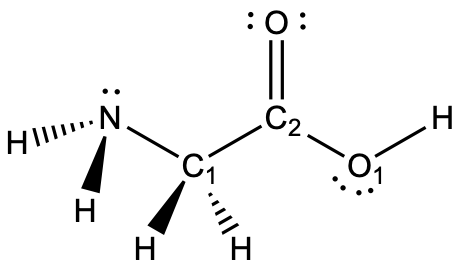
A structure is shown with lone pairs omitted. Starting at the left, there is a nitrogen atom connected to two hydrogen atoms. The nitrogen atom has a lone pair of electrons. The nitrogen atom is then connected to a carbon atom labeled “C 1”. The C 1 carbon is attached to two hydrogen atoms. The C 1 carbon is then attached to a carbon labeled “C 2”. This C 2 carbon is double bonded to an oxygen atom that has 2 lone pairs. The C 2 carbon is single bonded to an oxygen atom labeled “O 1”. The O 1 oxygen atom has 2 lone pairs and is bonded to a hydrogen atom.

The nitrogen atom is surrounded by four regions of electron density, which arrange themselves in a tetrahedral electron-pair geometry. The hybridization in a tetrahedral arrangement is sp3 (Figure 15). Nitrogen is sp3 hybridized.

The C1 carbon atom is surrounded by four regions of electron density, which arrange themselves in a tetrahedral electron-pair geometry. The hybridization in a tetrahedral arrangement is sp3 (Figure 15). C1 is sp3 hybridized.

The C2 carbon atom is surrounded by three regions of electron density, positioned in a trigonal planar arrangement. The hybridization in a trigonal planar electron pair geometry is sp2 (Figure 15), which is the hybridization of C2.

The O1 oxygen atom is surrounded by four regions of electron density, which arrange themselves in a tetrahedral electron-pair geometry. The hybridization in a tetrahedral arrangement is sp3 (Figure 15). O1 is sp3 hybridized.

Check Your Learning
Acetic acid, H3CC(O)OH, is the molecule that gives vinegar its odor and sour taste. What is the hybridization of the two carbon atoms in acetic acid?

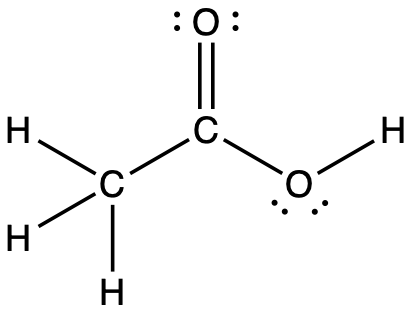
A Lewis structure is shown in which a carbon atom is double bonded to an oxygen atom that has two lone pairs of electrons and single bonded to another oxygen atom that is single boned to a hydrogen atom. This second oxygen atom has two lone pairs of electrons. The carbon is also single bonded to a carbon atom that is single bonded to three hydrogen atoms.

Answer:

H3C, sp3; C(O)OH, sp2

Key Concepts and Summary

We can use hybrid orbitals, which are mathematical combinations of some or all of the valence atomic orbitals, to describe the electron density around covalently bonded atoms. We can determine the type of hybridization around a central atom from the geometry of the regions of electron density about it. Two such regions imply sp hybridization; three, sp2 hybridization; four, sp3 hybridization.

Glossary

hybrid orbital
orbital created by combining atomic orbitals on a central atom
hybridization
model that describes the changes in the atomic orbitals of an atom when it forms a covalent compound
overlap
coexistence of orbitals from two different atoms sharing the same region of space, leading to the formation of a covalent bond
sp hybrid orbital
one of a set of two orbitals with a linear arrangement that results from combining one s and one p orbital
sp2 hybrid orbital
one of a set of three orbitals with a trigonal planar arrangement that results from combining one s and two p orbitals
sp3 hybrid orbital
one of a set of four orbitals with a tetrahedral arrangement that results from combining one s and three p orbitals
valence bond theory
description of bonding that involves atomic orbitals overlapping to form σ or π bonds, within which pairs of electrons are shared

Chemistry End of Section Exercises

  1. Why is the concept of hybridization required in valence bond theory?
  2. Give the shape that describes each hybrid orbital set:
    1. sp2
    2. sp
  3. What is the hybridization of the central atom in each of the following?
    1. BeH2
    2. PO43−
  4. Methionine, CH3SCH2CH2CH(NH2)CO2H, is an amino acid found in proteins. Draw a Lewis structure of this compound. What is the hybridization type of each carbon, nitrogen, and sulfur?
  5. Sulfuric acid is manufactured by a series of reactions represented by the following equations:
    S8(s)   +   8 O2(g)  →   8 SO2(g)
    2 SO2(g)   +   O2(g)  →   2 SO3(g)
    SO3(g)   +   H2O(l)  →   H2SO4(l)

    Draw a Lewis structure, predict the molecular geometry by VSEPR, and determine the hybridization of sulfur for the following:

    1. circular S8 molecule
    2. SO2 molecule
    3. SO3 molecule
    4. H2SO4 molecule (the hydrogen atoms are bonded to oxygen atoms)
  6. For many years after they were discovered, it was believed that the noble gases could not form compounds. Now we know that belief to be incorrect. A mixture of xenon and fluorine gases, confined in a quartz bulb and placed on a windowsill, is found to slowly produce a white solid. Analysis of the compound indicates that it contains 77.55% Xe and 22.45% F by mass.
    1. What is the formula of the compound?
    2. Write a Lewis structure for the compound.
    3. Predict the shape of the molecules of the compound.
  7. Consider nitrous acid, HNO2 (HONO).
    1. Write a Lewis structure.
    2. What are the electron pair and molecular geometries of the internal oxygen and nitrogen atoms in the HNO2 molecule?
    3. What is the hybridization on the internal oxygen and nitrogen atoms in HNO2?
  8. Identify the hybridization of each carbon atom in the following molecule. (The arrangement of atoms is given; you need to determine how many bonds connect each pair of atoms.)
    A Lewis structure is shown that is missing all of its bonds. Six carbon atoms form a chain. There are three hydrogen atoms located around the first carbon, two located around the second, one located near the fifth, and two located around the sixth carbon.
  9. In addition to NF3, two other fluoro derivatives of nitrogen are known: N2F4 and N2F2. What shapes do you predict for these two molecules? What is the hybridization for the nitrogen in each molecule?

Answers to Chemistry End of Section Exercises

  1. Hybridization is introduced to explain the geometry of bonding orbitals in valance bond theory.
  2. (a) trigonal planar; (b) linear
  3. (a) sp; (b) sp3
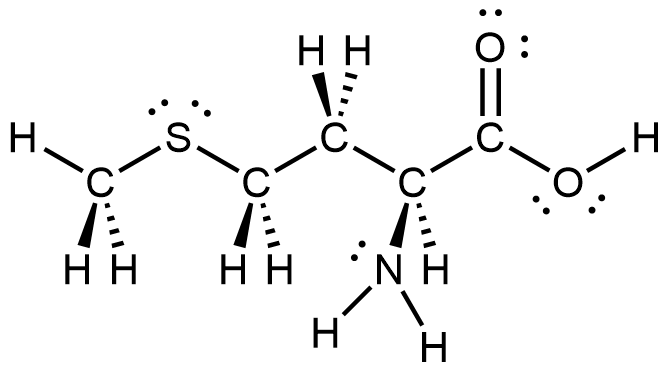  4. ; carbons from left to right: C (sp3), C (sp3), C (sp3), C (sp3), C (sp2). Then S (sp3) and N (sp3).
  5. (a) Each S has a bent (109°) geometry, sp3,  A Lewis structure is shown in which eight sulfur atoms, each with two lone pairs of eletrons, are single bonded together into an eight-sided ring.
    (b) Bent (120°), sp2,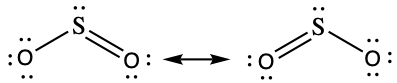 Two Lewis structures are shown with a double-headed arrow in between. The left structure shows a sulfur atom with a lone pair of electrons single bonded to the left to an oxygen atom with three lone pairs of electrons. The sulfur atom is also double bonded on the right to an oxygen atom with two lone pairs of electrons. The right structure depicts the same atoms, but this time the double bond is between the left oxygen and the sulfur atom. The lone pairs of electrons have also shifted to account for the change of bond types. The sulfur atom in the right structures, also has a third electron dot below it.
    (c) Trigonal planar, sp2,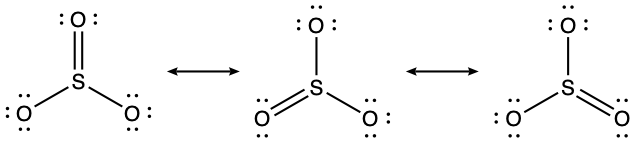
    (d) Tetrahedral, sp3,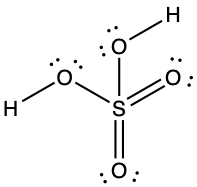 A Lewis structure shows a hydrogen atom single bonded to an oxygen atom with two lone pairs of electrons. The oxygen atom is single bonded to a sulfur atom. The sulfur atom is double bonded to two oxygen atoms, each of which have three lone pairs of electrons, and single bonded to an oxygen atom with two lone pairs of electrons. This oxygen atom is single bonded to a hydrogen atom.
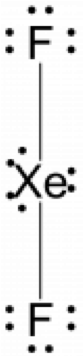  6. (a) XeF2; (b) A Lewis structure is shown in which a xenon atom that has three lone pairs of electrons is single bonded to two fluorine atoms, each of which has three lone pairs of electrons.; (c) linear
  7. (a) 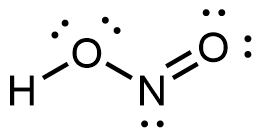; (b) internal O: electron pair geometry = tetrahedral, molecular geometry = bent; N: electron pair geometry = trigonal planar, molecular geometry = bent; (c) internal O: sp3; N: sp2
  8. from left to right: sp3, sp3, sp, sp, sp2, sp2
  9. N2F4: trigonal pyramidal, sp3 N; N2F2: bent, sp2 N
  1. Note that orbitals may sometimes be drawn in an elongated “balloon” shape rather than in a more realistic “plump” shape in order to make the geometry easier to visualize. ↵

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

UW-Madison Chemistry 103/104 Resource Book Copyright © by crlandis is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book