D3.1 Atoms with More than a Single Electron

The ideas already developed about quantum numbers, orbitals, and sizes and shapes of electron-density distributions apply to all atoms. However, when there are two or more electrons in an atom there are repulsive forces between the electrons as well as attractive forces between electrons and the nucleus. These repulsions affect electron energies.

For example, the energy levels in a He⁺ ion (which, like H, has a single electron) are significantly lower than in a H atom because of the stronger Coulomb’s law attraction between the one electron and the 2+ charge of the He nucleus. However, in a He atom, which has two electrons, electron-electron repulsions between the electrons raise energy levels significantly compared to He⁺, and a He atom is not as stable as we might have expected.

For atoms with many electrons, the effect of electron-electron repulsions differs for different subshells. Therefore orbital energy depends on both n and ℓ quantum numbers. For the same value of n (the same shell), as ℓ increases the energy also increases. Thus s-subshell electrons have lower energy than p-subshell electrons, which are lower than d-subshell electrons, and so forth. Orbitals within the same subshell (for example 2p_x, 2p_y, and 2p_z) all have the same energy; orbitals that have the same energy are said to be degenerate.

The Austrian physicist Wolfgang Pauli formulated what is now called the Pauli exclusion principle:

• Each electron in an atom must have a different set of values for the four quantum numbers.
  • If two electrons share the same orbital (have the same n, ℓ, and m_ℓ), then their spin quantum numbers m_s must have different values; we say the two electrons have opposite spin.
• Because m_s can only have two values, +½ or -½, no more than two electrons can occupy the same orbital.

By applying the Pauli exclusion principle, the arrangement of electrons in any multi-electron atom can be determined by recognizing that the ground state of an atom has all its electrons in orbitals with the lowest energies possible.

D3.2 Orbital Energy Level Diagrams

An orbital energy level diagram (or just orbital diagram) shows the relative energies of orbitals and how electrons are distributed among orbitals within a subshell. In an orbital energy level diagram, individual orbitals are usually represented by horizontal lines whose vertical position conveys the relative energies of the orbitals. The electrons are represented as arrows with the direction of the arrow communicating the sign of m_s (the ↑ arrow represents m_s = +½ and the ↓ arrow represents m_s = -½).

For example, a boron atom has this orbital energy level diagram:

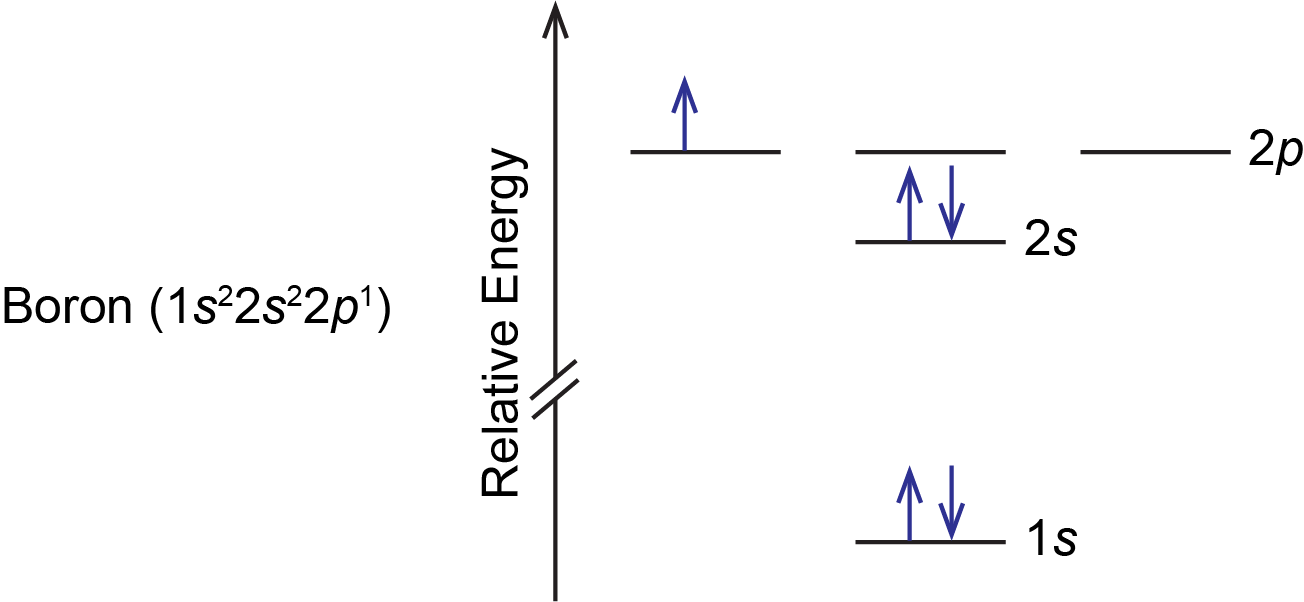
As illustrated by the boron example, an orbital diagram includes all orbitals in all subshells within a partially occupied shell, even if some orbitals are unoccupied. Note that the three 2p orbitals are degenerate, so the electron can occupy any one of them.

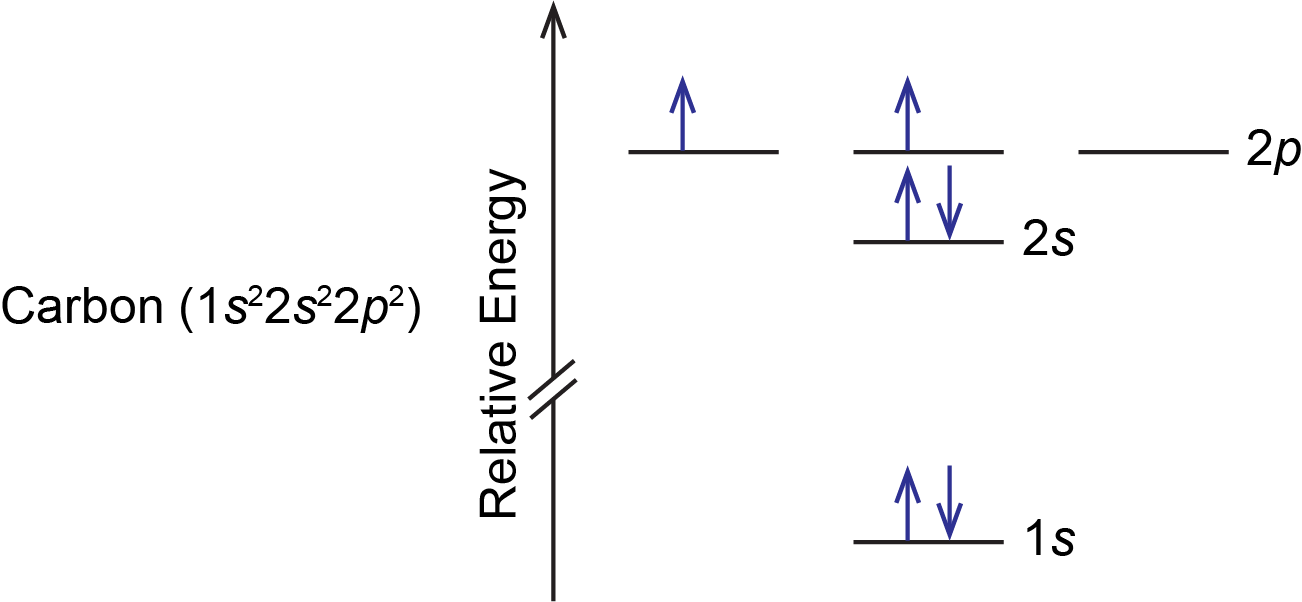
A carbon atom has six electrons, so there are two electrons in the 2p subshell. These two electrons could (1) pair in a single 2p orbital or (2) occupy separate orbitals but with opposite spin or (3) occupy separate orbitals but with parallel spin. All three possibilities are valid based on quantum numbers and the Pauli principle, but only one is lowest in energy. Electrons having parallel spins cannot occupy the same space (the same orbital), so repulsions between them must be smaller than if they had opposite spins. Thus option 3 is lowest in energy and therefore represents the ground state of a carbon atom; options 1 and 2 represent excited states.

According to Hund’s rule, the lowest energy configuration has the maximum number of unpaired electrons with parallel spin within a set of degenerate orbitals. Thus, the orbital diagram for the ground state of carbon is

D3.3 Electron Configurations

The specific arrangement of electrons in atomic orbitals is called the electron configuration of the atom. It determines many physical and chemical properties of that atom. The periodic table, which is arranged in accordance with the properties of the elements, can therefore be used to predict the ground state electron configurations of atoms.

An electron configuration is written symbolically to provide three pieces of information: the principal quantum number (shell number), n; a letter that designates the subshell (s, p, d, etc.); a superscript showing the number of electrons in that particular subshell. For example, the notation 2p⁴ indicates 4 electrons in a p subshell (ℓ = 1) with a principal quantum number (n) of 2.

For any element, the ground state electron configuration can be built up by starting with hydrogen and following the atomic-number order through the periodic table. To go from one element to the next, add one proton (and one or more neutrons) to the nucleus and one electron to the lowest energy subshell that has an incompletely filled orbital. Repeat until you reach the desired element. This process of filling electrons into orbitals is called the aufbau principle, from the German word Aufbauen (“to build up”). Watch the video in Figure 1 to see how to use the aufbau principle to determine the electron configurations of oxygen and chromium.

Writing the complete electron configuration all the time can be cumbersome, so chemists often abbreviate by using the noble-gas notation. For example, the ground-state electron configuration of vanadium (V) is 1s²2s²2p⁶3s²3p⁶4s²3d³. The noble gas that immediately precedes V is argon (Ar); it has a ground-state electron configuration of 1s²2s²2p⁶3s²3p⁶, which can be represented as [Ar]. Thus the ground-state electron configuration of V can be shortened to [Ar]4s²3d³, and it communicates the same information as the complete electron configuration. A list of ground state electron configurations for all elements in the appendix uses noble-gas notation.

The aufbau principle is based on the concept that for ground-state electron configurations, an electron occupies a lower energy atomic orbital rather than occupying a higher energy orbital. Hence, the fact that we observe the 4s orbital fill before the 3d orbitals indicates that the 4s orbital is lower in energy. Similarly, a 6s orbital is lower in energy compared to a 4f orbital.

The energy difference between s, p, d, and f subshells causes orbitals with different n values to have similar energies. In many cases these energies are so similar that there are exceptions to the periodic-table prediction of electron configuration. These exceptions occur for d-block and f-block elements, but not for s-block and p-block elements.

D3.4 Valence Electrons

Valence, the combining power of an atom, was defined near the end of Section D1.4. Ground state electron configurations of atoms provide insights into valence: for example, why does sodium oxide have formula Na₂O but magnesium oxide is MgO?. When two atoms approach and form a chemical bond, the electron density farthest from the nucleus of each atom, in the higher-energy orbitals, interacts with electron density in the other atom. Electrons in lower-energy orbitals, whose electron density is nearer the nucleus, are less important.

Electrons can be separated into two groups: valence electrons occupy the outermost orbitals of an atom; core electrons occupy inner orbitals, with electron density closer to the nucleus. When an electron configuration is written using the noble-gas notation, all electrons represented by the noble-gas symbol in brackets are core electrons. Electrons beyond the noble-gas configuration are valence electrons if they are in the outermost shell of the atom (have the highest n value) or if they are in incompletely filled subshells. For example, consider vanadium, V: [Ar]4s²3d³. There are five valence electrons: two 4s electrons and three 3d electrons. There are 18 core electrons in the 1s, 2s, 2p, 3s, and 3p subshells. The fact that V has five valence electrons results in V forming compounds in which the valence of vanadium ranges from 2 to 5. For example, fluorides of vanadium have formulas VF₂, VF₃, VF₄, and VF₅.

An American chemist, G. N. Lewis, suggested a simple way to keep track of the number of valence electrons: draw dots around the symbol of an element to represent the valence electrons. The element symbol then represents the nucleus and core electrons of an atom. A diagram in which dots represent valence electrons is called a Lewis diagram. Lewis diagrams are most useful for the main-group (representative) elements. Here are Lewis diagrams for atoms of elements in the third row of the periodic table:

When drawing a Lewis diagram dots are added one at a time to each of the four sides of the element symbol. If there are more than four dots to add, dots are paired. Lewis originated the idea that when an atom bonds to another atom the valence electrons rearrange to form an octet, a stable configuration of valence electrons (s²p⁶) that corresponds to each noble gas at the right side of a row in the periodic table. Thus electron configurations and Lewis diagrams for atoms can predict how an atom forms chemical bonds, an idea that we will explore later.

D3.5 Effective Nuclear Charge

Periodic trends in atomic properties can be predicted by applying these ideas about electron-nucleus attraction and electron-electron repulsion:

• Electron-density distributions are in shells that increase in size as the principal quantum number, n, increases. Electrons in larger shells are, on average, farther from the nucleus and less strongly attracted.
• Electrons repel other electrons, raising electrostatic potential energy. This partly counteracts the lowering of energy due to attraction of an electron by the nucleus. Electrons are said screen or shield other electrons from nuclear charge.

The electron density for a core electron (an electron in an inner shell) is, on average, closer to the nucleus than the electron density for a valence electron. Thus, core electrons can significantly counteract the effect of nuclear attraction. Consider a lithium atom (Li, 1s²2s¹), which has three protons in the nucleus. Because the 2s orbital is larger than the 1s orbital, the 1s electron density is mostly located between the nucleus and the 2s electron density. (Move the slider in the middle of Figure 2 to see how much of the 1s electron density lies between the nucleus and 2s electron density.) Thus, the two 1s electrons repel the 2s electron away from the nucleus, counteracting part of the 3+ charge of the nucleus.

To account for such electron-electron repulsions, we use an effective nuclear charge, Z_eff, the positive nuclear charge (given by the atomic number) reduced by the repulsion of a specific electron by all the other electrons. In the case of the Li 2s electron, quantum mechanics calculates that the repulsions from the two 1s electrons reduces the nuclear charge by 1.72; that is, Z_eff for the 2s electron is 3 − 1.72 = 1.28. If all the electron density of the 1s electrons were between the nucleus and the 2s electron, Z_eff would be reduced to 1.

D3.6 Periodic Variation in Atomic Radius

Given that electron density is distributed throughout space but concentrated near the nucleus, it is hard to define the size of an atom. Typically, chemists think of atoms as spheres with radii on the order of tens to hundreds of picometers. One way to determine atomic radii is to measure the distance between atomic nuclei in homonuclear diatomic molecules. (Homonuclear means two atoms of the same element bonded to each other.) The radius of one atom is half the internuclear distance. A second way is to measure the distance between the nuclei of two atoms in a solid metal, where each atom touches several nearest neighbors. Once a set of atomic radii has been determined, these values can be used to estimate the lengths of bonds that have not yet been measured.