Valence bond theory and hybrid orbitals were introduced in Section D9.7. The ideas summarized here will be developed further in today’s work:

• Hybrid orbitals are derived by combining two or more atomic orbitals from the valence shell of a single atom.
  • AOs are the most stable arrangement of electrons in isolated atoms.
  • Hybrid orbitals are important in molecules because they result in stronger σ bonding.
• Most σ bonds are formed from overlaps of hybrid orbitals. Most π bonds are formed from overlap of unhybridized AOs.
• The number of hybrid orbitals equals the number of valence AOs that were combined to produce the hybrid orbitals.
• The type of hybrid orbitals for each bonded atom in a molecule correlates with the local 3D geometry of that atom.
  • The type of hybrid orbitals for each atom can be determined from the Lewis structure (or resonance structures) of a molecule.
  • From the local 3D geometry of each atom, we can obtain the overall 3D geometry of the molecule.

D10.1 Types of Hybrid Orbitals

sp Hybrid Orbitals

Combining the valence s AO with one of the valence p AOs yields two degenerate sp hybrid orbitals, as shown in Figure 1 for the case of 2s and 2p AOs. The two sp hybrid orbitals are oriented at 180° to each other—a linear geometry. After the hybridization, there are two unhybridized 2p AOs left on the atom.

Because these hybrid orbitals are formed from one s AO and one p AO, they have a 1:1 ratio of “s” and “p” characteristics, hence the name “sp“. One of the ways in which the hybrid orbitals exhibit their mixed “s” and “p” characteristics is in their energy. Specifically, the sp hybrid orbitals’ relative energies are about half-way between the 2s and 2p AOs, as illustrated in Figure 1.

The hybridized orbitals are not energetically favorable for an isolated atom. For example, a beryllium atom is lower in energy with its two valence electrons in the 2s AO than if the electrons were in the two sp hybrid orbitals. However, in a covalent molecule, the one large lobe of each sp hybrid orbital gives greater overlap with another orbital from another atom, yielding σ bonds that lower the molecule’s energy.

sp² Hybridization

Combining one valence s AO and two valence p AOs produces three degenerate sp² hybrid orbitals, as shown in Figure 2 for the case of 2s and 2p AOs. The three sp² hybrid orbitals are oriented at 120° with respect to each other and are in the same plane—a trigonal planar (or triangular planar) geometry. Each hybrid orbital is pointed toward a different corner of an equilateral triangle. After hybridization, there is one unhybridized 2p AO left on the atom.

The sp² hybrid orbitals have twice as much “p” character as “s” character; this is indicated by the superscript “2” in sp². Energetically, sp² hybrid orbitals lie closer to the p AO than the s AO, as illustrated in Figure 2 (the sp² hybrid orbitals are higher in energy than the sp hybrid orbitals).

sp³ Hybridization

Combining one valence s AO and all three valence p AOs produces four degenerate sp³ hybridized orbitals, as shown in Figure 4 for the case of 2s and 2p AOs. The four sp³ hybridized orbitals are oriented at 109.5° with respect to each other, each pointing toward a different corner of a tetrahedron—a tetrahedral geometry.

A tetrahedron is a three-dimensional object that has four equilateral triangular faces and four apexes (corners). All four corners are equivalent. See Figure 3 .

An sp³ hybrid orbital has 75% “p” character and 25% “s” character, a 3:1 ratio, hence the superscript “3” in its name. The sp³ hybrid orbitals are higher in energy than the sp² hybrid orbitals, as illustrated in Figure 4.

Formation of a σ bond

When a σ bond forms between two atoms, a hybrid orbital with one unpaired electron from one atom overlaps with a hybrid orbital with one unpaired electron from the other atom. The resulting σ bond is an orbital that contains a pair of electrons (just as a line in a Lewis structure represents two electrons in a σ bond). For example, Figure 5 shows the formation of a C-C σ bond from two sp³ hybridized carbon atoms.

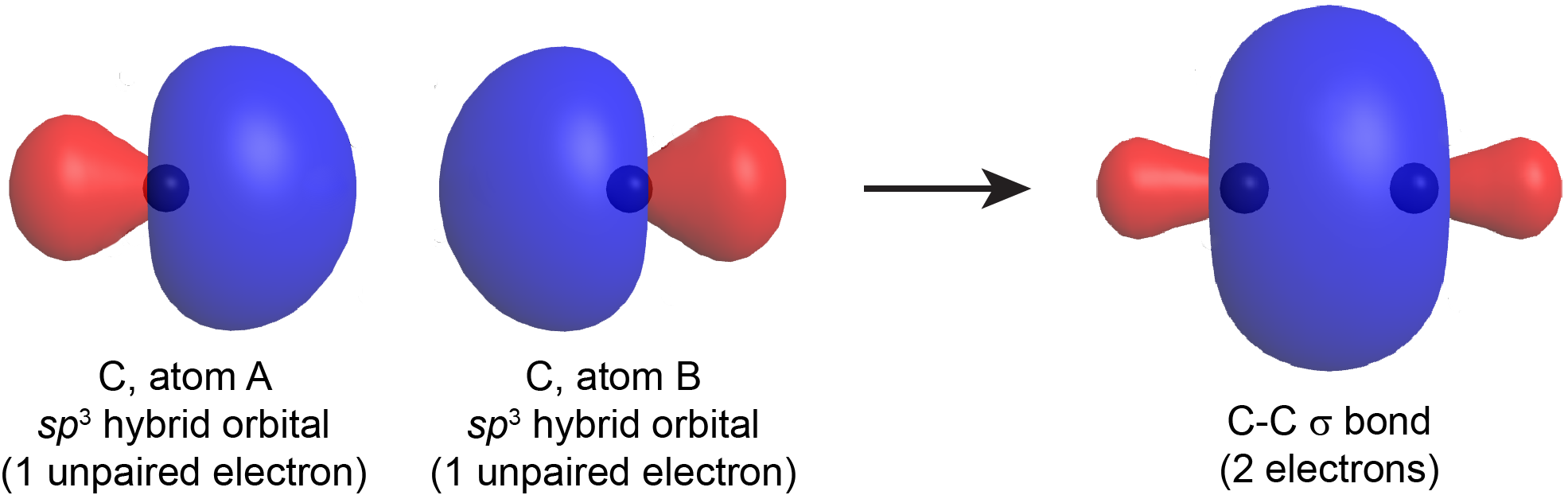
The σ bond thus formed by two hybrid orbitals (valence bond theory) is similar to a σ bond formed in a diatomic molecule as described by MO theory (Section D5.5). In polyatomic molecules with more than three atoms, the MOs are not localized between two atoms like this, but in valence bond theory, the bonds are described individually, between each pair of bonded atoms. Notice that in either MO or valence bond theory, the σ bond has a cylindrical symmetry with respect to the bonding axis.

If a hybridized orbital on an atom in a molecule has two electrons but is not pointing at another atom, the filled hybrid orbital is not involved in bonding. This corresponds to a lone pair on an atom in a Lewis structure.

D10.2 Predicting the Geometry of Bonds Around an Atom

Once you have drawn the best Lewis structure (or a set of resonance structures) for a molecule, you can use the structure(s) to assign hybridization to each atom, predict the geometric arrangement of bonds around each atom, and then predict the 3D structure for the molecule. This and the next few sections explain how this works.

For each atom in a molecule, determine the number of AOs that are hybridized, n_hyb, and use this value to predict hybridization.

• Count the number of σ bonds (n_σ) the atom forms.
  • A double (or triple) bond contains 1 σ bond and 1 (or 2) π bond(s).
• Are there any lone pairs on the atom?
  • If yes: n_hyb = n_σ + 1
  • If no: n_hyb = n_σ
• Is an atom’s n_hyb different in one resonance structure from another?
  • If yes, use the smaller n_hyb to determine hybridization.
• Use the value of n_hyb to determine the number of AOs combined and hence the type of hybridization:
  • For n_hyb = 2, the atom is sp hybridized (two AOs are combined);
  • for n_hyb = 3, the atom is sp² hybridized (three AOs are combined);
  • for n_hyb = 4, the atom is sp³ hybridized (four AOs are combined);
  • An H atom in a molecule has n_hyb = 1. It is not hybridized; its electron is in the 1s AO when forming a σ bond.

These rules derive from the idea that hybridized orbitals form stronger σ bonds. Therefore, the more σ bonds to an atom, the more atomic orbitals are combined to form hybrid orbitals.

D10.3 Three-dimensional Bond Geometry

The two examples so far were a linear (one-dimensional) molecule, BeCl₂, and a planar (two-dimensional) molecule, BF₃. What happens when a molecule is three dimensional?

Wedge-dash Notation

The Lewis structures in the activities above are drawn using wedge and dash notation. Straight lines represent bonds in the plane of the page/screen, solid wedges represent bonds coming toward you out of the plane, and dashed wedges represent bonds going away from you behind the plane.

Examine this 3D model of NH₃ and rotate it until it looks like the Lewis structure drawn in the answer in Activity 4. The NH₃ molecule has trigonal pyramidal geometry because the lone pair on nitrogen occupies one of the corners of a tetrahedron, leaving the three N-H bonds occupying the other three corners; this gives a three-cornered pyramid.

Redraw the Lewis structure you drew for ammonia in Activity 4 using wedge-dash notation. Then, rotate the 3D model until it matches your drawing. If you can find an orientation that matches, your wedge-dash Lewis structure is probably correct; if you cannot find a match, your Lewis structure is probably incorrect.

For simplicity, a wedge-dash Lewis structure draws as many as possible of a molecule’s bonds in a plane. However, as is the case with CH₄ and NH₃, most molecules do not have all bonds in the same plane.

D10.4 Molecules with More Than One Central Atom

Larger molecules have more than one “central” atom with several other atoms bonded to it. The arrangement of bonds for each central atom can be predicted as described in the preceding sections. The way these local structures are oriented with respect to each other influences the overall molecular shape.

Double and Triple Bonds

The Lewis structure of ethene, C₂H₄, shows that each carbon atom is surrounded by one other carbon atom and two hydrogen atoms:

Each carbon atom has n_hyb = 3 and therefore is sp² hybridized. Around each C atom there are three bonds in a plane. Two of the sp² orbitals form two C–H σ bonds and the third sp² orbital forms a C-C σ bond.

The double bond between the two C atoms contains a π bond as well as a σ bond. The π bond results from overlap of the unhybridized 2p AO on each carbon atom. The unhybridized 2p AO is perpendicular to the plane of the sp² hybrid orbitals (Figure 6). Thus when the 2p AOs overlap in a side-by-side fashion to form a π bond, the electron densities in the π bond are above and below the plane of the molecule (the plane containing the σ bonds).

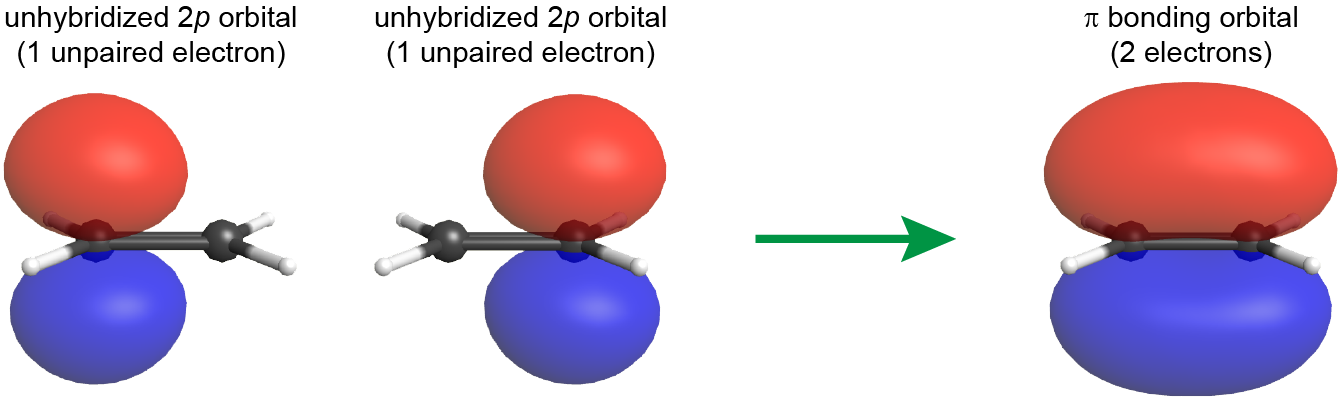
If the plane containing the sp² hybrid orbitals of one carbon atom were rotated 90° relative to the other carbon, the two 2p AOs would also be rotated 90° to each other (Figure 7). The 2p AOs would no longer be able to overlap and the π bond cannot form.

This is a significant difference between σ and π bonds: one atom rotating around the internuclear axis with respect to the other atom does not change the extent to which the σ bonding orbitals overlap because the σ bond is cylindrically symmetric about the bond axis (see Figure 5); in contrast, rotation by 90° about the internuclear axis breaks the π bond entirely because the p orbitals can no longer overlap.

In acetylene, H−C≡C−H, each carbon atom has n_hyb = 2 and therefore is sp hybridized with two unhybridized 2p orbitals. One sp hybrid orbital from each C atom overlaps to form a C-C σ bond, the other sp hybrid orbital forms a C-H σ bond with a hydrogen atom. The unhybridized 2p AOs overlap to form two perpendicular C-C π bonds (Figure 8). The two carbon atoms of acetylene are thus bound together by one σ bond and two π bonds, giving a triple bond.

Because π bonds are formed from unhybridized p AOs, an atom that is involved in π bonding cannot be sp³ hybridized.

D10.5 Hybridization and Bond Angles

Think back to the example molecules CH₄ and NH₃ in Section D9.4. Both involve sp³ hybridized orbitals on the central atom. In the case of CH₄, a 1s orbital on each of the four H atoms overlaps with each of the four sp³ hybrid orbitals to form four bonds. All angles between pairs of C–H bonds are 109.5°. In NH₃ the situation is different in that there are only three H atoms. Three of the four sp³ hybrid orbitals form three bonds to H atoms, but the fourth sp³ hybrid orbital contains the lone pair. The lone pair is different from the H atoms, and this is important.

In earlier sections we described each of a set of four sp³ hybridized orbitals as having ¼ s character and ¾ p character. This is what happens in CH₄. Each of the four C–H bonds involves a hybrid orbital that is ¼ s and ¾ p. Summing over the four bonds gives 4 × ¼ = 1 s orbital and 4 × ¾ = 3 p orbitals—exactly the number and type of AOs from which the hybrid orbitals were formed.

In NH₃, however, three of the four sp³ hybrids form bonds to H atoms and the fourth involves a lone pair. In this and similar situations, the partial s and p characters must still sum to 1 and 3 but each hybrid orbital does not have to be the same as all the others. That is, a hybrid orbital forming an N–H bond could have more p character (and less s character) compared to the hybrid orbital involving the lone pair. The only requirement is that the total s character and the total p character, summed over all four hybrid orbitals, must be one s and three p.

A different ratio of s character and p character gives a different bond angle. For example, in sp² hybridized orbitals (with one-third s character and two-thirds p character) the angle between bonds is 120°, whereas, for sp³ the angle is 109.5°. More p character results in a smaller bond angle. (This makes sense, because for the maximum p character, that is, for two unhybridized p orbitals, the bond angle would be 90° because the p orbitals are at 90°.)

How can you tell how much s character and how much p character is in a specific hybrid orbital? A MO-theory calculation can provide this information, but, for our purposes, a qualitative rule that indicates where there will be more p character is sufficient. Bent’s rule says that a hybrid orbital on a central atom has greater p character the greater the electronegativity of the other atom forming a bond. A lone pair is assigned zero electronegativity because there is no atom attracting electrons in the bond away from the central atom.

Applying Bent’s rule to NH₃, the three bonded H atoms have higher electronegativity than the lone pair (no atom) so we expect more p character in the hybrid orbitals that form the bond pairs. Thus, the angle between any two N–H bonds should be less than the tetrahedral angle. The experimentally measured angle is 106.7°, a bit less than the expected 109.5°.

In the H₂O molecule, two of the O’s sp² hybrid orbitals are involved in forming the O-H σ bonds. One of O lone pairs is in the other sp² hybrid orbital; the other O lone pair is in the unhybridized 2p AO. The overall molecular geometry is bent. If O had perfect sp² hybridization, the H-O-H angle would be 120°, but because the three hybrid orbitals are not equivalent, the angle deviates from ideal. To obtain an accurate bond angle requires an experiment or a high-level MO calculation.

D10.6 Hybridization in Resonance Hybrids

The assignment of hybridization and molecular geometry for molecules that have two or more major resonance structures is similar to the process discussed above, but remember that a set of resonance structures describes a single molecule. Hence the hybridization (and molecular geometry) assigned to one resonance structure must be the same as all other resonance structures in the set. Hence, when assigning hybridization, you should consider all the major resonance structures.

Consider Figure 9:

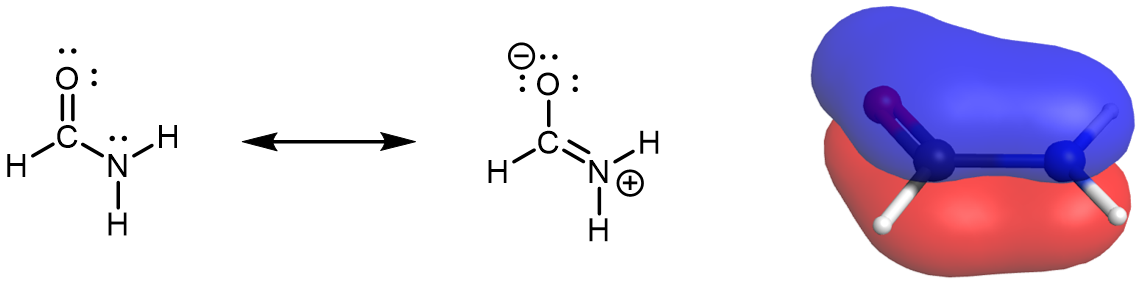
The delocalized π MO extends over the oxygen, carbon, and nitrogen atoms. One of the three AOs contributing to this π MO is an unhybridized 2p AO on the N atom. This is also described by the set of resonance structures, where there is double-bond character between O and C and between C and N. Therefore the nitrogen atom must have sp² hybridization (it forms three σ bonds) and a trigonal planar local geometry. This is more obvious when looking at the right resonance structure. When looking at the left resonance structure, you might be tempted to assign sp³ hybridization to N given its similarity to ammonia (NH₃). However, this is a resonance structure; the set of resonance structures describes a molecule that cannot be described correctly by a single Lewis structure. Hence, the lone pair on N in the left resonance structure is in an unhybridized 2p AO.

All atoms must remain in the same positions from one resonance structure to another in a set of resonance structures. There cannot be a N atom that is trigonal pyramidal in one resonance structure and trigonal planar in another resonance structure, because the atoms attached to the N would have to change positions. Experimental evidence and high-level MO calculations show that formamide is a planar molecule.