Review Section

Review: Waves

Much of modern technology is based on electromagnetic radiation. Radio waves from a mobile phone, X-rays used by dentists, the energy used to cook food in your microwave, the radiant heat from a toaster wire, and the colors emanating from your television screen are forms of electromagnetic radiation. All exhibit behaviors that can be explained by a wave theory.

A wave is an oscillation or periodic movement that can transfer energy from one point to another. For example, the expansion and compression of air that accompanies a lightning strike generates sound waves (thunder) that can travel several miles and cause your ear drums (and sometimes windows) to vibrate. Kinetic energy is transferred through matter (the air) while the matter remains essentially in place. An insightful example of a wave occurs in sports stadiums when fans in a narrow region of seats rise simultaneously and stand with their arms raised for a few seconds before sitting down again and fans in neighboring sections likewise stand up and sit down in sequence. While this wave can quickly encircle a large stadium, none of the fans actually travel with the wave—they all stay in or above their seats.

All waves are characterized by these properties: wavelength ([latex]\lambda[/latex]), the distance between two consecutive peaks, troughs, or other equivalent points in a wave; frequency ([latex]\nu[/latex]), the number of wave cycles (a cycle corresponds to one complete wavelength) that pass a specified point in space in a specified time; and amplitude (A), the magnitude of the wave’s displacement. See examples shown in Figure 1.

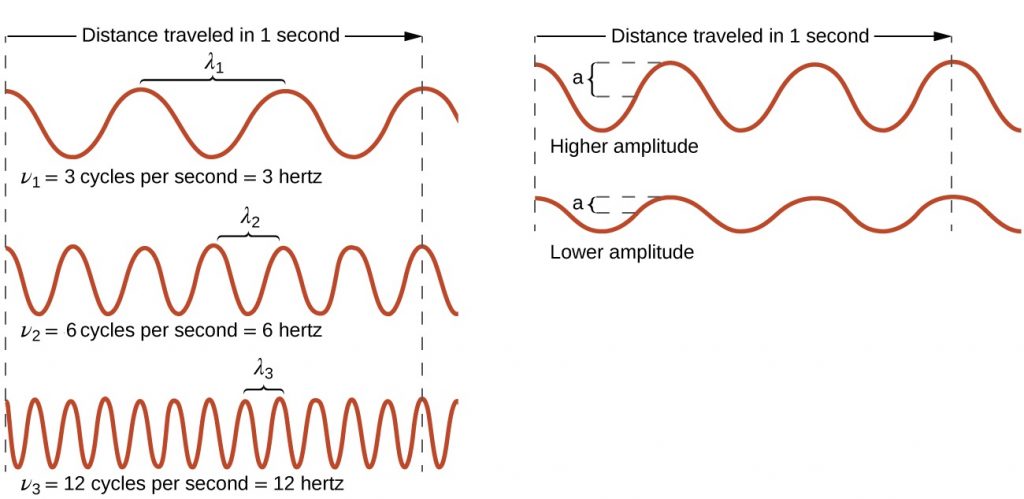
Frequency, wavelength, and amplitude
Figure 1. Waves are characterized by wavelength ($latex\lambda$), frequency ($latex\nu$), and amplitude (A). The product of frequency and wavelength is the distance a wave travels in a given time, i.e., the speed of propagation of the wave.

The SI unit for [latex]\lambda[/latex] is meter, and for [latex]\nu[/latex] is hertz (Hz), which is the number of cycles per second [Hz = [latex]\dfrac{1}{s}[/latex] = s−1]. Common multiples are megahertz, (1 MHz = 1 × 106 Hz) and gigahertz (1 GHz = 1 × 109 Hz). The maximum amplitude of a wave shown in Figure 1 corresponds to one-half the height between the peaks and troughs, and is related to the intensity of the wave. For example, for sound waves, greater amplitude means louder sound.

Waves are not restricted to traveling through matter. James Clerk Maxwell proposed the existence of electromagnetic waves, which consist of an electric field oscillating in the x dimension in step with a magnetic field oscillating in the y dimension. The electromagnetic oscillations travel in the z dimension, where x, y, and z are Cartesian coordinate axes. Electromagnetic radiation travels through a vacuum at a constant speed of 2.998 × 108 m/s, the speed of light (c).

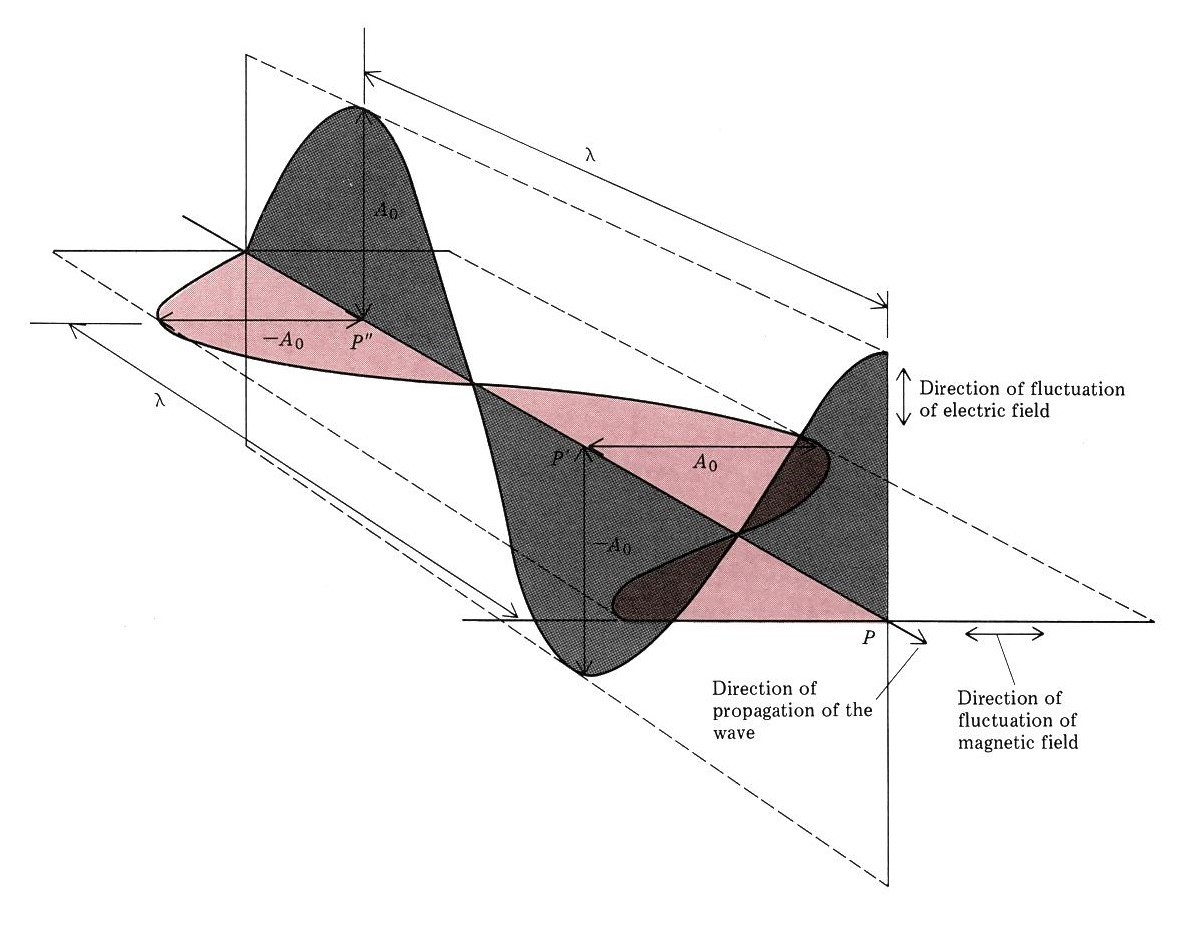
Electromagnetic wave
Figure 2. Electromagnetic radiation involves electric and magnetic fields fluctuating perpendicular to each other. A0 is the maximum amplitude. [latex]\lambda[/latex] is the wavelength.

The product of a wave’s wavelength and its frequency, [latex]\lambda\nu[/latex], is the speed of the wave. (The wave travels distance [latex]\lambda[/latex] per cycle and the number of cycles traveled in a given time is [latex]\nu[/latex].) Thus, for electromagnetic radiation in a vacuum:

[latex]c = 2.998 \times 10^8\;\dfrac{\text{m}}{\text{s}} = \lambda\nu[/latex]

Because the product [latex]\lambda\nu[/latex] is constant, wavelength and frequency are inversely proportional: as the wavelength increases, the frequency decreases.

Exercise 1: Photons and Laser Light

For all exercises, before doing any calculation or looking at the hint, write in your class notebook an explanation of how you plan to work out the problem. Do all the steps in the calculation in your notebook. Once you have arrived at an answer, submit your results below and click the “Check” button to see if it is correct. If one or more parts of your answer is incorrect, go over your work in your notebook carefully and check for errors. “Retry” with your new answer. Look at the hint (click on it to expand for view) only after you have made attempts at answering the question.

Solution: Click the word “solution” to see an explanation of how the result was obtained.

Additional Practice
One of the frequencies used to transmit and receive cellular telephone signals in the United States is 850 MHz. Calculate the wavelength of these radio waves; express your result in meters.

Solution: From here on we assume that you know that clicking the word “solution” shows the result.

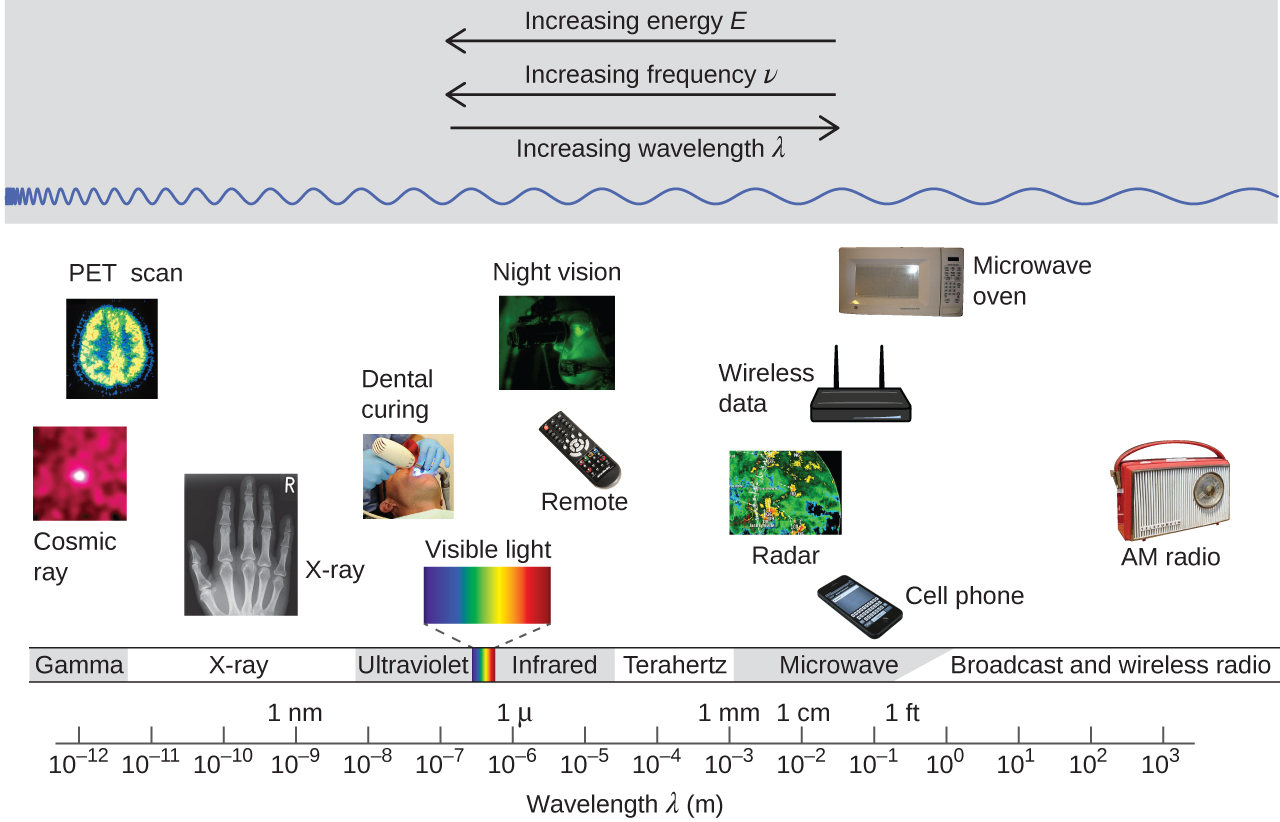
Figure 3 shows the electromagnetic spectrum, the range of all types of electromagnetic radiation. It spans an enormous range—wavelengths of kilometers (103 m) to picometers (10−12 m) have been observed, and the visible-light range makes up only a small portion of it. Given the expansive range of wavelengths, different units are typically used for different parts of the spectrum. For example, radio waves are usually specified as frequencies (typically in units of MHz or GHz), while the visible region is usually specified in wavelengths (typically in units of nm or Å).

The figure includes a portion of the electromagnetic spectrum which extends from gamma radiation at the far left through x-ray, ultraviolet, visible, infrared, terahertz, and microwave to broadcast and wireless radio at the far right. At the top of the figure, inside a grey box, are three arrows. The first points left and is labeled, “Increasing energy E.” A second arrow is placed just below the first which also points left and is labeled, “Increasing frequency nu.” A third arrow is placed just below which points right and is labeled, “Increasing wavelength lambda.” Inside the grey box near the bottom is a blue sinusoidal wave pattern that moves horizontally through the box. At the far left end, the waves are short and tightly packed. They gradually lengthen moving left to right across the figure, resulting in significantly longer waves at the right end of the diagram. Beneath the grey box are a variety of photos aligned above the names of the radiation types and a numerical scale that is labeled, “Wavelength lambda ( m ).” This scale runs from 10 superscript negative 12 meters under gamma radiation increasing by powers of ten to a value of 10 superscript 3 meters at the far right under broadcast and wireless radio. X-ray appears around 10 superscript negative 10 meters, ultraviolet appears in the 10 superscript negative 8 to 10 superscript negative 7 range, visible light appears between 10 superscript negative 7 and 10 superscript negative 6, infrared appears in the 10 superscript negative 6 to 10 superscript negative 5 range, teraherz appears in the 10 superscript negative 4 to 10 superscript negative 3 range, microwave infrared appears in the 10 superscript negative 2 to 10 superscript negative 1 range, and broadcast and wireless radio extend from 10 to 10 superscript 3 meters. Labels above the scale are placed to indicate 1 n m at 10 superscript negative 9 meters, 1 micron at 10 superscript negative 6 meters, 1 millimeter at 10 superscript negative 3 meters, 1 centimeter at 10 superscript negative 2 meters, and 1 foot between 10 superscript negative 1 meter and 10 superscript 0 meters. A variety of images are placed beneath the grey box and above the scale in the figure to provide examples of related applications that use the electromagnetic radiation in the range of the scale beneath each image. The photos on the left above gamma radiation show cosmic rays and a multicolor PET scan image of a brain. A black and white x-ray image of a hand appears above x-rays. An image of a patient undergoing dental work, with a blue light being directed into the patient's mouth is labeled, “dental curing,” and is shown above ultraviolet radiation. Between the ultraviolet and infrared labels is a narrow band of violet, indigo, blue, green, yellow, orange, and red colors in narrow, vertical strips. From this narrow band, two dashed lines extend a short distance above to the left and right of an image of the visible spectrum. The image, which is labeled, “visible light,” is just a broader version of the narrow bands of color in the label area. Above infrared are images of a television remote and a black and green night vision image. At the left end of the microwave region, a satellite radar image is shown. Just right of this and still above the microwave region are images of a cell phone, a wireless router that is labeled, “wireless data,” and a microwave oven. Above broadcast and wireless radio are two images. The left most image is a black and white medical ultrasound image. A wireless AM radio is positioned at the far right in the image, also above broadcast and wireless radio.
Figure 3. Portions of the electromagnetic spectrum are shown. Examples of some applications for various wavelengths include positron emission tomography (PET) scans, X-ray imaging, remote controls, wireless Internet, cellular telephones, and radios. (credit “Cosmic ray”: modification of work by NASA; credit “PET scan”: modification of work by the National Institute of Health; credit “X-ray”: modification of work by Dr. Jochen Lengerke; credit “Dental curing”: modification of work by the Department of the Navy; credit “Night vision”: modification of work by the Department of the Army; credit “Remote”: modification of work by Emilian Robert Vicol; credit “Cell phone”: modification of work by Brett Jordan; credit “Microwave oven”: modification of work by Billy Mabray; credit “Ultrasound”: modification of work by Jane Whitney; credit “AM radio”: modification of work by Dave Clausen)

D1.2 Wave Properties

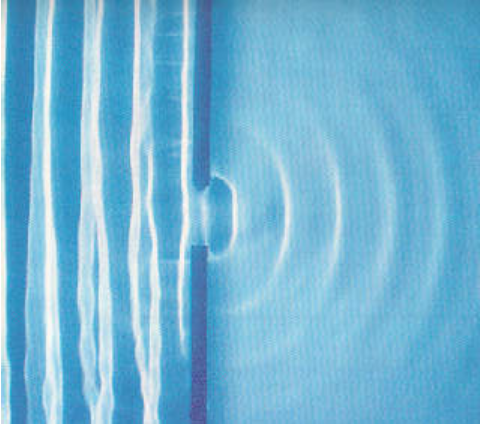When a wave passes through a pinhole or a very narrow slit, waves fan out on other side of the pinhole or slit. This is shown on the right for water waves moving from left to right through a hole about the same width as the wavelength.

When two or more waves occupy the same region, the total wave amplitude is the sum of the amplitudes of the individual waves. Figure 4 shows that this can result in a larger total amplitude if the amplitudes of the waves have the same mathematical sign (are in phase) or in a smaller total amplitude if the two waves have amplitudes with opposite sign (are out of phase).

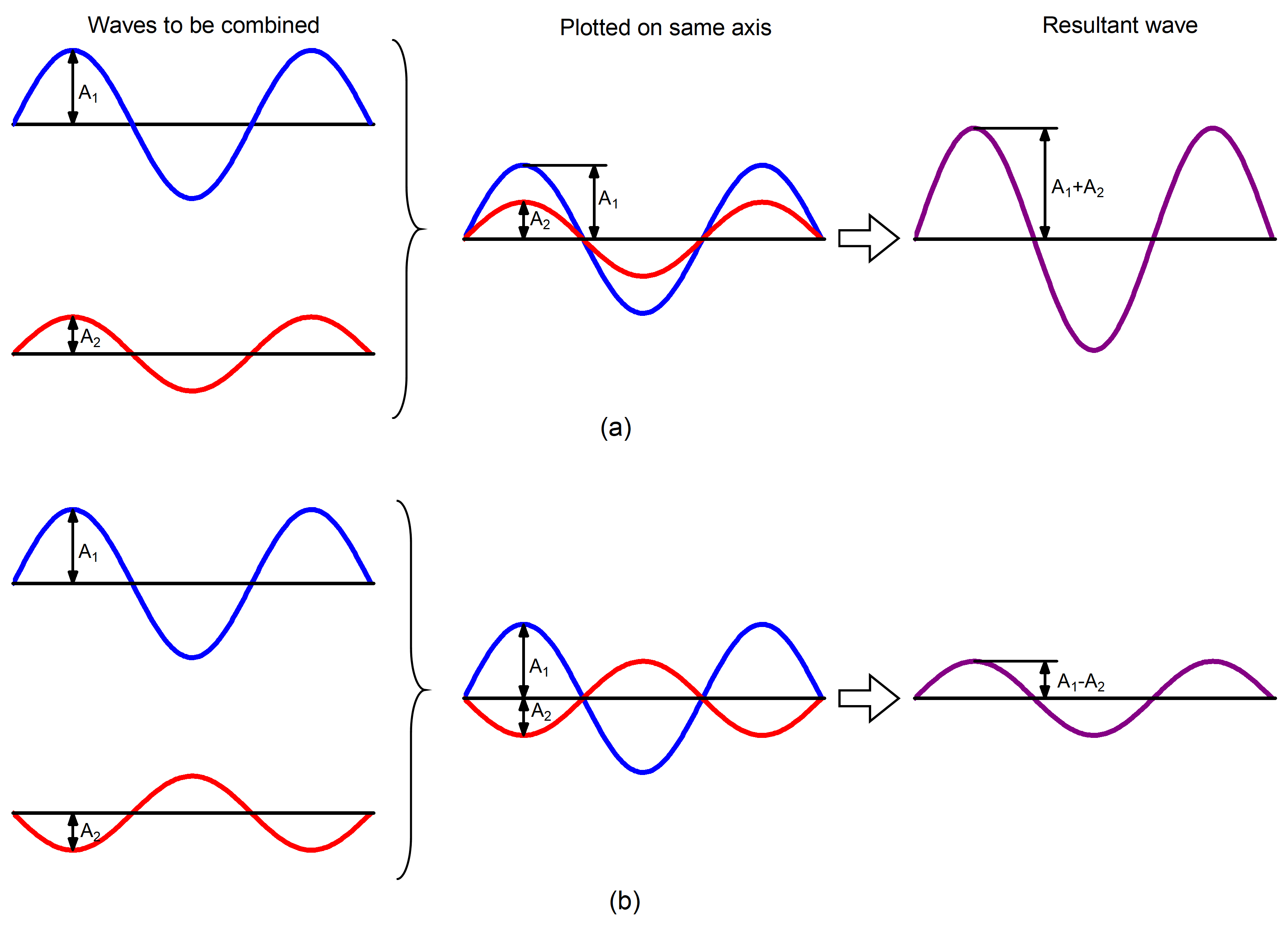
Figure 4. Summation of waves. When two or more waves occupy the same space the resultant amplitude is the sum of the individual amplitudes. This can result in a larger total amplitude (a) or a smaller total amplitude (b). When total amplitude is larger, we say there is constructive interference; when total amplitude is smaller there is destructive interference.

Summation of amplitudes of waves leads to interference of one wave with another. Figure 5 shows the interference patterns that arise when blue or red laser light passes through a diffraction grating with many narrow, vertical slits spaced about one wavelength apart.

Figure 5. Interference patterns. Blue and red laser beams passing through the same diffraction grating produce spots that are more closely spaced for the shorter-wavelength blue light. [Photo by James Maynard]

When laser light passes through closely spaced slits, each slit effectively acts as a new source with waves fanning out from it. This results in closely spaced waves coming into contact at the detector (the camera used to make Figure 5). The dark regions in Figure 5 correspond to regions where the peaks for the wave from one slit happen to coincide with the troughs for the wave from the other slit (destructive interference, Fig. 4b), while the brightest regions correspond to regions where the peaks for the two waves (or their two troughs) happen to coincide (constructive interference, Fig. 4a).

Exercise 2: Interference Patterns

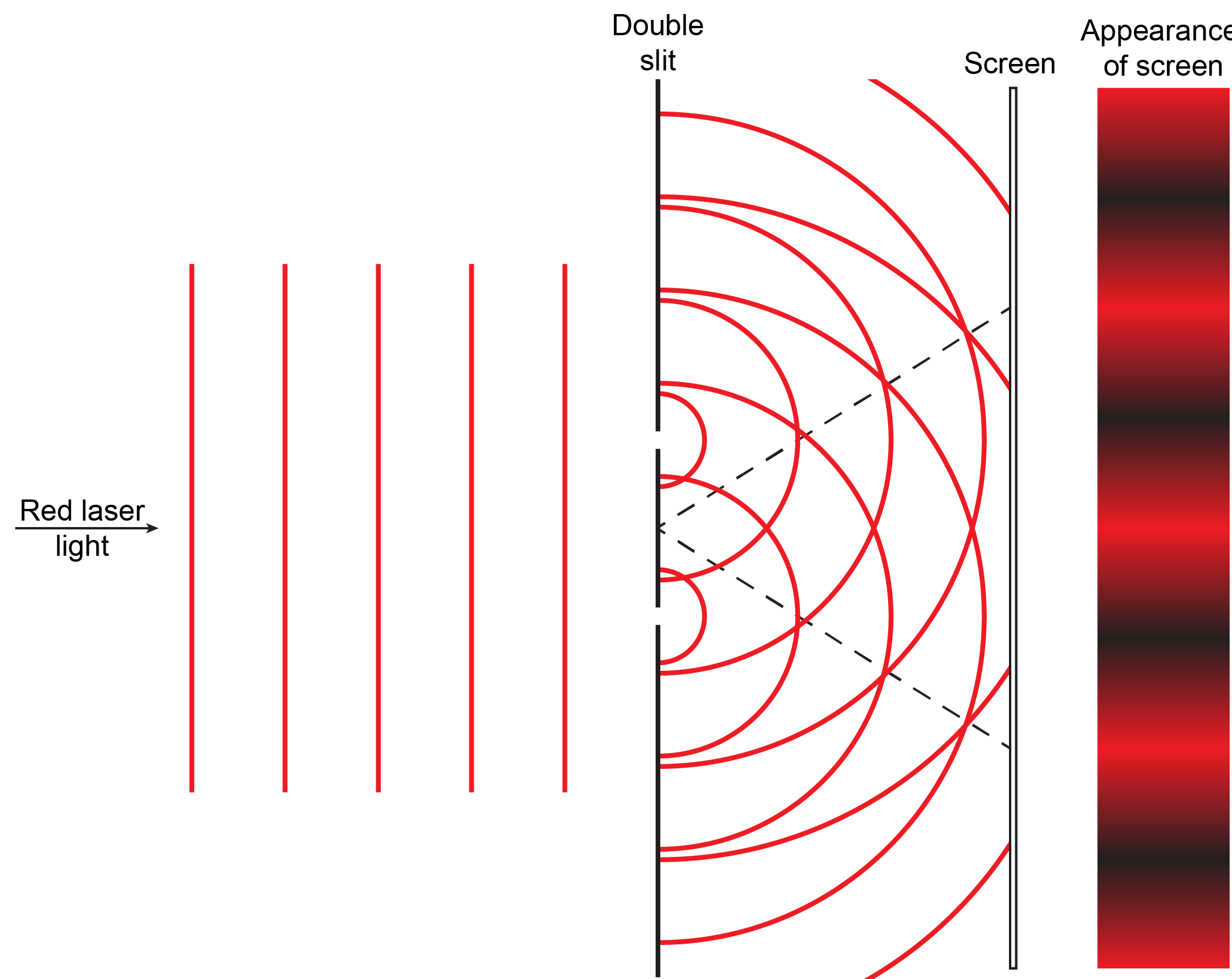
In the diagram below, a red laser light is propagating from left to right. The straight (before slit) and curved (after slit) red lines represent crests of maximum amplitude, and the white spaces halfway between pairs of lines represent troughs of minimum amplitude.

Based on what you have just read about summation of waves and interference patterns, write a paragraph that explains to a fellow student the meaning of the figure and how the figure illustrates the origin of interference patterns. If you are studying with another student each should write an explanation and then try it out on the other. Then compare with the result below and make sure each of you understands the explanations.

Result: Do not click the word “result” until you have written an explanation.

Additional Practice 1
Extend your explanation of the figure to account for the closer spacing in the interference pattern for blue light compared to red light. It might be useful to draw another figure.

Result: Do not click the word “result” until you have written an explanation and perhaps drawn a figure.

Additional Practice 2
Suppose that instead of waves, there is a stream of small particles (say, grains of sand or particles of dust) passing through the pair of slits. Assume that the particles are all traveling in exactly the same direction before they hit the double slit. Draw a diagram like the one above to show the pattern on the screen. Explain in words how and why the particle pattern may differ from the wave pattern.

Result: Do not click the word “result” until you have drawn a pattern and written an explanation.

Interference patterns are an inherent property of wave motion but they cannot be explained by particles moving according to the laws of classical mechanics. Particles would move in straight lines through a slit or pinhole and produce a single bright line or spot.

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chemistry 109, Fall 2020 Copyright © by John Moore; Jia Zhou; and Etienne Garand is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.