Lab 10: BACKGROUND

Enzyme Kinetics

The standard model to describe enzyme kinetics was first put forth by Michealis and Menten to describe initial velocities as a hyperbolic function of enzyme and substrate concentrations (Eqn 1). This is known as the Michealis-Menten equation and describes enzyme catalyzed reactions as shown (Eqn 2).

(1)

(2)

Two important parameters k_cat and K_M are used in the Michealis-Menton equation. The first, k_cat, is the first order rate constant of the conversion from substrate to product. When the enzyme is saturated with substrate, the initial velocity will be directly proportional to the enzyme concentration ([E]) and k_cat. This is a special case and the initial velocity under this condition is called the maximal velocity (V_max) (Eqn 3). This is a theoretical velocity which cost and solubility often prevent from being observed experimentally. Notice, V_max is a function of enzyme concentration, which can make comparing this value across different experiments problematic.

(3)

In contrast, at lower substrate concentrations, the initial velocity cannot be expressed as simply proportional to [E] and k_cat; the Michealis-Menton constant (K_M) must also be included to account for substrate binding. The Michealis-Menton constant is often thought of as the affinity of the substrate for the active site; however, it also includes k_cat so it is not synonymous with the equilibrium dissociation constant (K_d) even though they share the same units (Eqn 4). The Michealis-Menton constant also has a special significance. It is the concentration of substrate at which the initial velocity is half of V_max.

(4)

Another interesting condition to consider is when the substrate concentration is much lower than the Michealis-Menton constant (K_M/[S] > 20). The initial velocity exhibits a second-order relation to the enzyme and substrate concentration, first-order to both enzyme and substrate concentration. The Michealis-Menton can be rewritten in a linear form to describe the region of initial velocity plotted as a function of substrate concentration near the origin (Eqn 5).

(5)

Finally, the value of k_cat/K_M has a special significance in enzymology. It is the second order rate constant (units of M^-1s^-1) to describe enzyme-catalyzed reactions, and is referred to as the catalytic efficiency. Catalytic efficiency is the most commonly used criteria to judge enzymes as catalysts. Enzyme-catalyzed reactions have an inherent speed limit imposed by diffusion in aqueous solutions. The limit of diffusion is ~10^9-10 M^-1s^-1 which catalytic efficiencies of enzymes cannot typically exceed. HCAII is among the most efficient enzymes known and is considered a diffusion-limited catalyst (Khalifah, 1973).

Kinetic Assays for HCAII

While the in vivo function of HCAII is to hydrolyze CO_2, the enzyme also has an esterase function that allows it to catalyze the hydrolysis of p-nitrophenyl acetate (PNPA) to form PNP.

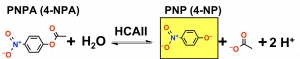
Although the hydrolysis of PNPA is not the biological function of HCAII, the esterase activity is much easier to assay than the hydrolysis activity so this is the assay we will use to study the kinetic parameters of the enzyme. Since the esterase activity utilizes the same active site as the hydrolysis of CO₂, we will use this assay to investigate the effect of the mutations on HCAII. The reaction will be done in protein buffer (PB: 50 mM Tris, pH 8.5, 0.1 M KSO₄) causing the PNP released to adopt its yellow-colored ionized form, which we can measure at 405 nm with the spectrophotometer.

In an aqueous buffer, PNPA will also gradually undergo spontaneous hydrolysis, meaning some yellowing will appear over time even in the absence of any enzymatic reaction. For this reason, it is essential to include a blank reaction. The blank should contain buffer and PNPA at the same concentration that is being tested, but no enzyme. The absorbance at 405 nm of this blank can then be subtracted from the sample to yield the amount of hydrolysis that is due to any enzymatic reaction.

We will conduct a Michaelis-Menten kinetics experiment to compare the esterase activity of mutant HCAII to that of wild type. By using a constant concentration of enzyme and measuring esterase activity across a range of substrate concentrations, you will determine a number of kinetic parameters.

Reference

Khalifah RG. (1973). Carbon dioxide hydration activity of carbonic anhydrase: paradoxical consequences of the unusually rapid catalysis. Proc. Nat. Acad. Sci. USA 70 (7): 1986-1989.