M15Q5: Weak Acid and Weak Base Calculations

Chem 103 Textbook Team; Chem 104 Textbook Team

M15Q5: Weak Acid and Weak Base Calculations

Learning Objectives

Calculate the pH of strong and weak acid and base solutions.
Calculate the equilibrium concentrations of a monoprotic weak acid and its conjugate base, or a weak base and its conjugate acid.
Calculate the percent ionization of a weak acid or base.
Calculate the equilibrium constant for a monoprotic weak acid, K_a, or a weak base, K_b, given pH, [H₃O⁺], or [OH^–].
Apply the relationship between K_a of a monoprotic acid and K_b of its conjugate base to problems as appropriate.

| Key Concepts and Summary | End of Section Exercises |

In this section, we will take what we have learned in the previous few sections and apply this knowledge to a variety of different chemistry problems, all involving weak acids and bases.

Example 1

The Product K_a× K_b = K_w
Given that the K_b for the nitrite ion, NO₂^–, is 2.17 × 10⁻¹¹, calculate the K_a for its conjugate acid.

Solution
The conjugate acid of NO₂^– is HNO₂; K_a for HNO₂ can be calculated using the relationship:

K_a × K_b = 1.0 × 10^-14 = K_w

Solving for K_a, we get:

K_a = $\dfrac{K_{w}}{K_{b}}$ = $\dfrac{1.0 \times 10^{-14}}{2.17 \times 10^{-11}}$ = 4.6 × 10^-4

This answer can be verified by finding the K_a for HNO₂ in Appendix I.

Check Your Learning
We can determine the relative acid strengths of NH₄⁺ and HCN by comparing their ionization constants. The ionization constant of HCN is given in Appendix I as 3.3 × 10⁻¹⁰. The ionization constant of NH₄⁺ is not listed, but the ionization constant of its conjugate base, NH₃, is listed as 1.8 × 10⁻⁵. Determine the ionization constant of NH₄⁺, and decide which is the stronger acid, HCN or NH₄⁺.

Answer:

NH₄⁺ is the slightly stronger acid (K_a for NH₄⁺ = 5.6 × 10⁻¹⁰).

Example 2

Determination of K_a from Equilibrium Concentrations
Acetic acid is the principal ingredient in vinegar (Figure 1); that’s why it tastes sour. At equilibrium, a solution contains [CH₃COOH] = 0.0787 M and [H₃O⁺] = [CH₃COO^–] = 0.00118 M. What is the value of K_a for acetic acid?

An image shows the label of a bottle of distilled white vinegar. The label states that the contents have been reduced with water to 5 percent acidity. — **Figure 1.** Vinegar is a solution of acetic acid, a weak acid. (credit: modification of work by “HomeSpot HQ”/Flickr)

Solution
We are asked to calculate an equilibrium constant from equilibrium concentrations. At equilibrium, the value of the equilibrium constant is equal to the reaction quotient for the reaction:

CH₃COOH(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + CH₃COO^–(aq)

K_a = $\dfrac{[\text{H}_{3}\text{O}^{+}][\text{CH}_{3}\text{COO}^{-}]}{[\text{CH}_{3}\text{COOH}]} = \dfrac{(0.00118)(0.00118)}{0.0787}$ = 1.77 × 10^-5

Check Your Learning
What is the equilibrium constant for the ionization of the HSO₄^– ion, the weak acid used in some household cleansers:

HSO₄^–(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + SO₄^2-(aq)

In one mixture of NaHSO₄ and Na₂SO₄ at equilibrium, [H₃O⁺] = 0.027 M; [HSO₄^–] = 0.29 M; and [SO₄^2-] = 0.13 M.

Answer:

K_a for HSO₄^– = 1.2 × 10^-2

Example 3

Determination of K_b from Equilibrium Concentrations
Caffeine, C₈H₁₀N₄O₂ is a weak base. What is the value of K_b for caffeine if a solution at equilibrium has [C₈H₁₀N₄O₂] = 0.050 M, [C₈H₁₀N₄O₂H⁺] = 5.0 × 10⁻³M, and [OH^–] = 2.5 × 10⁻³M?

Solution
At equilibrium, the value of the equilibrium constant is equal to the reaction quotient for the reaction:

C₈H₁₀N₄O₂(aq) + H₂O(ℓ) ⇌ C₈H₁₀N₄O₂H⁺(aq) + OH^–(aq)

K_b = $\dfrac{[\text{C}_{8}\text{H}_{10}\text{N}_{4}\text{O}_{2}\text{H}^{+}][\text{OH}^{-}]}{[\text{C}_{8}\text{H}_{10}\text{N}_{4}\text{O}_{2}]} = \dfrac{(5.0 \times 10^{-3})(2.5 \times 10^{-3})}{0.050}$ = 2.5 × 10^-4

Check Your Learning
What is the equilibrium constant for the ionization of the HPO₄^2- ion, a weak base:

HPO₄^2-(aq) + H₂O(ℓ) ⇌ H₂PO₄^–(aq) + OH^–(aq)

In a solution containing a mixture of NaH₂PO₄ and Na₂HPO₄ at equilibrium, [OH^–] = 1.3 × 10⁻⁶M; [H₂PO₄^–] = 0.042 M; and [HPO₄^2-] = 0.341 M.

Answer:

K_b for HPO₄^2- = 1.6 × 10^-7

Example 4

Determination of K_a or K_b from pH
The pH of a 0.0516 M solution of nitrous acid, HNO₂, is 2.34. What is its K_a?

HNO₂(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + NO₂^–(aq)

Solution
We determine an equilibrium constant starting with the initial concentrations of HNO₂, H₃O⁺, and NO₂^– as well as one of the final concentrations, the concentration of hydronium ion at equilibrium. (Remember that pH is simply another way to express the concentration of hydronium ion.)

We can solve this problem with the following steps in which x is a change in concentration of a species in the reaction:

pH → [H₃O⁺]_eq → x (in ICE table) → Calculate [HNO₂]_eq and [NO₂^–]_eq → K_a

We can first create an ICE table for this reaction:

HNO₂(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + NO₂^–(aq)

I

0.0516

–

0

C

–x

–

+x

E

0.0516 – x

–

x

Next, we can use the pH to find [H₃O⁺]_eq, which is “x” in our ICE table:

[H₃O⁺]_eq = 10^-pH = 10^-2.34 = 0.0046 M = x

	HNO₂(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + NO₂^–(aq)
I	0.0516	–	0	0
C	-0.0046	–	+0.0046	+0.0046
E	0.0470	–	0.0046	0.0046

Finally, we calculate the value of the equilibrium constant using the data in the table:

K_a = $\dfrac{[\text{H}_{3}\text{O}^{+}][\text{NO}_{2}^{-}]}{[\text{HNO}_{2}]} = \dfrac{(0.0046)(0.0046)}{(0.0470)}$ = 4.5 × 10^-4

Check Your Learning.
The pH of a solution of household ammonia, a 0.950 M solution of NH_3, is 11.612. What is K_b for NH₃.

Answer:

K_b = 1.8 × 10⁻⁵

Example 5

Equilibrium Concentrations in a Solution of a Weak Acid
Formic acid, HCO₂H, is the irritant that causes the body’s reaction to ant stings (Figure 2).

A photograph is shown of a large black ant on the end of a human finger. — **Figure 2.** The pain of an ant’s sting is caused by formic acid. (credit: John Tann)

What is the concentration of hydronium ion and the pH in a 0.534-M solution of formic acid?

HCOOH(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + HCOO^–(aq) K_a = 3.0 × 10^-4

Solution

Determine x and equilibrium concentrations. The equilibrium expression is:
HCOOH(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + HCOO^–(aq)

The concentration of water does not appear in the expression for the equilibrium constant, so we do not need to consider its change in concentration when setting up the ICE table.

The table shows initial concentrations (concentrations before the acid ionizes), changes in concentration, and equilibrium concentrations follows (the data given in the problem appear in color):

HCOOH(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + HCOO^–(aq)

I 0.534 – 0 0

C –x – +x +x

E 0.534 – x – x x
Solve for x and the equilibrium concentrations. At equilibrium:
K_a = 3.0 × 10^-4 = $\dfrac{[\text{H}_{3}\text{O}^{+}][\text{HCOO}^{-}]}{[\text{HCOOH}]} = \dfrac{(x)(x)}{0.534 - x}$

Now solve for x. Because the initial concentration of acid is reasonably large and K_a is very small, we assume that x << 0.534, which permits us to simplify the denominator term as (0.534 − x) = 0.534. This gives:

K_a = 3.0 × 10^-4 = $\dfrac{x^{2}}{0.534}$

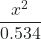
Solve for x as follows:

x² = 0.534 × (3.0 × 10^-4) = 1.6 × 10^-4x = (1.6 × 10^-4)^½ = 1.3 × 10^-2 M

To check the assumption that x is small compared to 0.534, we calculate:

$\dfrac{x}{0.534}$ = $\dfrac{1.3 \times 10^{-2}}{0.534}$ = 2.4 × 10^-2 = 2.4%

x is less than 5% of the initial concentration; the assumption is valid.

We find the equilibrium concentration of hydronium ion in this formic acid solution from its initial concentration and the change in that concentration as indicated in the last line of the table:

[H₃O⁺] = ~0 + x = 0 + 1.3 × 10^-2 M = 1.3 × 10^-2 M

The pH of the solution can be found by taking the negative log of the [H₃O⁺], so:

pH = -log[H₃O⁺] = -log(1.3 × 10^-2) = 1.89

Check Your Learning
Only a small fraction of a weak acid ionizes in aqueous solution. What is the percent ionization of acetic acid in a 0.100-M solution of acetic acid, CH₃COOH?

CH₃COOH(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + CH₃COO^–(aq) K_a = 1.8 x 10^-5

(Hint: Determine [CH₃COO^–] at equilibrium.) Recall that the percent ionization is the fraction of acetic acid that is ionized × 100%, or $\dfrac{[\text{CH}_{3}\text{COO}^{-}]}{[\text{CH}_{3}\text{COOH}]_{initial}}$ × 100%.

Answer:

percent ionization = 1.3%

The following example shows that the concentration of products produced by the ionization of a weak base can be determined by the same series of steps used with a weak acid.

Example 6

Equilibrium Concentrations in a Solution of a Weak Base
Find the concentration of hydroxide ion and the pH in a 0.25 M solution of trimethylamine, a weak base:

(CH₃)₃N(aq) + H₂O(ℓ) ⇌ (CH₃)₃NH⁺(aq) + OH^–(aq) K_b = 6.2 × 10^-5

Solution
This problem requires that we calculate an equilibrium concentration by determining concentration changes as the ionization of a base goes to equilibrium. The solution is approached in the same way as that for the ionization of formic acid in Example 5. The reactants and products will be different and the numbers will be different, but the logic will be the same:

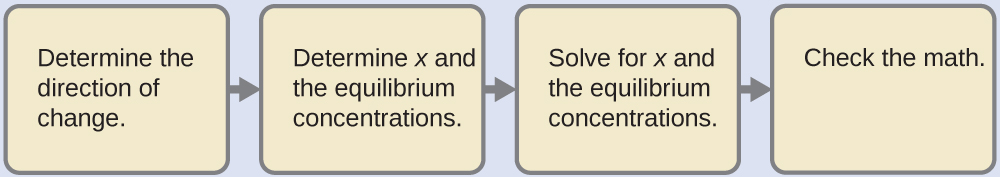
A diagram is shown with 4 tan rectangles connected with right pointing arrows. The first is labeled “Determine the direction of change.” The second is labeled “Determine x and the equilibrium concentrations.” The third is labeled “Solve for x and the equilibrium concentrations.” The final rectangle is labeled “Check the math.”

Determine x and equilibrium concentrations. The table shows the changes and concentrations

(CH₃)₃N(aq) + H₂O(ℓ) ⇌ (CH₃)₃NH⁺(aq) + OH^–(aq)

I 0.25 – 0 0

C –x – +x +x

E 0.25 – x – x x
Solve for x and the equilibrium concentrations. At equilibrium:
K_b = $\dfrac{[(\text{CH}_{3})_{3}\text{NH}^{+}][\text{OH}^{-}]}{[(\text{CH}_{3})_{3}\text{N}]} = \dfrac{(x)(x)}{0.25 - x}$ = 6.2 × 10^-5

If we assume that x is small relative to 0.25, then we can replace (0.25 − x) in the preceding equation with 0.25. Solving the simplified equation gives:

x = 3.9 × 10^-3

This change is less than 5% of the initial concentration (0.25), so the assumption is justified.

Recall that, for this computation, x is equal to the equilibrium concentration of hydroxide ion in the solution (see earlier tabulation):

[OH^–] = ~0 + x = x = 3.9 × 10^-3M

Then calculate pOH as follows:

pOH = -log[OH^–] = -log(3.9 × 10^-3) = 2.40

Using the relation introduced in the previous section of this chapter:

pH + pOH = pK_w = 14.00

permits the computation of pH:

pH = 14.00 – pOH = 14.00 – 2.40 = 11.60
Check the work. A check of our arithmetic shows that K_b = 6.2 × 10⁻⁵.

Check Your Learning
(a) Show that the calculation in Step 2 of this example gives an x of 3.9 × 10⁻³ and the calculation in Step 3 shows K_b = 6.2 × 10⁻⁵.

(b) Find the concentration of hydroxide ion in a 0.0325 M solution of ammonia, a weak base with a K_b of 1.77 × 10⁻⁵. Calculate the percent ionization of ammonia, the fraction ionized × 100%, or $\dfrac{[\text{NH}_{4}^{+}]}{[\text{NH}_{3}]}$ × 100%

Answer:

7.58 × 10⁻⁴M, 2.33%

Some weak acids and weak bases ionize to such an extent that the simplifying assumption that x is small relative to the initial concentration of the acid or base is inappropriate. As we solve for the equilibrium concentrations in such cases, we will see that we cannot neglect the change in the initial concentration of the acid or base, and we must solve the equilibrium equations by using the quadratic equation.

Example 7

Equilibrium Concentrations in a Solution of a Weak Acid
Sodium bisulfate, NaHSO₄, is used in some household cleansers because it contains the HSO₄^– ion, a weak acid. What is the pH of a 0.50 M solution of HSO₄⁻?

HSO₄^–(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + SO₄^2-(aq) K_a = 1.1 × 10^-2

Solution
We need to determine the equilibrium concentration of the hydronium ion that results from the ionization of HSO₄⁻ so that we can use [H₃O⁺] to determine the pH. As in the previous examples, we can approach the solution by the following steps:

Three rectangles are shown with right pointing arrows between them. The first is labeled “Determine x and the equilibrium concentrations.” The second is labeled “Solve for x and the equilibrium concentrations. The third is labeled “Check the math.”

Determine x and equilibrium concentrations. This table shows the changes and concentrations:

HSO₄^–(aq) + H₂O(ℓ) ⇌ H₃O⁺(aq) + SO₄^2-(aq)

I 0.50 – 0 0

C –x – +x +x

E 0.50 – x – x x
Solve for x and the concentrations. As we begin solving for x, we will find this is more complicated than in previous examples. As we discuss these complications we should not lose track of the fact that it is still the purpose of this step to determine the value of x.
At equilibrium:

K_a = 1.1 × 10^-2= $\dfrac{[\text{H}_{3}\text{O}^{+}][\text{SO}_{4}^{2-}]}{[\text{HSO}_{4}^{-}]} = \dfrac{(x)(x)}{0.50 - x}$

If we assume that x is small and approximate (0.50 − x) ≈ 0.50, we find:

1.1 × 10^-2= $\dfrac{(x)(x)}{0.50}$

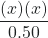
x = 7.4 × 10^-2M

When we check the assumption, we calculate:

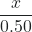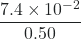$\dfrac{x}{0.50}$ = $\dfrac{7.4 \times 10^{-2}}{0.50}$ = 0.15 = 15%

The value of x is not less than 5% of 0.50, so the assumption is not valid. We need the quadratic formula to find x.

The equation:

K_a = 1.1 × 10^-2= $\dfrac{(x)(x)}{0.50 - x}$

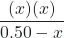
gives

(1.1 × 10^-2)(0.50 – x) = x²x² + (1.1 × 10^-2)x – (5.5 × 10^-3) = 0

This equation can be solved using the quadratic formula. Solving for x gives a negative root (which cannot be correct since concentration cannot be negative) and a positive root:

x = 6.9 × 10^-2

Now determine the hydronium ion concentration and the pH:

[H₃O⁺] = 0 + x = 0 + 6.9 × 10^-2 = 6.9 × 10^-2 M

The pH of this solution is:

pH = -log[H₃O⁺] = -log(6.9 × 10^-2) = 1.16

Check Your Learning
(a) Show that the quadratic formula gives x = 6.9 × 10⁻².

(b) Calculate the pH in a 0.010-M solution of caffeine, a weak base:

C₈H₁₀N₄O₂(aq) + H₂O(ℓ) ⇌ C₈H₁₀N₄O₂⁺(aq) + OH^–(aq) K_b = 2.5 × 10^-4

(Hint: It will be necessary to convert [OH^–] to [H₃O⁺] or pOH to pH toward the end of the calculation.)

Answer:

pH = 11.16

Key Concepts and Summary

In this section, we give many common examples of acid/base quantitative problems. Each example solves for a different variable, some using only the equilibrium expressions (Examples 1-3), but others require you to set up an ICE table during the course of solving the problem (Examples 4-7). We highly recommend you work through these examples and and try the “Check Your Learning” exercise to ensure that you have learned the concept. All of these examples are very common problems you might see on your graded assessments!

Chemistry End of Section Exercises

What is the ionization constant, K_a, at 25 °C for the weak acid CH₃NH₃⁺, given that the K_b of the weak base CH₃NH₂ is 5.0 × 10⁻⁴?
What is the ionization constant of the conjugate base of HNO₂, given that the K_a of HNO₂ is 7.4 × 10^-4?
Describe the method to find the pH of a benzoic acid, C₆H₅COOH, solution with a known concentration. How does this differ from the method to find the pH of an HCl solution of known concentration?
What is the pH of a 0.750 M C₆H₅COOH solution? (K_a = 1.2 × 10^-4)
What is the pH of a 1.25 CH₃NH₂ solution? (K_b = 5.0 × 10^-4)
What is the pH of a 0.0500 M C₆H₅NH₃⁺ solution? (K_b of C₆H₅NH₂ = 3.9 × 10^-10)
Propanoic acid, C₂H₅COOH (K_a = 1.33 × 10⁻⁵), is used in the manufacture of calcium propanoate, a food preservative. What is the hydronium ion concentration in a 0.698 M solution of C₂H₅COOH? What is the pH?
The pH of a 0.20-M solution of a weak acid, HA, is 1.933. Determine K_a for HA from these data.
The pH of a solution of household ammonia, a 0.950 M solution of NH_3, is 11.612. Determine K_b for NH₃ from these data.
The ionization constant of lactic acid, CH₃CH(OH)COOH, an acid found in the blood after strenuous exercise, is 1.36 × 10⁻⁴. If 20.0 g of lactic acid is used to make a solution with a volume of 1.00 L, what is the pH of the solution?
White vinegar is a 5.0% by mass solution of acetic acid, CH₃COOH, in water. If the density of white vinegar is 1.007 g/cm³, what is the pH? The K_a of CH₃COOH is 1.8 × 10^-5.
What is the pH of 0.10 M sodium acetate? (K_a Acetic acid, CH₃COOH = 1.8 × 10^-5)
What is the percent ionization of a 0.20 M HF(aq) solution? (K_a for HF = 7.2 × 10^–4)
The pH of an unknown 0.0100M solution is 3.25. Which of the following could be true about the solution?
1. It is a strong acid.
2. It is a strong base.
3. It is a weak acid.
4. It is a weak base.
5. A K_a value or other information is needed to answer this question.
Chloroacetic acid, CH₂ClCOOH, is a weak acid with K_a = 1.4 × 10^–3. Which of the following describes the relative concentrations of the chemical species found in a 0.10 M aqueous solution of chloroacetic acid?
1. H₂O >> CH₂ClCOOH > H₃O⁺ ≈ CH₂ClCOO^– >> OH^–
2. H₂O >> CH₂ClCOOH > H₃O⁺ ≈ OH^– >> CH₂ClCOO^–
3. H₂O >> CH₂ClCOO^– > H₃O⁺ ≈ OH^– >> CH₂ClCOOH
4. H₂O >> CH₂ClCOO^– ≈ CH₂ClCOOH > H₃O⁺ ≈ OH^–
5. H₂O >> CH₂ClCOO^– ≈ CH₂ClCOOH > H₃O⁺ >> OH^–
Use the table below to answer the following questions.

Name Formula K_a

Acetic acid CH₃COOH 1.8 × 10^–5

Formic acid HCOOH 3.0 × 10^–4

Hydrazoic acid HN₃ 1.0 × 10^–5

Hypochlorous acid HOCl 6.8 × 10^–8
1. Rank the following solutions in order from the lowest to highest pH: 0.10 M CH₃COO^–, 0.10 M HCOO^–, 0.10 M N₃^–, 0.10 M OCl^–.
2. Rank the following solutions in order from the lowest to highest percent ionization: 0.0010 HCOOH, 0.10 M HOCl, 0.0010 M HOCl.

Answers to Chemistry End of Section Exercises

2.0 × 10^-11
The conjugate base is NO₂^–, with K_b = 1.4 × 10^-11.
H₂C₂O₄ is a weak acid and will not completely dissociate in water. The equilibrium H₃O⁺ concentration must be found using an ICE table and the K_a value for the weak acid. Once the equilibrium H₃O⁺ concentration is found, the pH can be determined by calculating -log[H₃O⁺]. HCl is a strong acid and will dissociate completely in water so an ICE table is not necessary to find the H₃O⁺ concentration. It is equal to the original HCl concentration. The pH can be determined by calculating -log [HCl].
pH = 2.0515
pH = 12.40
pH = 2.946
[H₃O⁺] = 0.00305 M; pH = 2.52
[H₃O⁺] = 0.01167 M; K_a = 7.2 × 10^-4
K_b = 1.77 × 10^-5
pH = 2.260
pH = 2.41
pH = 8.87
6.0%
C
A
(a) 0.10 M HCOO^– < 0.10 M CH₃COO^– < 0.10 M N₃^– < 0.10 M OCl^–
(b) 0.10 M HOCl < 0.0010 M HOCl < 0.0010 M HCOOH

Comments
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chem 103/104 Resource Book Copyright © by Chem 103 Textbook Team and Chem 104 Textbook Team is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.