M18Q4: Voltaic Cells and Standard Reduction Potential

Learning Objectives

  • Quantify the electrical energy generated or used by chemical processes through calculations involving units of electric potential (volts) and electric charge (coulombs), as well as Faraday’s constant, F, (the charge of one mole of electrons).
  • Describe the standard hydrogen electrode and explain how it is used as a standard to determine cell potentials of half-reactions.

| Key Concepts and Summary | Key Equations | Glossary |

Electrical Units

Electricity refers to a number of phenomena associated with the presence and flow of electric charge. The flow or movement of charge is an electric current. The SI unit of charge is the coulomb (C) and the charge of an electron is 1.602 × 10−19 C. The presence of an electric charge generates an electric field. Electric current is the rate of flow of charge. The SI unit for electrical current is the SI base unit called the ampere (A), which is a flow rate of 1 coulomb of charge per second (1 A = 1 C/s). An electric current flows in a path, called an electric circuit. In most chemical systems, it is necessary to maintain a closed path for current to flow. The flow of charge is generated by an electrical potential difference, or potential, between two points in the circuit. Electrical potential is the ability of the electric field to do work on the charge. The SI unit of electrical potential is the volt (V). When 1 coulomb of charge moves through a potential difference of 1 volt, it gains or loses 1 joule (J) of energy. Table 1 summarizes some of this information about electricity.

Table 1. Common Electrical Terms
Quantity Definition Measure or Unit
Electric charge Charge on a proton 1.602 × 10−19 C
Electric current The movement of charge ampere = A = 1 C/s
Electric potential The force trying to move the charge volt = V = J/C
Electric field The force acting upon other charges in the vicinity

In a voltaic cell, electrons spontaneously flow from the anode (oxidation reaction) to the cathode (reduction reaction). The electromotive force (EMF) or cell potential (Ecell) in a voltaic cell results from the difference in the electrical potentials for each electrode. It is a relative measurement of the difference between the potential for the reducing agent to be oxidized and the oxidizing agent to be reduced, measured in volts (V). This can be summarized in the following equation:

Ecell = Ecathode – Eanode

Under standard conditions (1 bar for gases; 1 M for solutes, and pure solids), the equation is written as:

E°cell = Eºcathode – Eºanode

Because cell potential is potential energy per unit of charge, it is an intensive property and independent of stoichiometric coefficients

Standard Hydrogen Electrode

Because cell potential is the difference of potential between the two electrodes, it is impossible to determine the electrical potential of a single electrode. In order to obtain potentials for different electrodes, one electrode is assigned the value of zero and then used as a reference to assign other values. The electrode chosen as the zero is shown in Figure 1 and is called the standard hydrogen electrode (SHE). The SHE consists of 1 atm of hydrogen gas bubbled through a 1 M HCl solution, usually at room temperature, with platinum used as the electrode. The reduction half-reaction chosen as the reference is:

2 H+(aq, 1M) + 2 e–  →  H2(g, 1 atm)     E° = 0 V

The H+/H2 half-cell voltage is defined as zero volts for all temperatures.

Assigning the potential of the standard hydrogen electrode (SHE) as zero volts allows the determination of standard reduction potentials, E°, for half-reactions in electrochemical cells. As the name implies, standard reduction potentials use standard states and are written as reduction reactions (where electrons appear on the left side of the equation). The reduction reactions are reversible, so standard cell potentials can be calculated by subtracting the standard reduction potential for the reaction at the anode from the standard reduction for the reaction at the cathode. When calculating the standard cell potential, the standard reduction potentials are not scaled by the stoichiometric coefficients in the balanced overall equation.

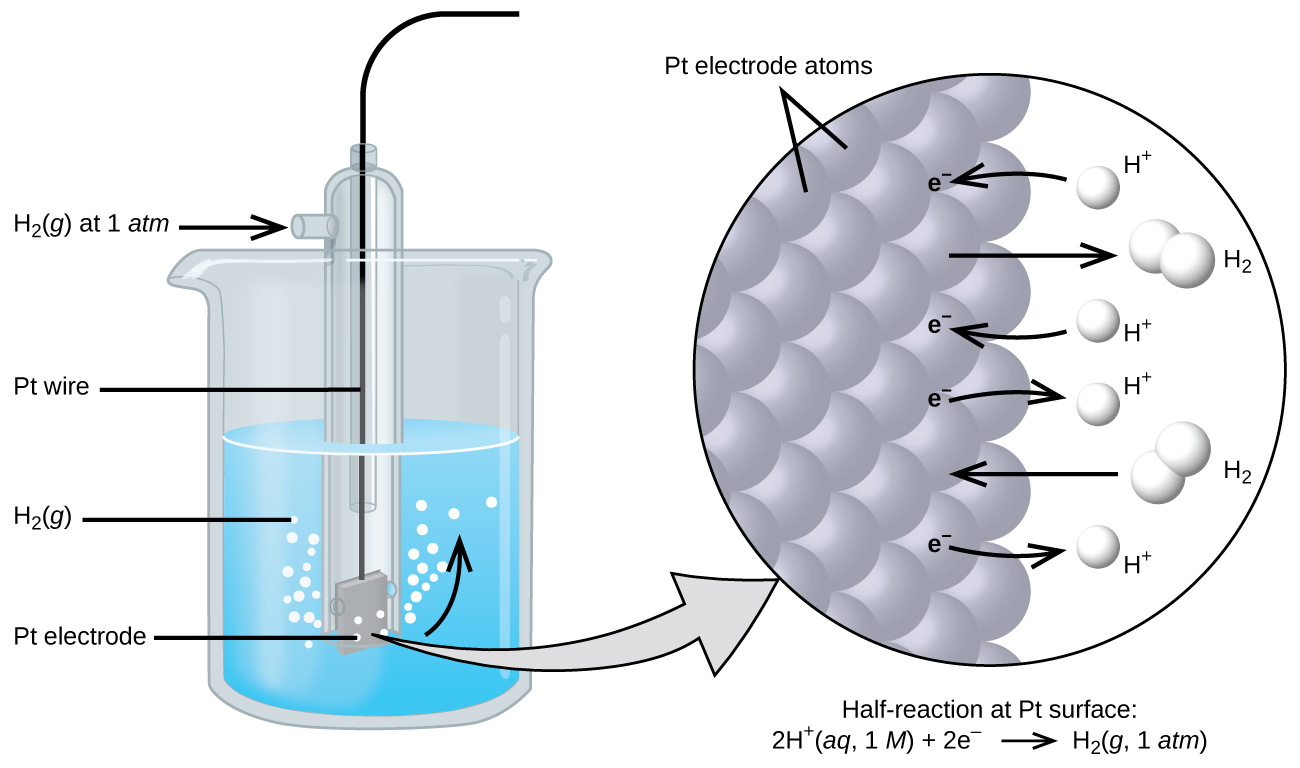
The figure shows a beaker just over half full of a blue liquid. A glass tube is partially submerged in the liquid. Bubbles, which are labeled “H subscript 2 ( g )” are rising from the dark grey square, labeled “P t electrode” at the bottom of the tube. A curved arrow points up to the right, indicating the direction of the bubbles. A black wire which is labeled “P t wire” extends from the dark grey square up the interior of the tube through a small port at the top. A second small port extends out the top of the tube to the left. An arrow points to the port opening from the left. The base of this arrow is labeled “H subscript 2 ( g ) at 1 a t m.” A light grey arrow points to a diagram in a circle at the right that illustrates the surface of the P t electrode in a magnified view. P t atoms are illustrated as a uniform cluster of grey spheres which are labeled “P t electrode atoms.” On the grey atom surface, the label “e superscript negative” is shown 4 times in a nearly even vertical distribution to show electrons on the P t surface. A curved arrow extends from a white sphere labeled “H superscript plus” at the right of the P t atoms to the uppermost electron shown. Just below, a straight arrow extends from the P t surface to the right to a pair of linked white spheres which are labeled “H subscript 2.” A curved arrow extends from a second white sphere labeled “H superscript plus” at the right of the P t atoms to the second electron shown. A curved arrow extends from the third electron on the P t surface to the right to a white sphere labeled “H superscript plus.” Just below, an arrow points left from a pair of linked white spheres which are labeled “H subscript 2” to the P t surface. A curved arrow extends from the fourth electron on the P t surface to the right to a white sphere labeled “H superscript plus.” Beneath this atomic view is the label “Half-reaction at P t surface: 2 H superscript plus ( a q, 1 M ) plus 2 e superscript negative right pointing arrow H subscript 2 ( g, 1 a t m ).”
Figure 1. Hydrogen gas at 1 atm is bubbled through 1 M HCl solution. Platinum, which is inert to the action of the 1 M HCl, is used as the electrode. When reduction occurs, electrons on the surface of the electrode combine with H+ in solution to produce hydrogen gas (top right). When oxidation occurs, H2 donates electrons to the surface of the electrode, leaving H+ in solution (lower right).

Using the Standard Hydrogen Electrode to find the E° for another half-reaction

A voltaic cell consisting of a SHE and Cu2+/Cu half-cell can be used to determine the standard reduction potential for Cu2+ (Figure 2).

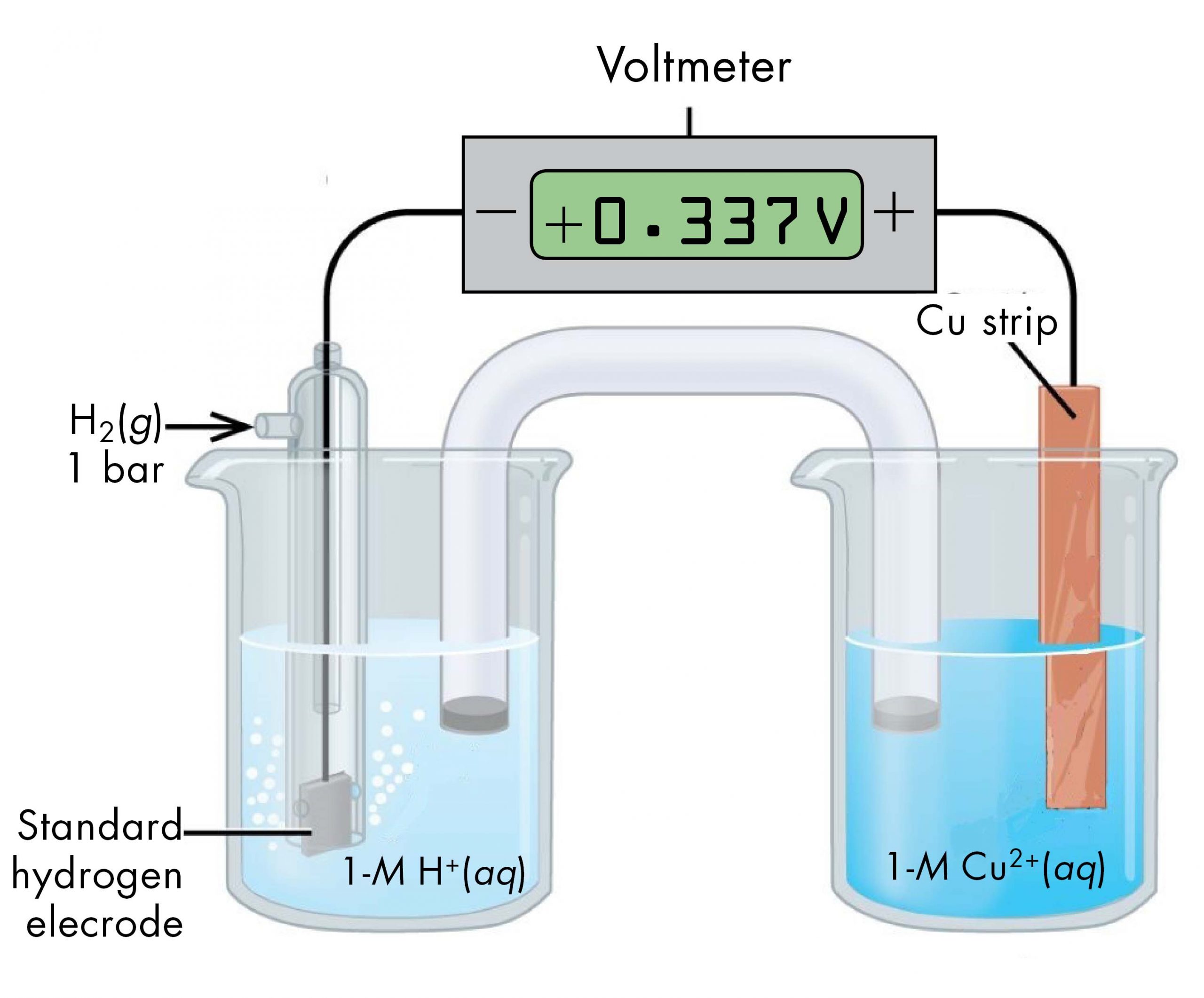
This figure contains a diagram of an electrochemical cell. Two beakers are shown. Each is just over half full. The beaker on the left contains a clear, colorless solution and is labeled below as “1 M H superscript plus.” The beaker on the right contains a blue solution and is labeled below as “1 M C u superscript 2 plus.” A glass tube in the shape of an inverted U, a salt bridge, connects the two beakers at the center of the diagram. The tube contents are colorless. The ends of the tube are beneath the surface of the solutions in the beakers and a small grey plug is present at each end of the tube. The beaker on the left has a glass tube partially submersed in the liquid. Bubbles are rising from a grey square, labeled “Standard hydrogen electrode”, at the bottom of the tube. A black wire extends from the grey square up the interior of the tube through a small port at the top to a rectangle with a digital readout of “positive 0.337 V” which is labeled “Voltmeter.” The wire connects to the negative terminal of the voltmeter. A second small port extends out the top of the tube to the left. An arrow points from the left to the port opening. The base of this arrow is labeled “H subscript 2 ( g ) 1 bar.” The beaker on the right has an orange-brown strip that is labeled “C u strip” at the top. A wire extends from the top of this strip to the voltmeter, connecting to the positive terminal. The solution in the right beaker is labeled “1-M H superscript plus.”
Figure 2. A voltaic cell involving the standard hydrogen electrode can be used to determine the standard half-cell potential of Cu2+(aq, 1 M) | Cu(s).

The standard reduction potential for copper can be determined by subtracting the standard reduction potential for the reaction occurring at the anode from the standard reduction potential for the reaction occurring at the cathode. The SHE is the anode on the “-” lead and copper is the cathode on the “+” lead.

E°cell = Eºcathode – Eºanode

+0.34 V = EºCu2+/Cu – EºH+/H2 = EºCu2+/Cu – 0

EºCu2+/Cu = +0.34 V

Since the measured voltage is positive, the assignments of anode and cathode are correct and electrons flow from the anode to the cathode. The reactions, which are reversible, are:

Anode (Oxidation): H2(g) ⟶ 2 H+(aq) + 2 e–
Cathode (Reduction): Cu2+(aq) + 2 e– ⟶ Cu(s)
Overall: Cu2+(aq) + H2(g) ⟶ 2 H+(aq) + Cu(s)

In line notation, the reaction is:

Pt(s) | H2(g, 1 atm) | H+(aq, 1 M) || Cu2+(aq, 1 M) | Cu(s)

Likewise, a voltaic cell consisting of a SHE and Zn2+/Zn half-cell can be used to determine the standard reduction potential for Zn2+ (Figure 3).

This figure contains a diagram of an electrochemical cell. Two beakers are shown. Each is just over half full. The beaker on the left contains a clear, colorless solution which is labeled “H N O subscript 3 ( a q ).” The beaker on the right contains a clear, colorless solution which is labeled “1 M H superscript plus ( a q ).” A glass tube in the shape of an inverted U salt bridge connects the two beakers at the center of the diagram. The tube contents are colorless. The ends of the tubes are beneath the surface of the solutions in the beakers and a small grey plug is present at each end of the tube. The beaker on the left has a glass tube partially submerged in the liquid. Bubbles are rising from a grey square, labeled “Standard hydrogen electrode” at the bottom of the tube. A black wire extends from the grey square up the interior of the tube through a small port at the top to a rectangle with a digital readout of “negative 0.76 V” which is labeled “Voltmeter.” There is a minus sign where the wire connects to the voltmeter. A second small port extends out the top of the tube to the left. An arrow points to the port opening from the left. The base of this arrow is labeled “H subscript 2 ( g ) 1 bar.” The beaker on the right has a gray strip that is labeled “Z n strip.” A wire extends from the top of this strip to the voltmeter where it connects to the positive terminal. The solution in the beaker on the right has the label “1-M Z n superscript 2 superscript plus ( a q )”
Figure 3. A voltaic cell involving the standard hydrogen electrode can be used to determine the standard half-cell potential of Zn2+(aq, 1 M) | Zn(s).

The standard reduction potential for zinc can be determined by subtracting the standard reduction potential for the reaction occurring at the anode from the standard reduction potential for the reaction occurring at the cathode. The SHE is the anode on the “-” lead and zinc is the cathode on the “+” lead.

E°cell = Eºcathode – Eºanode

-0.76 V = EºZn2+/Zn – EºH+/H2 = EºZn2+/Zn – 0

EºZn2+/Zn = -0.76 V

Since the measured voltage is negative, the leads must be flipped in order to write the spontaneous chemical reaction. Currently, the “-” lead, the anode, is attached to the SHE and the “+” lead, the cathode, is attached to the Zn. The negative voltage implies that for the spontaneous reaction, the anode is actually the Zn and the cathode is actually the SHE.

Key Concepts and Summary

Electricity is associated with the flow of electrons, typically using coulombs. This flow of electric charge creates and electric field and current, which flows on a given path (or circuit). The ability for this electric field to do work is called electric potential (or voltage), which is the basis of voltaic cells. Standard reduction potentials are used in order to predict the voltage that will be created (or if voltage will be created) between two half-cells. All half-cells are connected to a standard hydrogen electrode (SHE). The SHE is given a voltage of 0.00 V in order to be a baseline for all other half-cells. We can use the table of voltages generated through this comparison to calculate the electrical potential difference between any two electrodes using the equation, E°cell = Eºcathode – Eºanode, which always used values directly from the standard reduction potential table.

Key Equations

  • E°cell = Eºcathode – Eºanode

Glossary

cell potential
the voltage created from the difference in the electrical potentials of the anode and cathode
current
the rate of flow of charge, usually in amperes (A), the flow rate of 1 coulomb of charge per second (1 A = 1 C/s)
electric circuit
the path of an electric current
electrical potential
the ability of an electric field to do work on the charge
electromotive force (EMF)
also known as the cell potential
standard hydrogen electrode (SHE)
the electrode chosen as the basis of the standard reduction potential table, which consists of 1 atm of hydrogen gas bubbled through a 1 M HCl solution, with platinum used as the inert electrode. The SHE is given a standard reduction potential of 0.00 V
standard reduction potentials (Eº)
the difference of electrical potential of a reduction reaction when compared to the SHE
Comments
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chem 103/104 Resource Book Copyright © by Chem 103 Textbook Team and Chem 104 Textbook Team is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.