M3Q1: Solutions and Solubility (part 1)

Introduction

This section explores chemical reactions.  First, we examine the conservation of matter.  Second, we examine how chemists use chemical symbols to represent the changes that occur during a reaction. Third, we examine how ionic compounds behave when dissolved in water.  The section below provides a more detailed description of these topics, worked examples, practice problems and a glossary of important terms.

Learning Objectives for Balanced Chemical Equations, including Ionic Salts Dissolved in Water

| Key Concepts and Summary | Glossary | End of Section Exercises |

Conservation of Matter

The law of conservation of matter summarizes many scientific observations about matter: It states that there is no detectable change in the total quantity of matter present when matter converts from one type to another (a chemical change) or changes among solid, liquid, or gaseous states (a physical change). What does this mean for chemistry? In any chemical change, one or more initial substances change into a different substance or substances. Both the initial and final substances are composed of atoms because all matter is composed of atoms. According to the law of conservation of matter, matter is neither created nor destroyed, so we must have the same number and type of atoms after the chemical change as were present before the chemical change.

Although this conservation law holds true for all conversions of matter, convincing examples are few and far between because, outside of the controlled conditions in a laboratory, we seldom collect all of the material that is produced during a particular conversion. For example, when you eat, digest, and assimilate food, all of the matter in the original food is preserved. But because some of the matter is incorporated into your body, and much is excreted as various types of waste, it is challenging to verify by measurement.

When atoms gain or lose electrons to yield ions, or combine with other atoms to form molecules, their symbols are modified or combined to generate chemical formulas that appropriately represent these species. Extending this symbolism to represent both the identities and the relative quantities of substances undergoing a chemical (or physical) change involves writing and balancing a chemical equation. Consider as an example the reaction between one methane molecule (CH4) and two diatomic oxygen molecules (O2) to produce one carbon dioxide molecule (CO2) and two water molecules (H2O). The chemical equation representing this process is provided in the upper half of Figure 1, with space-filling molecular models shown in the lower half of the figure.

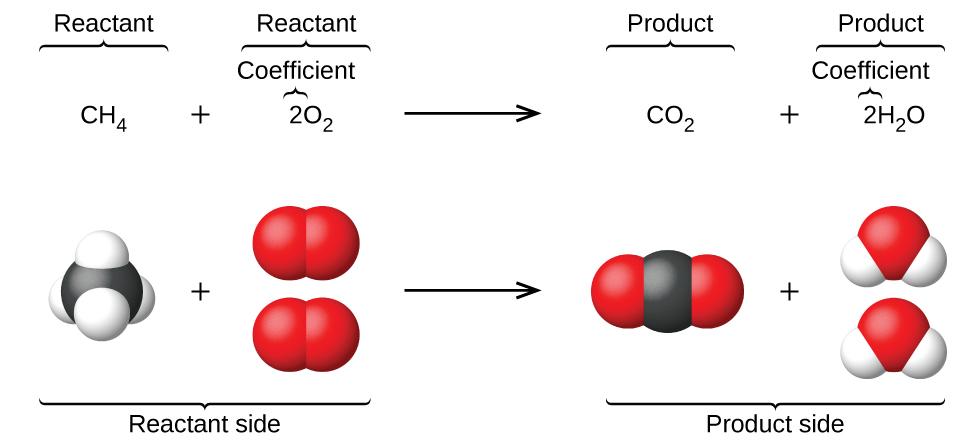
This figure shows a balanced chemical equation followed below by a representation of the equation using space-filling models. The equation reads C H subscript 4 plus 2 O subscript 2 arrow C O subscript 2 plus 2 H subscript 2 O. Under the C H subscript 4, the molecule is shown with a central black sphere, representing a C atom, to which 4 smaller white spheres, representing H atoms, are distributed evenly around. All four H atoms are bonded to the central black C atom. This is followed by a plus sign. Under the 2 O subscript 2, two molecules are shown. The molecules are each composed of two red spheres bonded together. The red spheres represent O atoms. To the right of an arrow and under the C O subscript 2, appears a single molecule with a black central sphere with two red spheres bonded to the left and right. Following a plus sign and under the 2 H subscript 2 O, are two molecules, each with a central red sphere and two smaller white spheres attached to the lower right and lower left sides of the central red sphere. Note that in space filling models of molecules, spheres appear slightly compressed in regions where there is a bond between two atoms.
Figure 1. The reaction between methane and oxygen to yield carbon dioxide and water (shown at bottom) may be represented by a chemical equation using formulas (top).

This example illustrates the fundamental aspects of any chemical equation:

  1. The substances undergoing reaction are called reactants, and their formulas are placed on the left side of the equation.
  2. The substances generated by the reaction are called products, and their formulas are placed on the right side of the equation.
  3. Plus signs (+) separate individual reactant and product formulas, and an arrow (⟶) separates the reactant and product (left and right) sides of the equation.
  4. The relative numbers of reactant and product species are represented by coefficients (numbers placed immediately to the left of each formula). A coefficient of 1 is typically omitted.

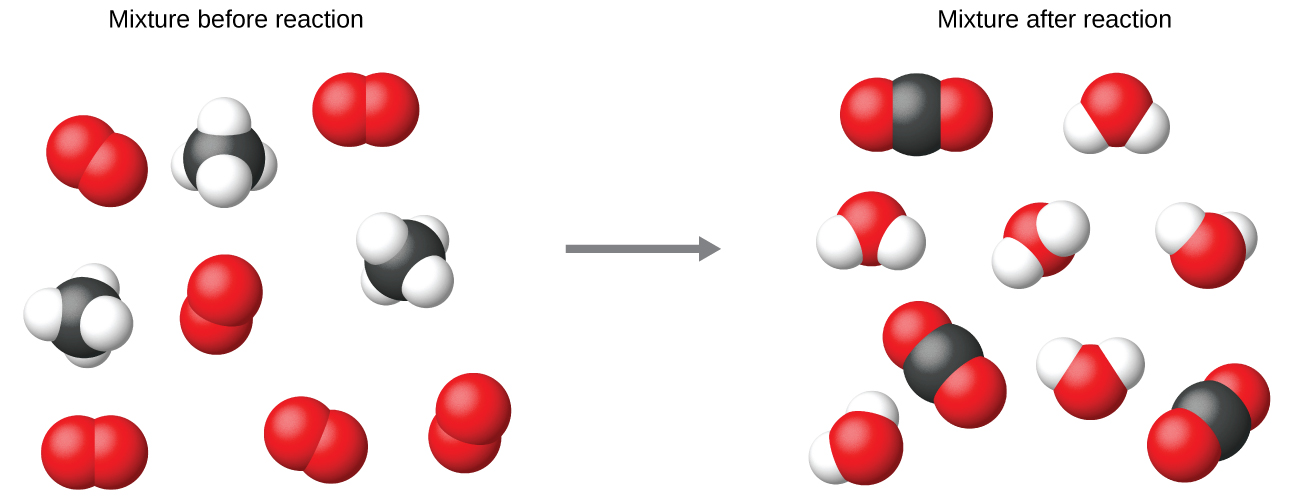
It is common practice to use the smallest possible whole-number coefficients in a chemical equation, as is done in this example. Realize, however, that these coefficients represent the relative numbers of reactants and products, and, therefore, they may be correctly interpreted as ratios. Methane and oxygen react to yield carbon dioxide and water in a 1:2:1:2 ratio. This ratio is satisfied if the numbers of these molecules are, respectively, 1-2-1-2, or 2-4-2-4, or 3-6-3-6, and so on (Figure 2). Likewise, these coefficients may be interpreted with regard to any amount (number) unit, and so this equation may be correctly read in many ways, including:

  • 1 methane molecule and 2 oxygen molecules react to yield 1 carbon dioxide molecule and 2 water molecules.
  • 1 dozen methane molecules and 2 dozen oxygen molecules react to yield 1 dozen carbon dioxide molecules and 2 dozen water molecules.
  • 1 mole of methane molecules and 2 moles of oxygen molecules react to yield 1 mole of carbon dioxide molecules and 2 moles of water molecules. (The mole will be covered in the next module.)
This image has a left side, labeled, “Mixture before reaction” separated by a vertical dashed line from right side labeled, “Mixture after reaction.” On the left side of the figure, two types of molecules are illustrated with space-filling models. Six of the molecules have only two red spheres bonded together. Three of the molecules have four small white spheres evenly distributed about and bonded to a central, larger black sphere. On the right side of the dashed vertical line, two types of molecules which are different from those on the left side are shown. Six of the molecules have a central red sphere to which smaller white spheres are bonded. The white spheres are not opposite each other on the red atoms, giving the molecule a bent shape or appearance. The second molecule type has a central black sphere to which two red spheres are attached on opposite sides, resulting in a linear shape or appearance. Note that in space filling models of molecules, spheres appear slightly compressed in regions where there is a bond between two atoms. On each side of the dashed line, twelve red, three black, and twelve white spheres are present.
Figure 2. Regardless of the absolute numbers of molecules involved, the ratios between numbers of molecules of each species that react (the reactants) and molecules of each species that form (the products) are the same and are given by the chemical reaction equation.

Balancing Equations

The chemical equation described above is a balanced equation, meaning that equal numbers of atoms for each element involved in the reaction are represented on the reactant and product sides. This is a requirement the equation must satisfy to be consistent with the law of conservation of matter. It may be confirmed by simply summing the numbers of atoms on either side of the arrow and comparing these sums to ensure they are equal. Note that the number of atoms for a given element is calculated by multiplying the coefficient of any formula containing that element by the element’s subscript in the formula. If an element appears in more than one formula on a given side of the equation, the number of atoms represented in each must be computed and then added together. For example, both product species in the example reaction, CO2 and H2O, contain the element oxygen, and so the number of oxygen atoms on the product side of the equation is 2 (from CO2) + 2 (from 2 H2O)  =  4 product oxygen atoms.  Thus, we’ll need two O2 molecules as reactants to similarly have 4 reactant oxygen atoms.

The equation for the reaction between methane and oxygen to yield carbon dioxide and water is confirmed to be balanced per this approach, as shown here:

CH4 + 2 O2  ⟶  CO2 + 2 H2O

Element Reactants Products Balanced?
C 1 × 1  =  1 1 × 1  =  1 1  =  1, yes
H 4 × 1  =  4 2 × 2  =  4 4  =  4, yes
O 2 × 2  =  4 (1 × 2) + (2 × 1)  =  4 4  =  4, yes

A balanced chemical equation often may be derived from a qualitative description of some chemical reaction by a fairly simple approach known as balancing by inspection. Consider as an example the decomposition of water to yield molecular hydrogen and oxygen. This process is represented qualitatively by an unbalanced chemical equation:

H2O  ⟶  O2 + H2

Comparing the number of H and O atoms on either side of this equation confirms its imbalance:

Element Reactants Products Balanced?
H 1 × 2  =  2 1 × 2  =  2 2  =  2, yes
O 1 × 1  =  1 1 × 2  =  2 1  ≠  2, no

The numbers of H atoms on the reactant and product sides of the equation are equal, but the numbers of O atoms are not. To achieve balance, the coefficients of the equation may be changed as needed. Keep in mind, of course, that the formula subscripts define, in part, the identity of the substance, and so these cannot be changed without altering the qualitative meaning of the equation. For example, changing the reactant formula from H2O to H2O2 would yield balance in the number of atoms, but doing so also changes the reactant’s identity (it’s now hydrogen peroxide and not water). The O atom balance may be achieved by changing the coefficient for H2O to 2.

2 H2O  ⟶  O2 + H2

Element Reactants Products Balanced?
H 2 × 2  =  4 1 × 2  =  2 4  ≠  2, no
O 2 × 1  =  2 1 × 2  =  2 2  =  2, yes

The H atom balance was upset by this change, but it is easily reestablished by changing the coefficient for the H2 product to 2.

Element Reactants Products Balanced?
H 2 × 2  =  4 2 × 2  =  4 4  =  4, yes
O 2 × 1  =  2 1 × 2  =  2 2  =  2, yes

These coefficients yield equal numbers of both H and O atoms on the reactant and product sides, and the balanced equation is, therefore:

2 H2O  ⟶  O2 + 2 H2

Notice that in all these equations, the charge is also balanced on both the reactant and product sides. In the previous example, there is a total of zero charge on both sides of the equation since H2O, O2, and H2 are all neutral molecules. This will become important later when we start writing equations with ions—the total charge on the reactant side must be equal to the total charge on the product side since electrons cannot be lost in a chemical equation.

Example 1

Balancing Chemical Equations
Write a balanced equation for the reaction of molecular nitrogen (N2) and oxygen (O2) to form dinitrogen pentoxide.

Solution
First, write the unbalanced equation.

N2 + O2  ⟶  N2O5

Next, count the number of each type of atom present in the unbalanced equation.

Element Reactants Products Balanced?
N 1 × 2  =  2 1 × 2  =  2 2  =  2, yes
O 1 × 2  =  2 1 × 5  =  5 2  ≠  5, no

Though nitrogen is balanced, changes in coefficients are needed to balance the number of oxygen atoms. To balance the number of oxygen atoms, a reasonable first attempt would be to change the coefficients for the O2 and N2O5 to integers that will yield 10 O atoms (the least common multiple for the O atom subscripts in these two formulas).

N2 + 5 O2  ⟶  2 N2O5

Element Reactants Products Balanced?
N 1 × 2  =  2 2 × 2  =  4 2  ≠  4, no
O 5 × 2  =  10 2 × 5  =  10 10  =  10, yes

The N atom balance has been upset by this change; it is restored by changing the coefficient for the reactant N2 to 2.

2 N2 + 5 O2  ⟶  2 N2O5

Element Reactants Products Balanced?
N 2 × 2 = 4 2 × 2 = 4 4 = 4, yes
O 5 × 2 = 10 2 × 5 = 10 10 = 10, yes

The numbers of N and O atoms on either side of the equation are now equal, and so the equation is balanced.

Check Your Learning
Write a balanced equation for the decomposition of ammonium nitrate to form molecular nitrogen, molecular oxygen, and water. (Hint: Balance oxygen last, since it is present in more than one molecule on the right side of the equation.)

Answer:

2 NH4NO3  ⟶  2 N2 + O2 + 4 H2O

It is sometimes convenient to use fractions instead of integers as intermediate coefficients in the process of balancing a chemical equation. When balance is achieved, all the equation’s coefficients may then be multiplied by a whole number to convert the fractional coefficients to integers without upsetting the atom balance. For example, consider the reaction of ethane (C2H6) with oxygen to yield H2O and CO2, represented by the unbalanced equation:

C2H6 + O2  ⟶  CO2 + H2O

Following the usual inspection approach, one might first balance C and H atoms by changing the coefficients for the two product species, as shown:

C2H6 + O2  ⟶  2 CO2 + 3 H2O

This results in seven O atoms on the product side of the equation, an odd number—no integer coefficient can be used with the O2 reactant to yield an odd number, so a fractional coefficient, 7/2, is used instead to yield a provisional balanced equation:

C2H6 + \frac{7}{2}O2  ⟶  2 CO2 + 3 H2O

A conventional balanced equation with integer-only coefficients is derived by multiplying each coefficient by 2:

2 C2H6 + 7 O2  ⟶  4 CO2 + 6 H2O

Finally with regard to balanced equations, recall that convention dictates use of the smallest whole-number coefficients. Although the equation for the reaction between molecular nitrogen and molecular hydrogen to produce ammonia is, indeed, balanced,

3 N2 + 9 H2  ⟶  6 NH3

the coefficients are not the smallest possible integers representing the relative numbers of reactant and product molecules. Dividing each coefficient by the greatest common factor, 3, gives the preferred equation:

N2 + 3 H2  ⟶  2 NH3

 

Use this interactive tutorial for additional practice balancing equations.

Additional Information in Chemical Equations

The physical states of reactants and products in chemical equations very often are indicated with a parenthetical abbreviation following the formulas. Common abbreviations include s for solids, l for liquids, g for gases, and aq for substances dissolved in water (aqueous solutions are solutions where water is the solvent, and will be covered later in this section). These notations are illustrated in the example equation here:

2 Na(s) + 2 H2O(ℓ)  ⟶  2 NaOH(aq) + H2(g)

This equation represents the reaction that takes place when sodium metal is placed in water. The solid sodium reacts with liquid water to produce molecular hydrogen gas and the ionic compound sodium hydroxide (a solid in pure form, but readily dissolved in water).

Introduction to Solution Chemistry

Solutions are defined as homogeneous mixtures of two or more substances. Often, one component of a solution is present at a significantly greater concentration, in which case it is called the solvent. The other components of the solution present in relatively lesser concentrations are called solutes. Sugar is a covalent solid composed of sucrose molecules, C12H22O11. When this compound dissolves in water, its molecules become uniformly distributed among the molecules of water:

C12H22O11(s)  ⟶  C12H22O11(aq)

The parenthetical “aq” in the equation signifies that the sucrose molecules are solutes and are therefore individually dispersed throughout the aqueous solution (water is the solvent). Although sucrose molecules are heavier than water molecules, they remain dispersed throughout the solution; gravity does not cause them to “settle out” over time.

Potassium dichromate, K2Cr2O7, is an ionic compound composed of colorless potassium ions, K+, and orange dichromate ions, Cr2O72-.  When a small amount of solid potassium dichromate is added to water, the compound dissolves and dissociates to yield potassium ions and dichromate ions uniformly distributed throughout the mixture (Figure 3), as indicated in this equation:

K2Cr2O7(s)  ⟶  2 K+(aq) + Cr2O72-(aq)

As with the mixture of sugar and water, this potassium dichromate mixture is also an aqueous solution. Its solutes, potassium and dichromate ions, remain individually dispersed among the solvent (water) molecules.

The first photo shows a small mound of an orange crystalline solid. There is a right-facing arrow. The second photo shows a translucent, colorless liquid in a clear container. There is a right-facing arrow. The third photo shows a translucent orange liquid in a clear, covered container.
Figure 3. When potassium dichromate (K2Cr2O7) is mixed with water, it forms a homogeneous orange solution. (credit: modification of work by Mark Ott)

Water is used so often as a solvent that the word solution has come to imply an aqueous solution to many people. However, almost any gas, liquid, or solid can act as a solvent.

Solutions exhibit these defining traits:

  • They are homogeneous; that is, after a solution is mixed, it has the same composition at all points throughout (its composition is uniform).
  • The components of a solution are dispersed on a molecular scale; that is, they consist of a mixture of separated molecules, atoms, and/or ions.
  • The dissolved solute in a solution will not settle out or separate from the solvent.

Dissolving and Electrolytes

When ionic compounds dissolve in water, the ions in the solid separate and disperse uniformly throughout the solution because water molecules surround and solvate the ions, reducing the strong electrostatic forces between them. Water and other polar molecules are attracted to ions, as shown in Figure 4. The electrostatic attraction between an ion and a molecule with a dipole is called an ion-dipole attraction (we will examine these interactions more fully later in the semester). These attractions play an important role in the dissolution of ionic compounds in water.

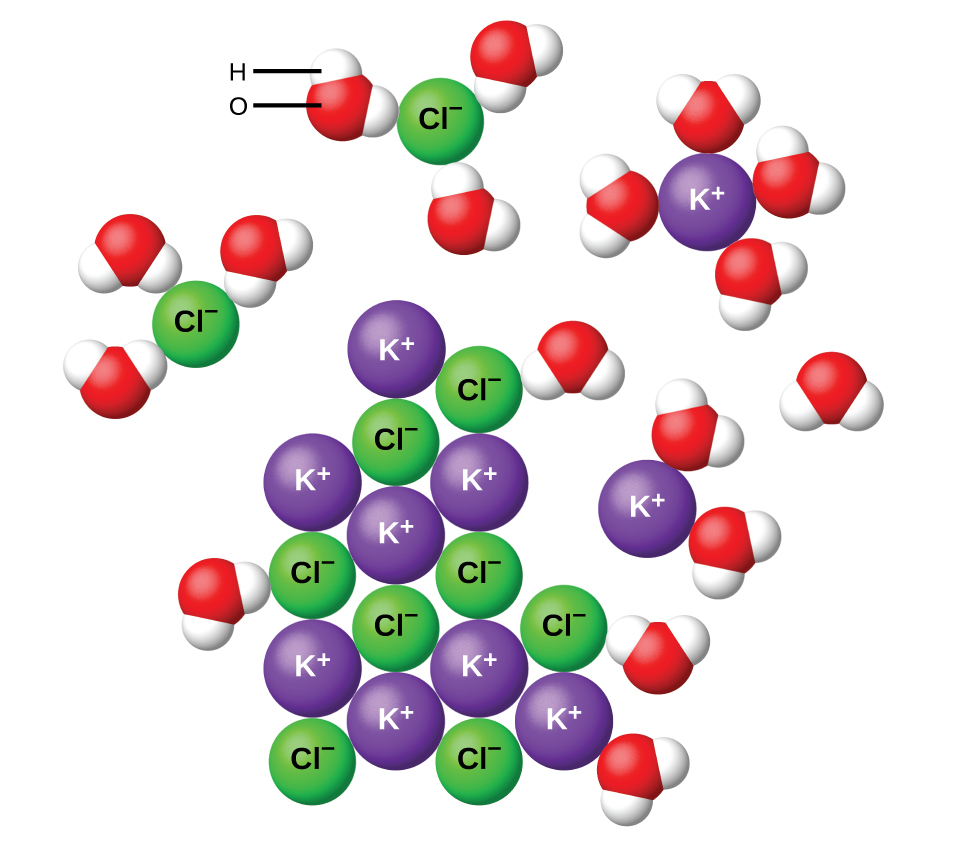
The diagram shows eight purple spheres labeled K superscript plus and eight green spheres labeled C l superscript minus mixed and touching near the center of the diagram. Outside of this cluster of spheres are seventeen clusters of three spheres, which include one red and two white spheres. A red sphere in one of these clusters is labeled O. A white sphere is labeled H. Two of the green C l superscript minus spheres are surrounded by three of the red and white clusters, with the red spheres closer to the green spheres than the white spheres. One of the K superscript plus purple spheres is surrounded by four of the red and white clusters. The white spheres of these clusters are closest to the purple spheres.
Figure 4. As potassium chloride (KCl) dissolves in water, the ions are hydrated. The polar water molecules are attracted by the charges on the K+ and Cl− ions. Water molecules in front of and behind the ions are not shown.

Substances that produce ions in solutions when they dissolve constitute an important class of compounds called electrolytes. Substances that do not yield ions when dissolved are called non-electrolytes. If the physical or chemical process that generates the ions is essentially 100% efficient (all of the dissolved compound yields ions), then the substance is known as a strong electrolyte. If only a relatively small fraction of the dissolved substance undergoes the ion-producing process, it is called a weak electrolyte.

Substances may be identified as strong, weak, or non-electrolytes by measuring the electrical conductance of an aqueous solution containing the substance. To conduct electricity, a substance must contain freely mobile, charged species. Most familiar is the conduction of electricity through metallic wires, in which case the mobile, charged entities are electrons. Solutions may also conduct electricity if they contain dissolved ions, with conductivity increasing as ion concentration increases. Applying a voltage to electrodes immersed in a solution permits assessment of the relative concentration of dissolved ions, either quantitatively, by measuring the electrical current flow, or qualitatively, by observing the brightness of a light bulb included in the circuit.

Demonstration: Electrolytes and electrical conductivity

Set up. The movie below demonstrates the measurement of conductivity using two graphite electrodes. If a solution is conductive, the light bulb will light up. The electrodes are initially tested using a conducting piece of metal, and then first placed in water and then in NaCl(aq).

Prediction. Before watching the video, make a prediction about whether the bulb will light up in water or a solution of NaCl(aq).

Explanation. There is no significant concentration of ions (charge carriers) in water as it consists of mainly neutral H2O molecules. The lamp does not light. However, the presence of freely mobile ions Na+(aq) and Cl–(aq) in NaCl(aq), a strong electrolyte, results in a conducting solution.

Set up. The next movie demonstrates the conductivity of a sucrose solution.

Prediction. Before watching the video, make a prediction about whether the bulb will light up in a sucrose solution.

Explanation. The electrode set up is once again tested to make sure that it is functioning correctly. Sucrose is a non-electrolyte and although the molecules of sucrose separate from each other when the solid dissolves in water, the result is mobile neutral molecules and no ions. The solution does not conduct electricity.

Set up. The next movie demonstrates the conductivity of liquid ethanol.

Prediction. Before watching the video, make a prediction about whether the bulb will light up in ethanol.

Explanation. The electrode set up is once again tested to make sure that it is functioning correctly. Ethanol is a non-electrolyte and the neutral molecules do not conduct electricity.

Set up. The fourth movie in this series demonstrates the conductivity of weak electrolytes.

Prediction. Before watching the video, make a prediction about whether the bulb will light up/how brightly the bulb will light up in the weak electrolyte solutions.

Explanation. This movie shows the conductivity of weak versus strong acids which is relevant to this discussion of electrolytes. This will be expanded on when acids and bases are considered later in this module. The first solution tested is HCl(aq). HCl(g) is a molecule but when dissolved in water clearly produces many ions in solution as evidenced by the brightness of the bulb and is classified as a strong electrolyte. The second solution tested is acetic acid which also produces some ions in solution, although not many as evidenced by the dim glow of the bulb filament. Acetic acid is classified as a weak electrolyte. The third solution is the strong electrolyte NaOH(aq) and the fourth solution is another example of a weak electrolyte, NH3(g) dissolved in water to produce “aqueous ammonia”.

Set up. Another way to produce mobile ions that conduct electricity is to use a molten salt, as shown in the following video. A molten salt is a salt that has been heated to its melting point.

Explanation. The setup is once again tested to make sure that it is functioning properly. When the salt is a solid, the light bulb does not light because the ions are stationary. Once the salt has been heated so that it is now in its liquid state, the mobile ions allow the current to flow and the bulb to light.

Key Concepts and Summary

Chemical equations are symbolic representations of chemical and physical changes. Formulas for the substances undergoing the change (reactants) and substances generated by the change (products) are separated by an arrow and preceded by integer coefficients indicating their relative numbers. Balanced equations are those whose coefficients result in equal numbers of atoms for each element in the reactants and products.

Electrolytes (such as salts) conduct electricity when dissolved in water; non-electrolytes (such as sugar and ethanol) do not conduct electricity when dissolved in water.

Glossary

balanced equation
chemical equation with equal numbers of atoms for each element in the reactant and product
chemical equation
symbolic representation of a chemical reaction
coefficient
number placed in front of symbols or formulas in a chemical equation to indicate their relative amount
product
substance formed by a chemical or physical change; shown on the right side of the arrow in a chemical equation
reactant
substance undergoing a chemical or physical change; shown on the left side of the arrow in a chemical equation

Chemistry End of Section Exercises

  1. What does it mean to say an equation is balanced? Why is it important for an equation to be balanced?
  2. Balance the following equations:
    1. PCl5 + H2O  ⟶  POCl3 + HCl
    2. Cu(s) + HNO3(aq)  ⟶  Cu(NO3)2(aq) + H2O(ℓ) + NO2(g)
    3. H2(g) + I2(s)  ⟶  HI(s)
    4. Fe(s) + O2(g)  ⟶  Fe2O3(s)
    5. Na(s) + H2O(ℓ)  ⟶  NaOH(aq) + H2(g)
    6. (NH4)2Cr2O7(s)  ⟶  Cr2O3(s) + N2(g) + H2O(g)
    7. P4(s) + Cl2(g)  ⟶  PCl3(ℓ)
    8. PtCl4(s)  ⟶  Pt(s) + Cl2(g)
  3. Balance the following chemical equation for the complete combustion of liquid propanol (C3H8O) in the presence of oxygen, and determine the value of “x“.  Be sure to use the lowest whole numbers.
    ___C3H8O(ℓ) + ___O2(g)  →  _x_CO2(g) + ___H2O(ℓ)
  4. Write a balanced molecular equation describing each of the following chemical reactions.
    1. Solid calcium carbonate is heated and decomposes to solid calcium oxide and carbon dioxide gas.
    2. Gaseous butane, C4H10, reacts with diatomic oxygen gas to yield gaseous carbon dioxide and water vapor.
    3. Aqueous solutions of magnesium chloride and sodium hydroxide react to produce solid magnesium hydroxide and aqueous sodium chloride.
    4. Solid lithium metal is added to water and produces aqueous lithium hydroxide and hydrogen gas.
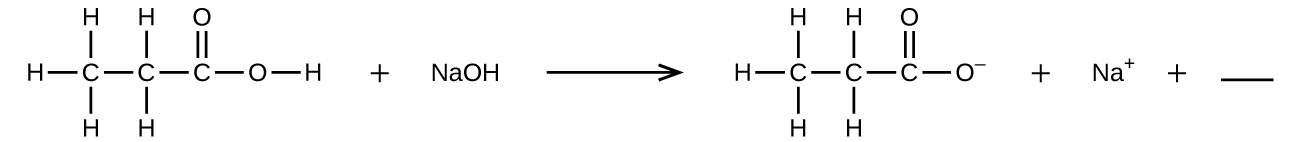
  5. Fill in the blank with a single chemical formula for a covalent compound that will balance the equation:This figure shows a chemical reaction. On the left side of the reaction arrow, a structural formula for a molecule is shown on the far left. It has a C atom on the left to which H atoms are bonded above, below, and to the left. To the right, another C atom is bonded which has H atoms bonded above and below. To the right, another C atom is bonded, which has a double bonded O atom above and another O atom singly bonded to the right. To the right of the singly bonded O atom, an H atom is bonded. This is followed by a plus sign and N a O H. A reaction arrow appears to the right, which is followed by another structural formula. It has a C atom on the left to which H atoms are bonded above, below, and to the left. To the right, another C atom is bonded which has H atoms bonded above and below. To the right, another C atom is bonded, which has a double bonded O atom above and another O atom singly bonded to the right. The singly bonded O atom is followed by a superscript negative sign. This is followed to the right by a plus sign, N a superscript positive sign, another plus sign, and a horizontal line segment, indicating a space for an answer to be written.
  6. Aqueous hydrogen fluoride (hydrofluoric acid) is used to etch glass and to analyze minerals for their silicon content. Hydrogen fluoride will also react with sand (silicon dioxide).  Write an equation for the reaction of solid silicon dioxide with hydrofluoric acid to yield gaseous silicon tetrafluoride and liquid water.
Electrolytes
  1. Consider the solutions presented:
    1. Which of the following sketches best represents the ions in a solution of Fe(NO3)3(aq)?
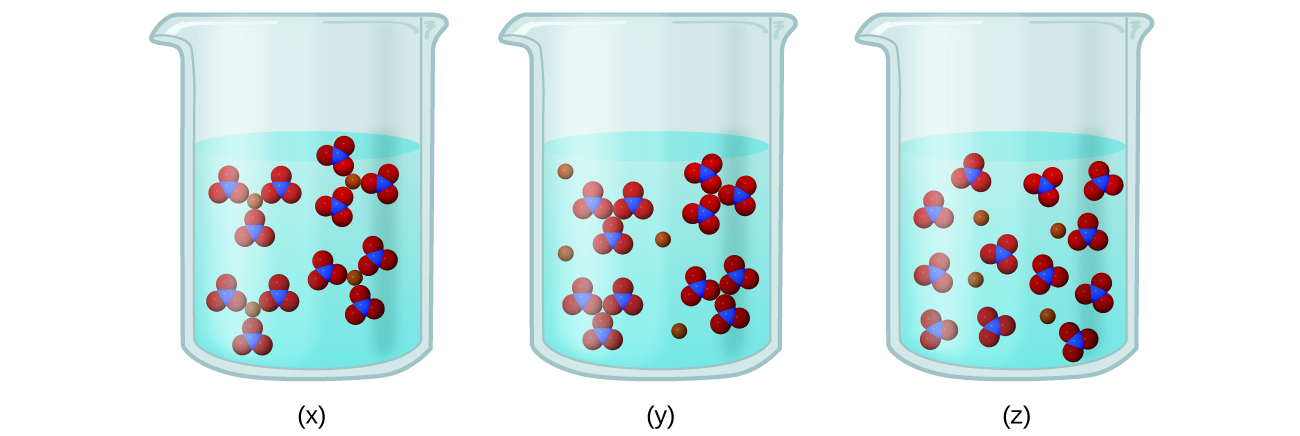
      In this figure, three beakers labeled x, y, and z are shown containing various arrangements of blue and red spheres suspended in solution. In beaker x, three small red spheres surround a single central blue sphere in small clusters which in turn are grouped in threes around a single red sphere, forming four larger clusters. In beaker y, the four large clusters are present without the central red spheres. Four individual red spheres are now present. In beaker z, the large clusters are not present. Twelve of the small clusters of three red and one blue sphere are present along with four single red spheres.
    2. Write a balanced chemical equation showing the products of the dissolution of Fe(NO3)3.
  2. Compare the processes that occur when methanol (CH3OH), lithium chloride (LiCl), and glucose (C6H12O6) dissolve in water. Write equations and prepare sketches showing the form in which each of these compounds is present in its respective solution.
  3. Why are most solid ionic compounds electrically nonconductive, whereas aqueous solutions of ionic compounds are good conductors? Would you expect a liquid (molten) ionic compound to be electrically conductive or nonconductive? Explain.
  4. The military has experimented with lasers that produce very intense light when fluorine combines explosively with hydrogen. What is the balanced equation for this reaction?
  5. Great Lakes Chemical Company produces bromine, Br2, from bromide salts such as NaBr, in Arkansas brine by treating the brine with chlorine gas. Write a balanced equation for the reaction of NaBr with Cl2.

Answers to Chemistry End of Section Exercises

  1. To say you have a balanced equation means you have an equal number of total atoms on the reactant and product side of the reaction. This is important since the law of conservation has to be applied; no matter is lost throughout the reaction
  2. (a) PCl5 + H2O  ⟶  POCl3 + 2 HCl
    (b) Cu(s) + 4 HNO3(aq)  ⟶  Cu(NO3)2(aq) + 2 H2O(ℓ) + 2 NO2(g)
    (c) H2(g) + I2(s)  ⟶  2 HI(s)
    (d) 4 Fe(s) + 3 O2(g)  ⟶  2 Fe2O3(s)
    (e) 2 Na(s) + 2 H2O(ℓ)  ⟶  2 NaOH(aq) + H2(g)
    (f) (NH4)2Cr2O7(s)  ⟶  Cr2O3(s) + N2(g) + 4 H2O(g)
    (g) P4(s) + 6 Cl2(g)  ⟶  4 PCl3(ℓ)
    (h) PtCl4(s) ⟶  Pt(s) + 2 Cl2(g)
  3. x = 6
  4. (a) CaCO3(s)  →  CaO(s) + CO2(g)
    (b) 2 C4H10(g) + 13 O2(g)  →  8 CO2(g) + 10 H2O(g)
    (c) MgCl2(aq) + 2 NaOH(aq)  →  Mg(OH)2(s) + 2 NaCl(aq)
    (d) 2 Li(s) + 2 H2O(ℓ)  →  2 LiOH(aq) + H2(g)

    Left-click here to watch Exercise 4 problem solving video.

  5. H2O
  6. 4 HF(aq) + SiO2(s)  →  SiF4(g) + 2 H2O(ℓ)
  7. (a) Fe(NO3)3 is a strong electrolyte, thus it should completely dissociate into Fe3+ and NO3– ions. Therefore, (z) best represents the solution.
    (b) Fe(NO3)3(s)  →  Fe3+(aq) + 3 NO3–(aq)
  8. CH3OH(l) is completely miscible in water, forming aqueous solution CH3OH(aq). LiCl(s)  →  Li+(aq) + Cl–(aq). When CH3OH(ℓ) and C6H12O6(s) dissolve in water, their molecules become uniformly distributed among the molecules of water with no dissociations.
  9. Most solid ionic compounds have neutral charge, and so there are no electrolytes able to conduct charge. Aqueous solutions of ionic compounds are good conductors because they dissociate to form ions (atoms with charge), which are good electrolytes. One would expect a liquid (molten) ionic compound to conduct electricity because the ions are free to move more in a liquid state than a solid state, and thus, able to allow electricity to flow.
  10. H2(g) + F2(g)  →  2 HF(g)
  11. 2 NaBr(aq) + Cl2(g)  ⟶  2 NaCl(aq) + Br2(ℓ)
Comments
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chem 103/104 Resource Book Copyright © by Chem 103 Textbook Team and Chem 104 Textbook Team is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.