M5Q4: Gas Mixtures and Partial Pressure

Introduction

Gases are rarely found in isolation in nature, but rather in mixtures. This section will explore the independent nature of gases in mixtures through Dalton’s Law of partial pressures. This section includes worked examples, sample problems, and a glossary.

Learning Objectives for Gas Mixtures and Partial Pressures

| Key Concepts and Summary | Key Equations | Glossary | End of Section Exercises |

The Pressure of a Mixture of Gases: Dalton’s Law

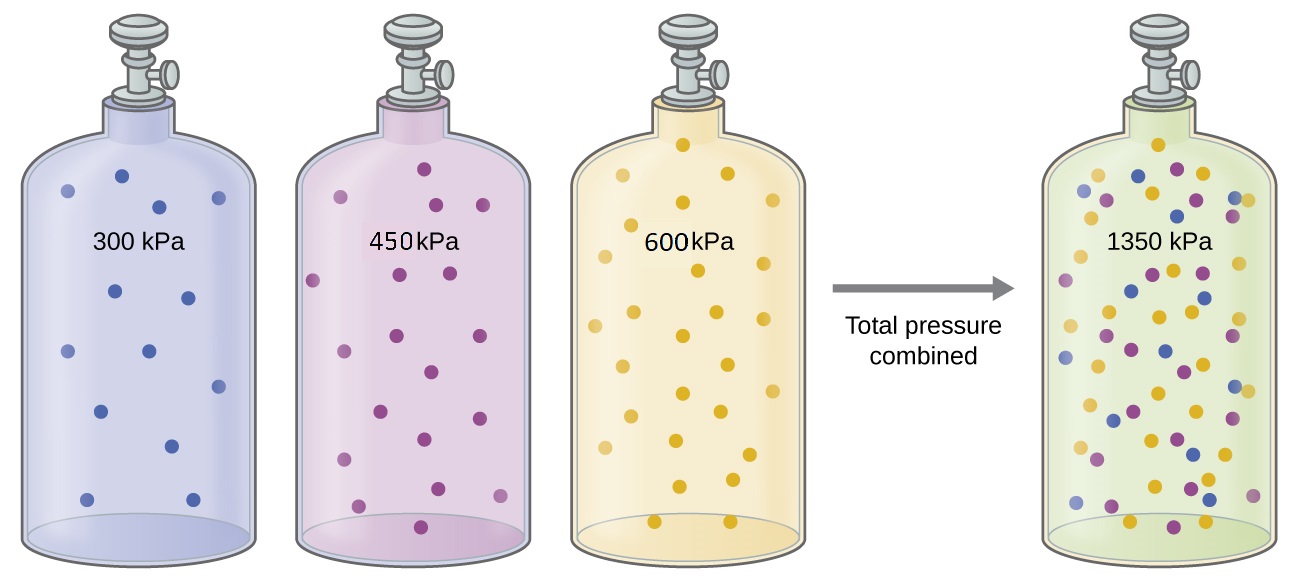
When two or more non reactive gases are added to a container, they will ultimately occupy all of the available container volume as a uniform mixture. The process by which molecules disperse in space in response to differences in concentration is called diffusion, and will be described in more detail in M5Q5. Unless they chemically react with each other, the individual gases in a mixture of gases do not affect each other’s pressure. Each individual gas in a mixture exerts the same pressure that it would exert if it were present alone in the container (Figure 1). The pressure exerted by each individual gas in a mixture is called its partial pressure. This observation is summarized by Dalton’s Law of partial pressures: The total pressure of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases:

PTotal = PA + PB + PC + … = ∑i Pi

In the equation, PTotal is the total pressure of a mixture of gases, PA is the partial pressure of gas A; PB is the partial pressure of gas B; PC is the partial pressure of gas C; and so on.

Figure 1. When the three gases are mixed together, the total pressure of the mixture is equal to the sum of the individual gases’ partial pressures: 300 kPa + 450 kPa + 600 kPa = 1350 kPa. (all four containers are the same size.)

The partial pressure of gas A is related to the total pressure of the gas mixture via its mole fraction (X), a unit of concentration defined as the number of moles of a component of a mixture divided by the total number of moles of all components:

PA = XA × PTotal     with XA = \dfrac{n_{\text{A}}}{n_{\text{Total}}}

where PA, XA, and nA are the partial pressure, mole fraction, and number of moles of gas A, respectively, and nTotal is the number of moles of all components in the mixture.

Example 1

The Pressure of a Mixture of Gases
A 10.0 L vessel contains 2.50 × 10−3 mol of H2, 1.00 × 10−3 mol of He, and 3.00 × 10−4 mol of Ne at 35 °C.

(a) What are the partial pressures of each of the gases?

(b) What is the total pressure in atmospheres?

Solution
The gases behave independently, so the partial pressure of each gas can be determined from the ideal gas equation, using P = \dfrac{nRT}{V}:

PH2 = \dfrac{(2.50 \times 10^{-3} \;\rule[0.5ex]{1.7em}{0.1ex}\hspace{-1.7em}\text{mol})\left(0.08206 \;\frac{\rule[0.4ex]{0.6em}{0.1ex}\hspace{-0.6em}\text{L}\cdot\text{atm}}{\rule[0.3ex]{1.2em}{0.1ex}\hspace{-1.2em}\text{mol}\cdot\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{K}}\right)(308 \;\rule[0.6ex]{0.8em}{0.1ex}\hspace{-0.8em}\text{K})}{10.0 \;\rule[0.7ex]{0.7em}{0.1ex}\hspace{-0.7em}\text{L}} = 6.32 × 10-3 atm

PHe = \dfrac{(1.00 \times 10^{-3} \;\rule[0.5ex]{1.7em}{0.1ex}\hspace{-1.7em}\text{mol})\left(0.08206 \;\frac{\rule[0.4ex]{0.6em}{0.1ex}\hspace{-0.6em}\text{L}\cdot\text{atm}}{\rule[0.3ex]{1.2em}{0.1ex}\hspace{-1.2em}\text{mol}\cdot\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{K}}\right)(308 \;\rule[0.6ex]{0.8em}{0.1ex}\hspace{-0.8em}\text{K})}{10.0 \;\rule[0.7ex]{0.7em}{0.1ex}\hspace{-0.7em}\text{L}} = 2.53 × 10-3 atm

PNe = \dfrac{(3.00 \times 10^{-4} \;\rule[0.5ex]{1.7em}{0.1ex}\hspace{-1.7em}\text{mol})\left(0.08206 \;\frac{\rule[0.4ex]{0.6em}{0.1ex}\hspace{-0.6em}\text{L}\cdot\text{atm}}{\rule[0.3ex]{1.2em}{0.1ex}\hspace{-1.2em}\text{mol}\cdot\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{K}}\right)(308 \;\rule[0.6ex]{0.8em}{0.1ex}\hspace{-0.8em}\text{K})}{10.0 \;\rule[0.7ex]{0.7em}{0.1ex}\hspace{-0.7em}\text{L}} = 7.58 × 10-4 atm

The total pressure is given by the sum of the partial pressures:

PT = PH2 + PHe + PNe = 0.00632 atm + 0.00253 atm + 0.000758 atm = 9.61 × 10-3 atm

Check Your Learning
A 5.73-L flask at 25 °C contains 0.0388 mol of N2, 0.147 mol of CO, and 0.0803 mol of H2. What is the total pressure in the flask in atmospheres?

Answer:

1.14 atm

Here is another example of this concept, but dealing with mole fraction calculations.

Example 2

The Pressure of a Mixture of Gases
A gas mixture used for anesthesia contains 2.83 mol oxygen, O2, and 8.41 mol nitrous oxide, N2O. The total pressure of the mixture is 192 kPa.

(a) What are the mole fractions of O2 and N2O?

(b) What are the partial pressures of O2 and N2O in kPa?

Solution
The mole fraction is given by XA = \dfrac{n_{\text{A}}}{n_{\text{Total}}} and the partial pressure is PA = XA × PTotal

For O2,

XO2 = \dfrac{n_{\text{O}_{2}}}{n_{\text{Total}}} = \dfrac{2.83\;\text{mol}}{(2.83\; +\; 8.41)\;\text{mol}} = 0.252

PO2 = XO2 × PTotal = 0.252 × 192 kPa = 48.4 kPa

For N2O,

XN2O = \dfrac{n_{\text{N}_{2}\text{O}}}{n_{\text{Total}}} = \dfrac{8.41\;\text{mol}}{(2.83\; +\; 8.41)\;\text{mol}} = 0.748

PN2O = XN2O × PTotal = 0.748 × 192 kPa = 143.6 kPa

Check Your Learning
What is the pressure of a mixture of 0.200 g of H2, 1.00 g of N2, and 0.820 g of Ar in a container with a volume of 2.00 L at 20 °C?

Answer:

1.87 atm

Example 3

The pressure of Gases in a Multi-Chamber System

Imagine gases are stored in separate chambers with closed valves. As shown in Figure 2, the two chambers are connected by a tube and two valves. The oxygen gas is contained in the right-hand chamber with an initial volume (Vinitial) of 2.50 L. When both valves are opened, the oxygen gas can occupy both chambers with a total final volume (Vfinal) of 3.75 L.  The initial pressure is given (Pinitial) as 3.0 atm.  When the valves are opened, then the gases mix and come to a new equilibrium pressure.  What is the final pressure?

Figure 2. One chamber is filled with oxygen gas, and it’s connected to a second empty chamber by valves. The oxygen gas is contained in the right-hand chamber with an initial volume of 2.50 L. When the valves are opened, the oxygen gas can occupy both chambers with a total final volume of 3.75 L.

Thinking back to Boyle’s Law (PV = k), one would predict that as the volume increases by opening the valves, the pressure should decrease.  We can calculate the pressure, too.  As the system is closed to the outside, the number of moles of gas (n) remains unchanged.  Even if other gases are present in the new chamber, ideal gases do not interact.

ninitial = nfinal

We assume the temperature (T) does not change.

Tinitial = Tfinal

The gas constant, R (0.08206 L atm mol–1 K–1) does not change .  Thus, we can re-write the ideal gas law (PV = nRT) as:

nRT = PinitialVinitial       and       nRT = PfinalVfinal

Thus,

PinitialVinitial = PfinalVfinal

Solution:

The initial volume is the volume of a single chamber, but the final volume is the volume of the whole system.  Plugging in the values from Figure 2:

(3.0 atm)(2.50 L) = (x)(3.75 L)

Solving for the final pressure, x = 2.0 atm. This lower pressure makes sense since the volume increased.

Check Your Learning
Now imagine that the left bulb contains nitrogen gas.  Determine the final pressure of oxygen, nitrogen, and the total gas pressure once the valves are opened.

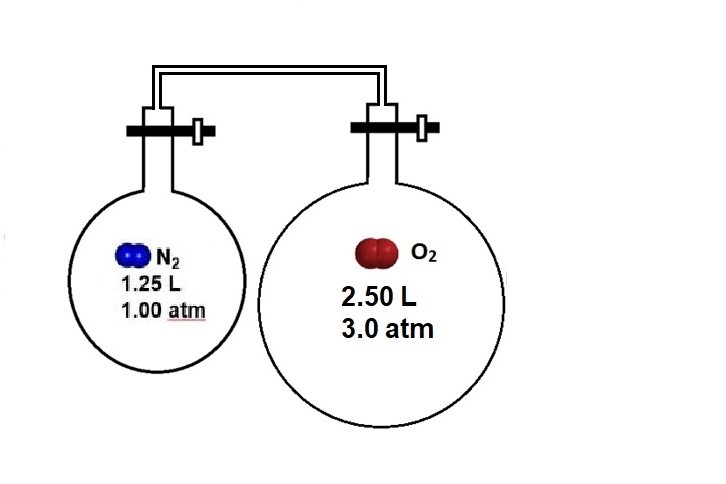
Figure 3. One chamber is filled with oxygen gas, and it’s connected to a second chamber filled with nitrogen gas.

Answer:

The partial pressure of oxygen gas is 2.0 atm; the presence of nitrogen does not affect the partial pressure of oxygen.

The partial pressure of nitrogen gas is 0.33 atm, and the total pressure of the system is 2.3 atm.

Key Concepts and Summary

Dalton’s Law of partial pressure says that the total pressure of a mixture of gases is equal to the sum of the partial pressures of the individual gases. This is because, unless they are chemically reacting with one another, gases do not affect one another. Determining the mole fraction requires knowing the moles of a component gas and the total moles of gas in the mixture. Once the mole fraction is known, it can be used to determine the partial pressure of a component gas.

Mixtures of gases are formed quickly via rapid diffusion of the gaseous molecules. Diffusion of gases will lead to an equilibrium and equal concentrations of gas throughout the mixture.

Key Equations

  • PTotal = PA + PB + PC + … = ∑iPi
  • PA = XAPTotal
  • XA = \dfrac{n_{\text{A}}}{n_{\text{Total}}}
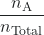
  • PinitialVinitial = PfinalVfinal

Glossary

Dalton’s law of partial pressures
total pressure of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases.
diffusion
movement of an atom or molecule from a region of relatively high concentration to one of relatively low concentration (discussed in this chapter with regard to gaseous species, but applicable to species in any phase)
mole fraction (X)
concentration unit defined as the ratio of the molar amount of a mixture component to the total number of moles of all mixture components
partial pressure
pressure exerted by an individual gas in a mixture

Chemistry End of Section Exercises

  1. A cylinder of a gas mixture used for calibration of blood gas analyzers in medical laboratories contains 5.0% CO2, 12.0% O2, and the remainder N2 at a total pressure of 146 atm. What is the partial pressure of each component of this gas? (The percentages given indicate the percent of the total pressure that is due to each component.)
  2. A sample of gas isolated from unrefined petroleum contains 90.0% CH4, 8.9% C2H6, and 1.1% C3H8 at a total pressure of 307.2 kPa. What is the partial pressure of each component of this gas? (The percentages given indicate the percent of the total pressure that is due to each component.)
  3. A commercial mercury vapor analyzer can detect, in air, concentrations of gaseous Hg atoms (which are poisonous) as low as 2 × 10−6 mg/L of air. At this concentration, what is the partial pressure of gaseous mercury if the atmospheric pressure is 733 torr at 26 °C?
  4. 300 mmHg of NO(g) and 300 mmHg of O2(g) are placed in a 2.0 L glass flask. After complete reaction to form NO2(g), what are the partial pressures of the three gases in the flask? What is the total pressure of gas in the flask? What volume of the flask does the O2(g) occupy? (Assume temperature is the same before and after reaction, and all species in the flask are at the same temperature.)
  5. A sample of a compound of xenon and fluorine was confined in a bulb with a pressure of 18 torr. Hydrogen was added to the bulb until the pressure was 72 torr. Passage of an electric spark through the mixture produced Xe and HF. After the HF was removed by reaction with solid KOH, the final pressure of xenon and unreacted hydrogen in the bulb was 36 torr. What is the empirical formula of the xenon fluoride in the original sample? (Note: Xenon fluorides contain only one xenon atom per molecule.)
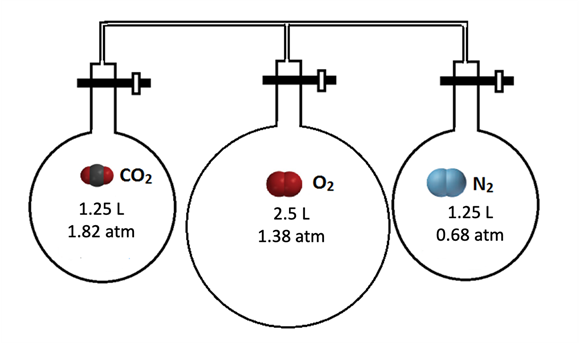
  6. Three bulbs containing different gases at 25 °C are connected by valves, as shown in the diagram below. The diagram shows the volume and pressure in each flask when the valves are closed. What is the partial pressure of O2 gas after all the valves are opened?
    Three bulbs containing different gases at 25 °C are shown. When the valves are closed, bulb 1 contains 1.25 L of CO2 at 1.82 atm, bulb 2 contains 2.5 L of O2 at 1.38 atm, and bulb 3 contains 1.25 L of N2 at 0.68 atm.
  7. In a particular mixture of helium and argon, the partial pressure of argon is higher than the partial pressure of helium.  Select all possibilities below that could explain this observation.
    1. The argon is at a higher temperature, creating more forceful collisions with the container walls.
    2. There are more moles of argon present than moles of helium.
    3. The helium has a larger volume than the argon, leading to a lower pressure.
    4. The helium and argon atoms are moving at the same speed, so the heavier argon atoms create more pressure.
    5. It is not possible for argon to have a higher partial pressure than helium in a mixture.

Answers to Chemistry End of Section Exercises

  1. CO2 = 7.3 atm; O2 = 17.5 atm; N2 = 121.2 atm
  2. CH4: 276 kPa; C2H6: 27 kPa; C3H8: 3.4 kPa
  3. 1.86 × 10-7 torr
  4. PNO = 0 mmHg (limiting reagent), PO2 = 150 mmHg, PNO2 = 300 mmHg; PTotal = 450 mmHg; 2.0 L
  5. XeF4
    Left-click here to watch Exercise 5 problem solving video.

  6. 0.69 atm
  7. B
Comments
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chem 103/104 Resource Book Copyright © by Chem 103 Textbook Team and Chem 104 Textbook Team is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.