D12.4 Energy and Isomerism

The energy needed for rotation around a single bond is relatively small because the molecular orbital of a σ covalent bond has cylindrical symmetry along the internuclear axis (Section: Molecular Orbital (MO) Diagram, Section: Formation of a Sigma Bond). This cylindrical symmetry means that regardless of how two σ-bonded atoms are rotated with respect to each other around the internuclear axis, the σ bond remains unbroken between them.

Therefore, most of the energy change during rotation around a single bond is from increased electron-electron repulsions between the substituents on the two bonded atoms when the substituents get closer to each other. For example, consider the rotation around the C-C single bond in 1,2-dichloroethane:

Each of the three maxima in energy (tops of the hills) corresponds to when a C-H or C-Cl bond on one carbon is spatially close to a C-H or C-Cl bond on the other carbon due to the rotation angle. (The highest energy point corresponds to when the two C-Cl bonds are closest.)

Notice in the figure above that complete 360° rotation around the C–C bond requires an energy input of 40 kJ/mol. From this energy it is possible to calculate that, at room temperature, it takes about 0.1 μs (1 × 10^-7 seconds) for half of a sample of 1,2-dichloroethane to rotate fully about the C-C bond. Therefore, if you could see all the molecules in a room-temperature sample of 1,2-dichloroethane at a specific instant in time, you would find all three of these structures:

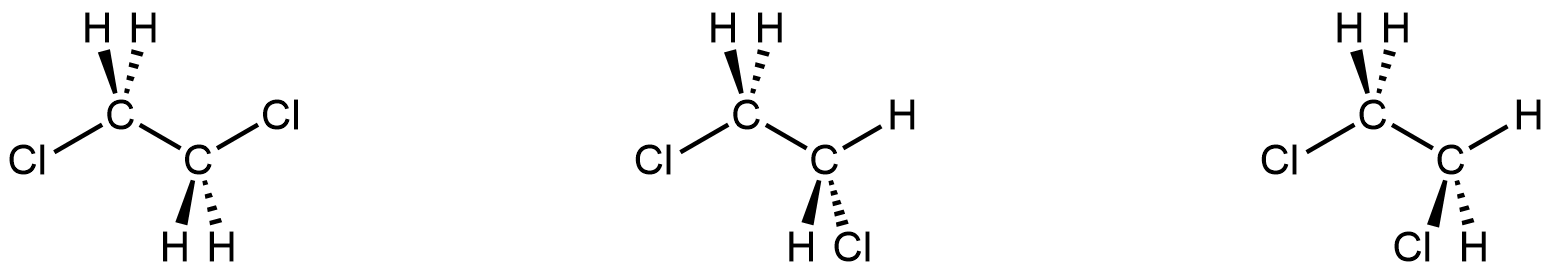
Each structure corresponds to one of the minima (bottom of the valley) in the energy curve in the figure above. If, instead, you followed one molecule over time, you would see it go from one structure to the next in microseconds as the C-C bond rotates (as seen in the animation in the figure). If one structure were separated from the others at room temperature, after a few microseconds the separated structure would change into the other two. Hence they are all conformers of the same molecule. You can draw any of them as a representation of 1,2-dichlororethane.

In contrast to bond rotation, the average bond enthalpy of a C–C single bond is 346 kJ/mol. If we imagine a 1,2-dichloroethane molecule simply falling apart into two equal pieces, breaking at the C-C bond, it would need an energy input of 346 kJ/mol. By a similar calculation, at room temperature, it would take 1 × 10³⁸ years—much longer than the lifetime of our universe—for half of a sample of 1,2-dichloroethane to have its C-C bond broken.

For the simpler ethane molecule (H₃C-CH₃), the energy needed for C-C bond rotation is lower, only 12.1 kJ/mol. Hence, ethane’s C-C bond rotation happens faster, needing only 1 ps (1 × 10^-12 seconds) for half of a sample of ethane to change conformation. (These reaction time scale considerations will be delved into in detail later on in our course.) While the exact energy requirement for rotation about a single bond varies depending on the groups bonded to the two atoms, the timescale for rotation about a single bond is generally short enough that conformers cannot be separated from each other.