"

D20.5 Temperature Dependence of Gibbs Free Energy

Whether a reaction is product-favored, that is, whether the reactants are converted to products under standard-state conditions, is reflected in the arithmetic sign of its ΔrG°. This equation

ΔrG° = ΔrH° − TΔrS°

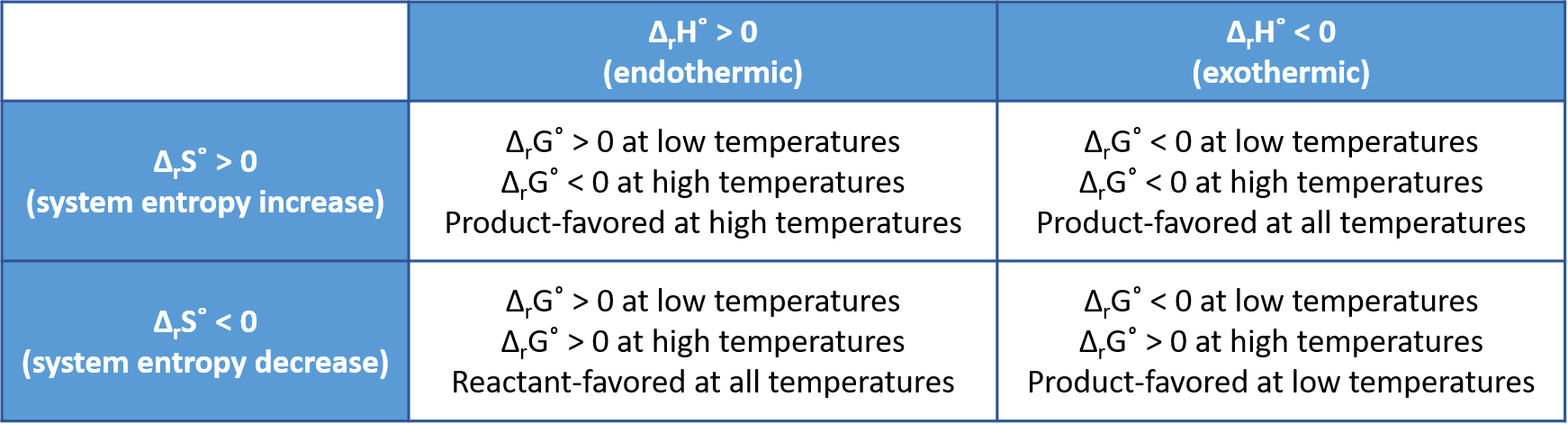
shows that the sign of ΔrG° depends on the signs of ΔrH° and ΔrS°, and, in some cases, the absolute temperature (which can only have positive values). Four possibilities exist:

  1. Both ΔrH° and ΔrS° are positive—an endothermic process with an increase in system entropy. ΔrG° is negative if TΔrS° > ΔrH°, and positive if TΔrS° < ΔrH°. Such a process is product-favored at high temperatures and reactant-favored at low temperatures.
  2. Both ΔrH° and ΔrS° are negative—an exothermic process with a decrease in system entropy. ΔrG° is negative if |TΔrS°| < |ΔrH°| and positive if |TΔrS°| > |ΔrH°|. Such a process is product-favored at low temperatures and reactant-favored at high temperatures. (Remember that |TΔrS°| represents the magnitude of TΔrS°, ignoring mathematical sign.)
  3. ΔrH° is positive and ΔrS° is negative—an endothermic process with a decrease in system entropy. ΔrG° is positive regardless of the temperature. Such a process is reactant-favored at all temperatures.
  4. ΔrH° is negative and ΔrS° is positive—an exothermic process with an increase in system entropy. ΔrG° is negative regardless of the temperature. Such a process is product-favored at all temperatures.

These four scenarios are summarized in this figure:

A table with three columns and four rows is shown. The first column has the phrase, “Delta sub r capital S standard greater than zero ( entropy increase ),” in the third row and the phrase, “Delta sub r capital S standard less than zero ( entropy decrease ),” in the fourth row. The second and third columns have the phrase, “Summary of the Four possibilities for Standard Reaction Enthalpy and Entropy Changes,” written above them. The second column has, “Delta sub r capital H standard greater than zero ( endothermic ),” in the second row, “Delta sub r capital G less than zero at high temperature, delta G greater than zero at low temperature, Process is product-favored at high temperature,” in the third row, and “Delta sub r capital G standard greater than zero at low temperature, Delta sub r capital G standard greater than zero at high temperature, Process is reactant-favored at all temperatures,” in the fourth row. The third column has, “delta sub r capital H standard less than zero ( exothermic ),” in the second row, “delta sub r capital G standard less than zero at low temperature, delta sub r capital G standard less than zero at high temperature, Process is product-favored at all temperatures,” in the third row, and “delta sub r capital G standard less than zero at low temperature, delta sub r capital G standard greater than zero at high temperature, Process is product-favored at low temperature.
Figure: Four combinations of signs of ΔrH° and ΔrS°. There are four possible combinations of signs of ΔrH° and ΔrS°. For two combinations, whether a process is reactant-favored or product-favored under standard-state conditions depends on temperature.

Activity: Temperature and Product-favored or Reactant-favored Reactions

The next figure illustrates the four scenarios graphically, where ΔrG° is plotted versus temperature:

ΔrG° = − ΔrS°(T) + ΔrH°
y = m(x) + b
Figure: Graphic representation of variation in ΔrG° with temperature. These plots show the variation in ΔrG° with temperature for the four possible combinations of arithmetic signs of ΔrH° and ΔrS°. Click on each “+” for more information.

For most reactions, neither ΔrH° nor ΔrS° change significantly as temperature changes. Thus, in the preceding figure, the plots representing ΔrG° are linear because the slope of each plot (−ΔrS°) is the same at all temperatures. The orange and green plots (representing examples of scenario 1 and 2, respectively) cross from product-favored to reactant-favored (cross ΔrG° = 0) at a temperature that is characteristic to each specific process. This temperature is represented by the x-intercept, the value of T for which ΔrG° is zero:

ΔrG° = 0 = ΔrH° − TΔrS°
T_{{\Delta} _{\text{r}}G^{\circ}=0} = \dfrac{{\Delta} _{\text{r}}H^{\circ}}{{\Delta}_{\text{r}}S^{\circ}}

Hence, saying a process is product-favored at “high” or “low” temperatures is simply indicating whether the temperature is above or below TΔrG°=0 for that process. These relative terms are reaction-specific, that is, what is a “high” temperature for one reaction may very well be a “low” temperature for another reaction.

Exercise: Estimating Boiling Point

Comments
Please use this form to report any inconsistencies, errors, or other things you would like to change about this page. We appreciate your comments. 🙂

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chemistry 109 Fall 2021 Copyright © by John Moore; Jia Zhou; and Etienne Garand is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book